5 Quick Steps to Solving One-Step Linear Equations
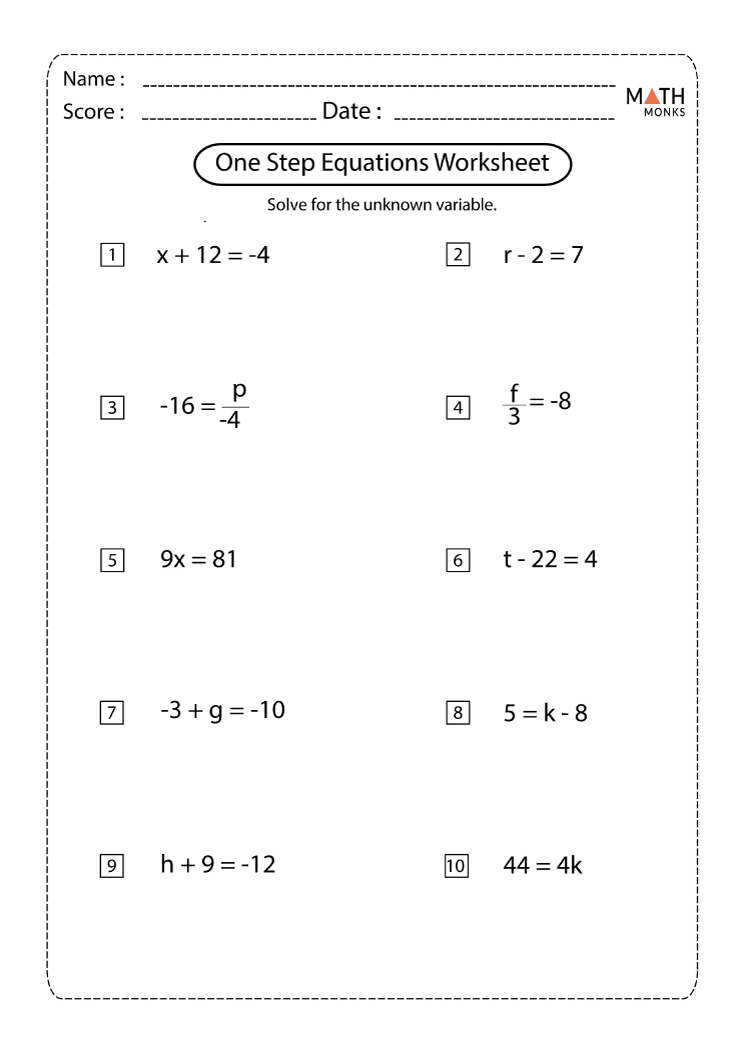
Introduction to One-Step Linear Equations

One-step linear equations are the simplest form of algebraic equations that you’ll encounter in mathematics. These equations involve performing just one operation to isolate the variable on one side of the equation. Whether you’re tackling homework assignments, studying for exams, or simply refreshing your math skills, understanding how to solve these equations efficiently can greatly simplify your math journey.
Step 1: Identifying the Equation Type


First, identify the type of operation you need to perform. One-step linear equations typically involve:
- Addition or Subtraction
- Multiplication or Division
Here’s an example to illustrate:
| Equation | Operation |
|---|---|
| x + 3 = 10 | Subtraction |
| 5x = 25 | Division |

💡 Note: By identifying the operation, you can quickly determine how to solve the equation without confusion.
Step 2: Perform the Operation to Isolate the Variable

Once you’ve identified the operation, execute it on both sides of the equation to isolate the variable:
- If the equation is x + 3 = 10, subtract 3 from both sides:
- Equation: x + 3 - 3 = 10 - 3
- Result: x = 7
- If the equation is 5x = 25, divide both sides by 5:
- Equation: (5x) / 5 = 25 / 5
- Result: x = 5
Step 3: Simplify the Equation

After performing the operation, simplify the equation to its simplest form. In the examples above:
- x + 3 = 10 becomes x = 7
- 5x = 25 becomes x = 5
Step 4: Check Your Solution

Always verify your solution by plugging the found value of the variable back into the original equation:
- For x + 3 = 10:
- Original: 7 + 3 = 10
- Check: 10 = 10 (True)
- For 5x = 25:
- Original: 5 * 5 = 25
- Check: 25 = 25 (True)
Step 5: Understanding Your Answer

After solving and checking, take a moment to understand the significance of the solution:
- The value of x gives you a specific quantity or measure.
- It represents a solution that balances both sides of the equation.
By following these simple steps, you can confidently solve one-step linear equations with ease. This method not only helps in solving basic algebra but also lays the groundwork for more complex equations you might encounter later on. Remember, practice is key to mastering these techniques, so don't hesitate to apply what you've learned to various types of equations.
What if the variable is on the right side of the equation?

+
It doesn’t matter where the variable is in a one-step equation. Perform the inverse operation on both sides to isolate the variable, regardless of its position.
Can I perform the operation in reverse?

+
Yes, you can. If you’re adding or subtracting, you can do the opposite to isolate the variable. For multiplication and division, you can also reverse the operation.
Why do we perform operations on both sides?

+
This maintains the equality of the equation. By performing the same operation on both sides, you’re ensuring that whatever you do to one side, you do to the other to keep the balance.