One Step Equations with Integers: Answer Key Revealed
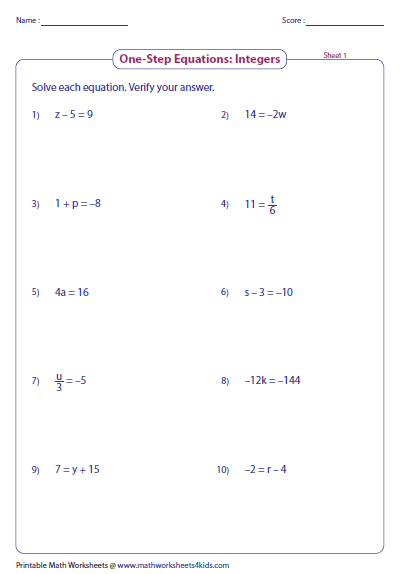
Welcome back to our blog series on mastering algebra! Today, we'll dive into the world of one-step equations with integers, and we'll make sure you leave with a clear, confident understanding of how to solve them. As we progress, we'll unravel the concept in such a way that it not only simplifies these equations for you but also equips you with a vital mathematical tool for future challenges.
Understanding One-Step Equations

Before we jump into solving, let’s solidify what one-step equations are. These are algebraic expressions where you can solve for the unknown variable with just one operation. Here’s a quick recap:
- Addition: x + a = b
- Subtraction: x - a = b
- Multiplication: ax = b
- Division: x ÷ a = b
The Basics of Solving One-Step Equations

The key to solving these equations is isolating the variable. You do this by performing the inverse operation on both sides of the equation to keep it balanced. Here’s a step-by-step approach:
- Identify the operation: Look at the equation to see what operation is locking the variable in place.
- Perform the inverse operation: If the variable is added, subtract; if multiplied, divide; and so on.
- Do this on both sides: Maintain equality by applying the same inverse operation to both sides of the equation.
- Check your work: Ensure your solution fits the original equation.
⚠️ Note: Always verify your solution to avoid common calculation errors.
Solving One-Step Equations with Integers

Now, let’s apply our understanding to specific examples with integers. Each example will walk you through the process:
Addition and Subtraction Equations

Consider the equation x + 5 = -2. Here’s how to solve it:
| Step | Action | Result |
|---|---|---|
| 1 | Subtract 5 from both sides | x = -2 - 5 |
| 2 | Simplify | x = -7 |

✨ Note: The sign change from 5 to -5 occurs because of subtraction.
Another example is x - 3 = 4:
| Step | Action | Result |
|---|---|---|
| 1 | Add 3 to both sides | x = 4 + 3 |
| 2 | Simplify | x = 7 |
Multiplication and Division Equations

Let’s tackle an equation involving multiplication: 2x = -6. Here’s the breakdown:
| Step | Action | Result |
|---|---|---|
| 1 | Divide both sides by 2 | x = -6 ÷ 2 |
| 2 | Simplify | x = -3 |
For division, consider x ÷ 4 = -1:
| Step | Action | Result |
|---|---|---|
| 1 | Multiply both sides by 4 | x = -1 × 4 |
| 2 | Simplify | x = -4 |
☑️ Note: When dealing with negative numbers, you might need to think twice about the signs.
Wrapping Up
Now that we’ve thoroughly explored solving one-step equations with integers, you should have a firm grasp on this fundamental concept of algebra. We’ve gone through the principles, applied them to various equation types, and highlighted key points to remember. By consistently practicing, your problem-solving skills will sharpen, and you’ll find these equations become second nature. As you continue your journey in algebra, remember that each mathematical operation has its inverse; understanding this will keep you in good stead for tackling more complex equations.
Why do I need to perform the same operation on both sides of the equation?

+
Maintaining balance is crucial in algebra. To keep the equation equal, any operation you apply to one side must also be applied to the other. This ensures the value of the variable is correctly isolated.
What are common mistakes when solving one-step equations?

+
Neglecting the sign of numbers, performing the wrong operation, or not applying the operation to both sides of the equation are frequent errors students make.
How can I check my solution for one-step equations?

+
Substitute your solution back into the original equation. If both sides of the equation are equal, your solution is correct.



