Master Ohm's Law: Interactive Worksheet with Answers

Understanding Ohm's Law is essential for anyone delving into the world of electronics, whether you are a student, a hobbyist, or a professional electrical engineer. This law, named after the German physicist Georg Simon Ohm, forms the backbone of electrical circuit analysis. In this comprehensive guide, we'll explore Ohm's Law through an interactive worksheet format, providing you with step-by-step guidance, answers, and insights into its application.
What is Ohm's Law?

Ohm's Law states that the current I flowing through a conductor between two points is directly proportional to the voltage V across the two points, and inversely proportional to the resistance R of the conductor. Mathematically, it's expressed as:
V = IR
Here, V is the voltage (in volts, V), I is the current (in amperes, A), and R is the resistance (in ohms, Ω).
Interactive Ohm's Law Worksheet
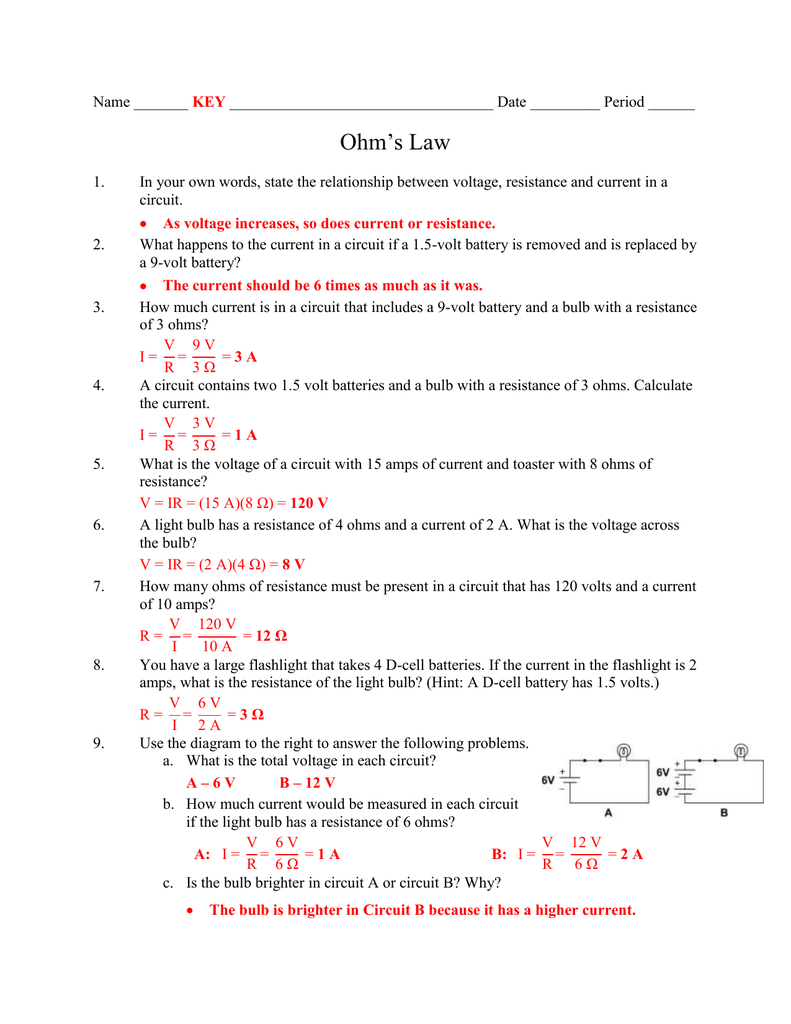
Section 1: Basic Calculations

Let’s start with some basic calculations to familiarize yourself with Ohm’s Law:
- Problem 1: If a light bulb has a resistance of 100 ohms and the voltage applied across it is 220V, calculate the current.
- Solution: Using Ohm's Law, I = V/R = 220V/100Ω = 2.2A. Therefore, the current through the bulb is 2.2 Amperes.
- Problem 2: A circuit component draws a current of 5A with a voltage of 10V across it. What is its resistance?
- Solution: R = V/I = 10V/5A = 2Ω. Thus, the resistance is 2 Ohms.
Section 2: Power and Ohm’s Law

Power (P) in electrical circuits can also be understood through Ohm’s Law. Power is related by the formula:
P = IV or P = I²R or P = V²/R
- Problem 3: If the current in the above example was 5A with a resistance of 2Ω, calculate the power dissipated by the component.
- Solution: Using P = I²R, P = (5A)² * 2Ω = 25 * 2 = 50W. Hence, the power dissipated is 50 Watts.
Section 3: Application in Real-World Scenarios

Understanding Ohm’s Law isn’t just theoretical; it has practical applications in designing and troubleshooting electrical systems:
- Designing Resistor Networks: When engineers design circuits, they often need to know how resistors will behave in series or parallel combinations.
- Troubleshooting: If you know two out of the three variables (V, I, or R), you can solve for the third to diagnose issues in circuits.
Section 4: Advanced Calculations

Here, we’ll delve into more complex scenarios involving Ohm’s Law:
| Component | Resistance (Ω) | Voltage (V) | Current (A) | Power (W) |
|---|---|---|---|---|
| Lamp 1 | 40 | 220 | 5.5 | 1210 |
| Lamp 2 | 60 | 220 | 3.67 | 807.4 |

Given the above table:
- Problem 4: Calculate the combined resistance of Lamp 1 and Lamp 2 when connected in parallel.
- Solution: For parallel resistors, 1/R_eq = 1/R_1 + 1/R_2. Here, 1/R_eq = 1/40 + 1/60 = (3+2)/120 = 1/24, hence R_eq = 24Ω.
- Problem 5: Using the equivalent resistance from Problem 4, find the total current if the voltage applied is 220V.
- Solution: Using Ohm's Law, I = V/R = 220V/24Ω = 9.17A. Thus, the total current is 9.17 Amperes.
Key Insights from Ohm's Law

From the above examples and applications:
- The relationship between voltage, current, and resistance is simple yet fundamental.
- Ohm's Law is universally applicable in DC circuits, with some modifications for AC circuits.
- Power dissipation is crucial to consider to prevent overheating or damage in components.
💡 Note: Always ensure to verify your calculations with real-world measurements, as components might not always behave as ideal resistors due to factors like temperature changes.
End Thoughts

We’ve explored Ohm’s Law through interactive problems, power calculations, and real-world applications. It’s clear that this law is not just a theoretical concept but a tool for practical use in electronics. Understanding and applying Ohm’s Law allows for effective troubleshooting, design, and maintenance of electrical systems. By mastering Ohm’s Law, you equip yourself with the foundational knowledge necessary for deeper exploration into more complex electronic principles.
Why is Ohm’s Law important?

+
Ohm’s Law is fundamental for understanding how electrical circuits function. It helps predict how changing one variable affects the others, essential for designing, troubleshooting, and optimizing electronic systems.
Can Ohm’s Law be applied to all materials?

+
While Ohm’s Law is accurate for ohmic materials, which exhibit a linear relationship between voltage and current, some materials like semiconductors or non-linear devices do not follow this law precisely due to their complex behavior.
What if the voltage in a circuit is unknown?

+
If you know the current and the resistance, you can calculate the voltage using V = IR. This approach is particularly useful for diagnosing issues in circuits or during the design phase where not all parameters are known.