Ohm's Law Worksheet Answers Revealed: Ace Your Exams Easily

In the realm of physics, Ohm's Law is a fundamental principle that every student needs to master. Whether you're preparing for exams, engaging in experimental work, or simply aiming to deepen your understanding of electrical circuits, Ohm's Law is indispensable. Today, we're going to delve into Ohm's Law, explain its various applications, and walk through an Ohm's Law worksheet, ensuring you have all the answers to ace your physics assignments or exams.
Understanding Ohm’s Law

Ohm’s Law, named after Georg Simon Ohm, states that the current passing through a conductor between two points is directly proportional to the voltage across the two points, given that the temperature and other physical conditions remain constant. This relationship can be mathematically expressed as:
- V = I * R
Where:
- V is the potential difference measured in volts (V)
- I is the current in amperes (A)
- R is the resistance in ohms (Ω)
Let's now explore how this law applies in real-world scenarios.
Ohm’s Law Worksheet Walkthrough

To truly master Ohm’s Law, practical application through worksheets is key. Below, we’ll go through a typical Ohm’s Law worksheet, providing answers and explanations for each question:
Question 1: Basic Calculations

Given:
- Voltage (V) = 9V
- Current (I) = 3A
Calculate the resistance.
Answer: R = V / I = 9V / 3A = 3Ω
Question 2: Voltage Determination

Given:
- Current (I) = 0.5A
- Resistance ® = 10Ω
Find the voltage.
Answer: V = I * R = 0.5A * 10Ω = 5V
Question 3: Current Calculation

Given:
- Voltage (V) = 15V
- Resistance ® = 5Ω
Determine the current.
Answer: I = V / R = 15V / 5Ω = 3A
Question 4: Resistor in Series and Parallel

Consider two resistors in series:
- R1 = 2Ω
- R2 = 3Ω
What is the equivalent resistance?
Answer: Equivalent Resistance = R1 + R2 = 2Ω + 3Ω = 5Ω
Now, if these resistors were in parallel?
Answer: 1/Req = 1/2Ω + 1/3Ω. Solving this, we get Req ≈ 1.2Ω
Applications of Ohm’s Law

Understanding Ohm’s Law isn’t just about acing exams; it’s also about its practical applications:
- Electronics Design: Engineers use Ohm’s Law to design circuits, ensuring the right components are used for optimal performance.
- Electrical Troubleshooting: Electricians diagnose problems in electrical systems by understanding current, voltage, and resistance relationships.
- Power Supply: Determining the correct power supply for devices involves applying Ohm’s Law to ensure safety and efficiency.
- Scientific Research: From particle physics to electrical properties of materials, Ohm’s Law plays a critical role in research.
Capstone
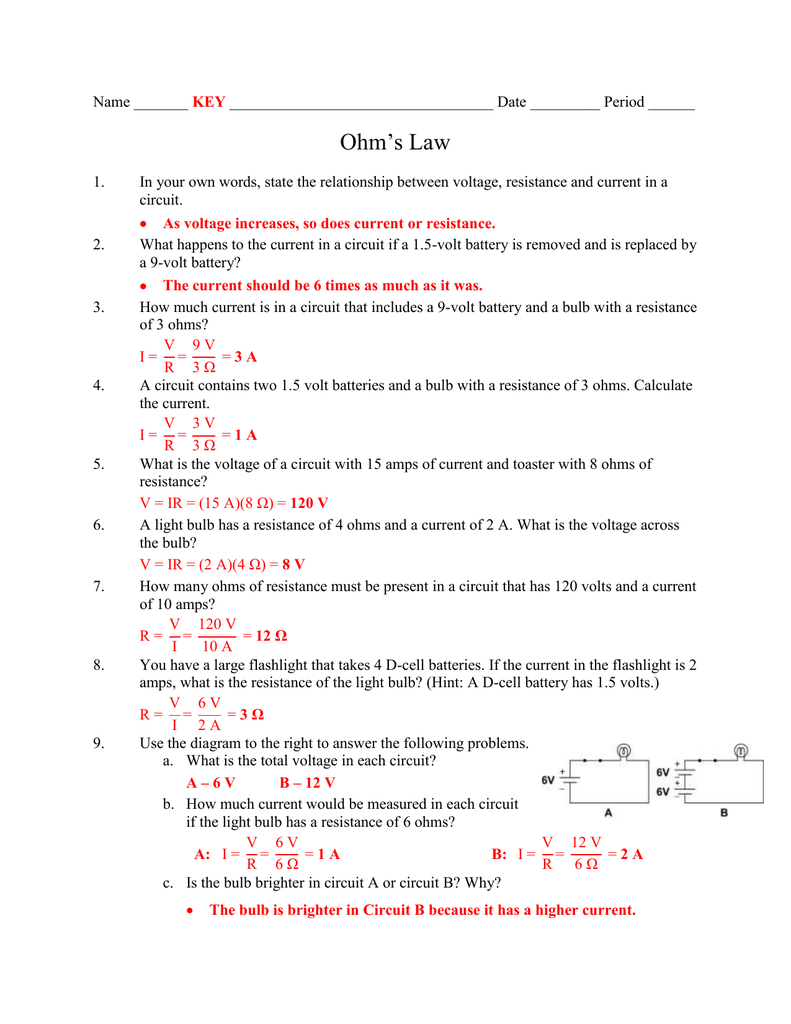
Mastering Ohm’s Law requires not just memorizing the formula but understanding how it applies in various scenarios. We’ve walked through a practical worksheet, explored the concept’s applications, and ensured you have the tools to confidently tackle any related problems. This foundational knowledge in physics will not only help you ace your exams but also provide a strong basis for any future study or career involving electrical principles. By understanding Ohm’s Law, you’re equipped to handle more complex electrical topics, making you a competent and insightful student of physics.
Why is Ohm’s Law important in electronics?

+
Ohm’s Law is crucial in electronics because it allows for the calculation of voltage, current, and resistance, which are essential for designing, analyzing, and troubleshooting electrical circuits. Without understanding these relationships, it would be challenging to create reliable and safe electronic devices.
Can Ohm’s Law be applied to all conductors?

+
Ohm’s Law applies well to ohmic materials (e.g., most metals at constant temperature), but it has limitations for non-ohmic materials like diodes, semiconductors, and materials under extreme conditions where the relationship between voltage, current, and resistance isn’t linear.
What’s the difference between series and parallel circuits in terms of Ohm’s Law?

+
In series circuits, the same current flows through all components, so the total resistance is the sum of individual resistances. In parallel circuits, the voltage across each branch is the same, and the total current is the sum of currents through each branch, with the inverse relationship for resistance.