5 Essential Number Properties for Math Mastery
In the realm of mathematics, understanding number properties is akin to mastering the basic vocabulary of any language. These fundamental concepts provide the groundwork upon which complex arithmetic operations and mathematical problems are built. Whether you're a student, a math enthusiast, or someone aiming to brush up on their mathematical prowess, recognizing and applying these 5 essential number properties will not only enhance your understanding of numbers but also make mathematical calculations much more intuitive. Let's delve into these properties and see how they can transform your approach to mathematics.
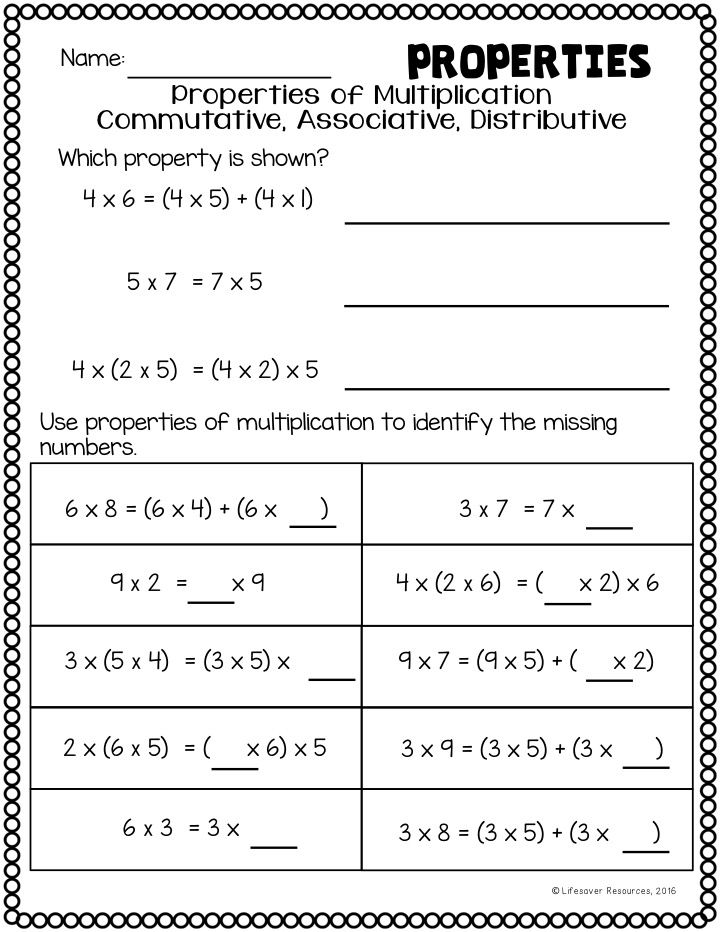
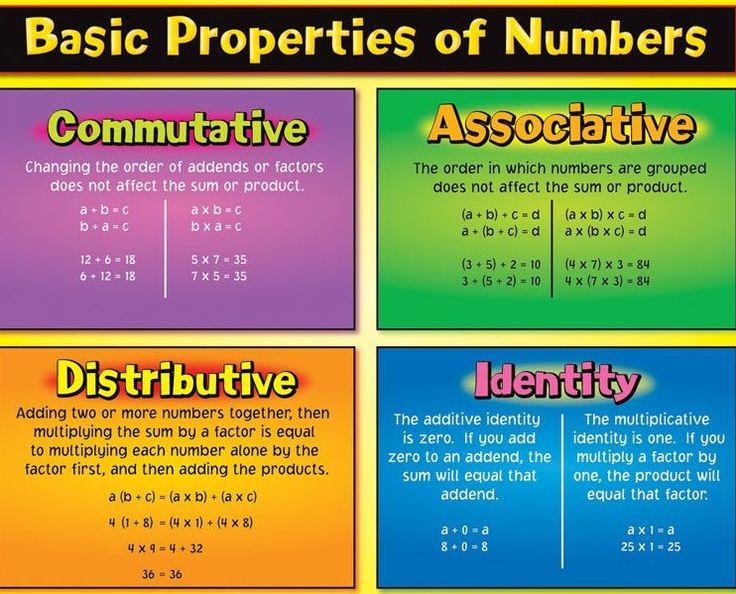
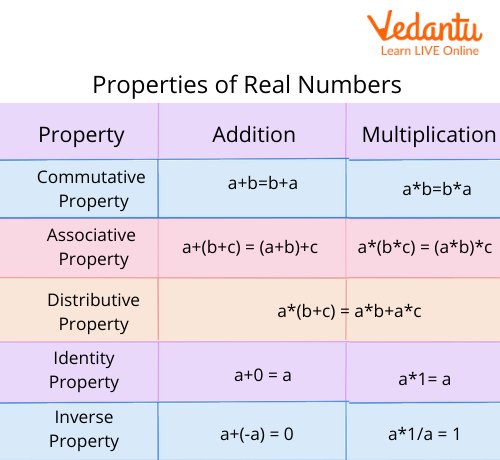
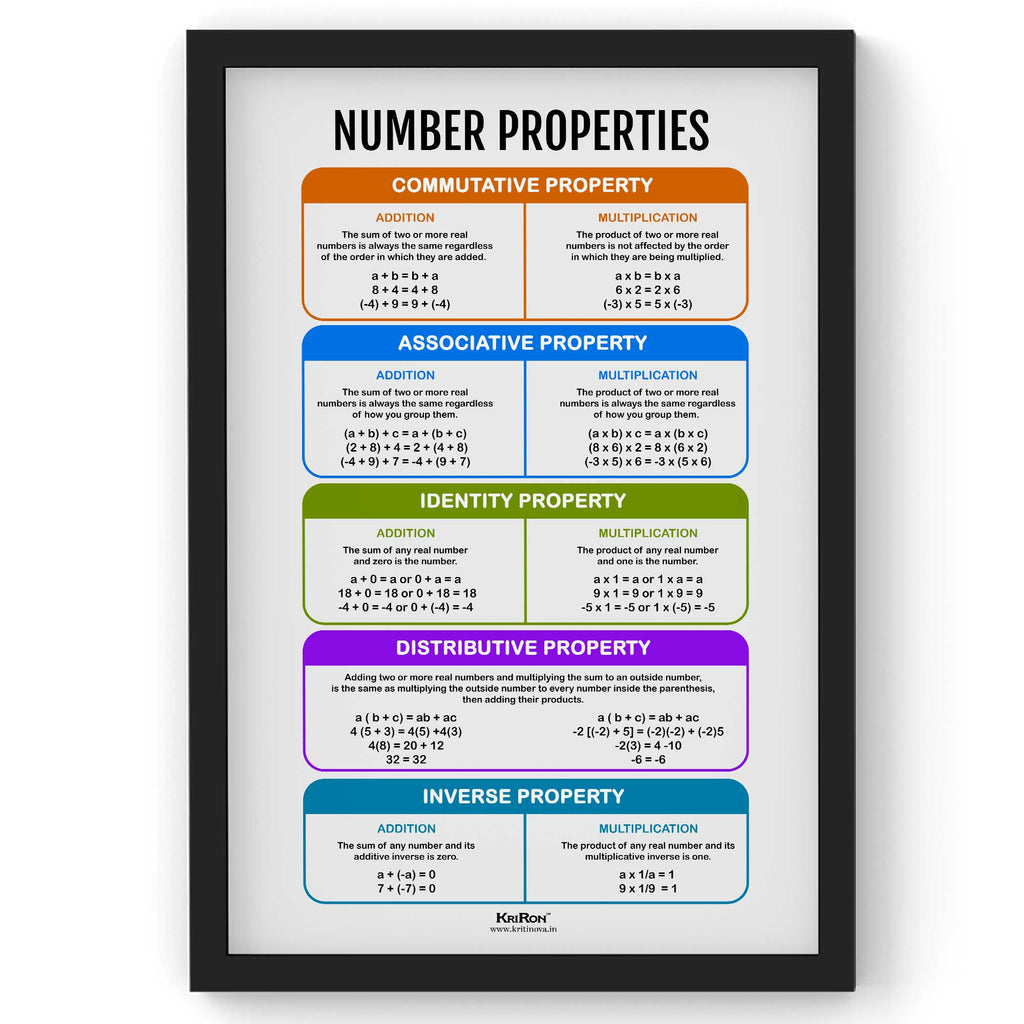
1. Commutative Property

The commutative property is one of the first properties you’ll encounter when exploring mathematics. It states:
- In addition: a + b = b + a
- In multiplication: a × b = b × a
Why is this important? The commutative property tells us that the order of the numbers in an addition or multiplication operation does not affect the result. Here are some practical examples:
- If you’re adding numbers in a column, you can start from the top or the bottom; the sum remains the same.
- Similarly, in multiplication, if you’re calculating 2 × 3, it’s the same as 3 × 2.
2. Associative Property
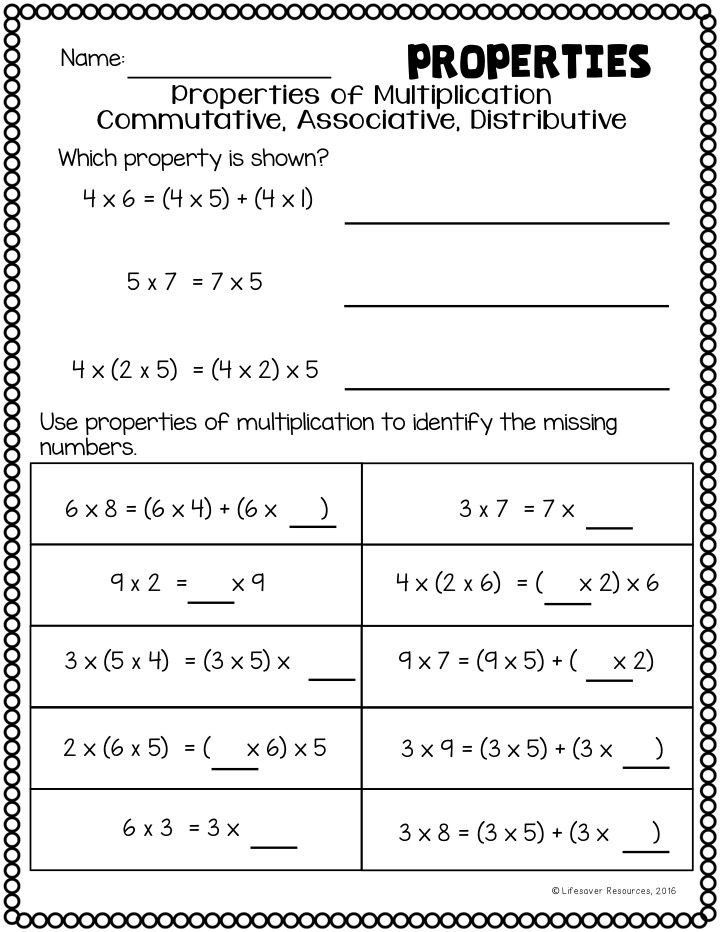
Close behind the commutative property is the associative property, which states:
- In addition: (a + b) + c = a + (b + c)
- In multiplication: (a × b) × c = a × (b × c)
The associative property helps in reorganizing numbers to simplify calculations, particularly useful when dealing with large or fractional numbers:
- For example, when adding (15 + 9) + 1, you can instead perform 15 + (9 + 1), making the calculation easier.
3. Distributive Property
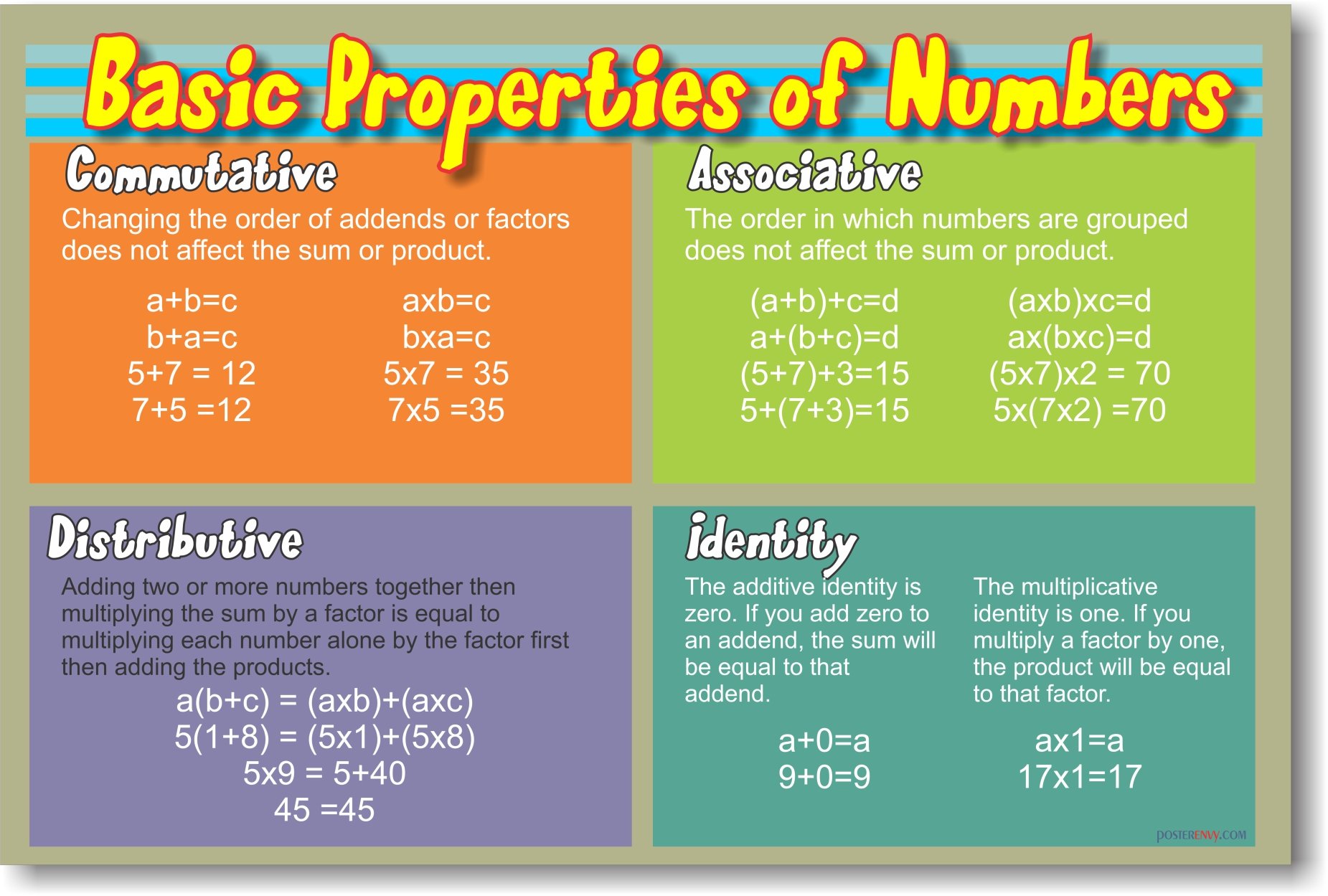
The distributive property links addition and multiplication in a unique way. It’s expressed as:
- a × (b + c) = (a × b) + (a × c)
This property is pivotal for breaking down complex calculations into simpler steps:
- Consider simplifying 3 × (4 + 2). Instead of performing the operation within the parentheses first, you can apply the distributive property to get 3 × 4 + 3 × 2, which equals 12 + 6 = 18.
4. Identity Property
There are two identity properties:
- Additive Identity: a + 0 = a
- Multiplicative Identity: a × 1 = a
The identity properties are essential because they guarantee that there exists a number which does not change the value of another number when operated upon:
- For addition, this number is 0. No matter what number you add to zero, the result is that number.
- For multiplication, this number is 1. Multiplying any number by 1 yields the same number.
5. Inverse Property

The inverse property introduces the concept of an inverse for each operation:
- Additive Inverse: For every number a, there exists a number -a such that a + (-a) = 0
- Multiplicative Inverse: For every non-zero number a, there exists a number 1/a (or a^(-1)) such that a × 1/a = 1
This property is fundamental when dealing with equations, solving for unknowns, or simplifying expressions:
- For example, when solving x + 3 = 5, we subtract 3 (the additive inverse of 3) from both sides to find x = 2.
- In 2x = 4, we divide both sides by 2 (the multiplicative inverse of 2) to find x = 2.
These five number properties form the backbone of arithmetic operations, algebraic manipulations, and many higher-level mathematical concepts. Understanding these properties allows for a more intuitive approach to problem-solving. You'll find that your ability to simplify expressions, solve equations, and understand number relationships will dramatically improve. Moreover, these properties are not just theoretical constructs; they're practical tools that can streamline daily calculations and logical reasoning.
🎯 Note: While these properties primarily relate to integers, they also apply to rational, real, and complex numbers, albeit with some nuances in the case of complex numbers.
⚠️ Note: Remember, while the commutative property applies to addition and multiplication, it does not hold for subtraction or division.
How do these number properties help in everyday calculations?
+
These properties simplify calculations by allowing for the rearrangement of numbers, breaking down complex operations, and providing shortcuts in solving equations.
What’s an example of using the associative property?

+
If you need to add 25, 7, and 13, instead of doing (25 + 7) + 13, you can use the associative property to do 25 + (7 + 13), which might be easier to compute.
Why is the distributive property important?

+
It allows us to simplify complex expressions by distributing the multiplication over addition or subtraction, which can lead to easier computation and problem-solving.
Can the inverse property help in solving equations?
+
Yes, by applying the inverse property, you can isolate the variable or eliminate constants from one side of the equation, aiding in the solution process.
Is there a common mistake involving these properties?

+
One common mistake is assuming all arithmetic operations are commutative, which isn’t true for subtraction and division. For instance, 5 - 3 is not equal to 3 - 5.