5 Simple Steps to Master Net Force Calculations
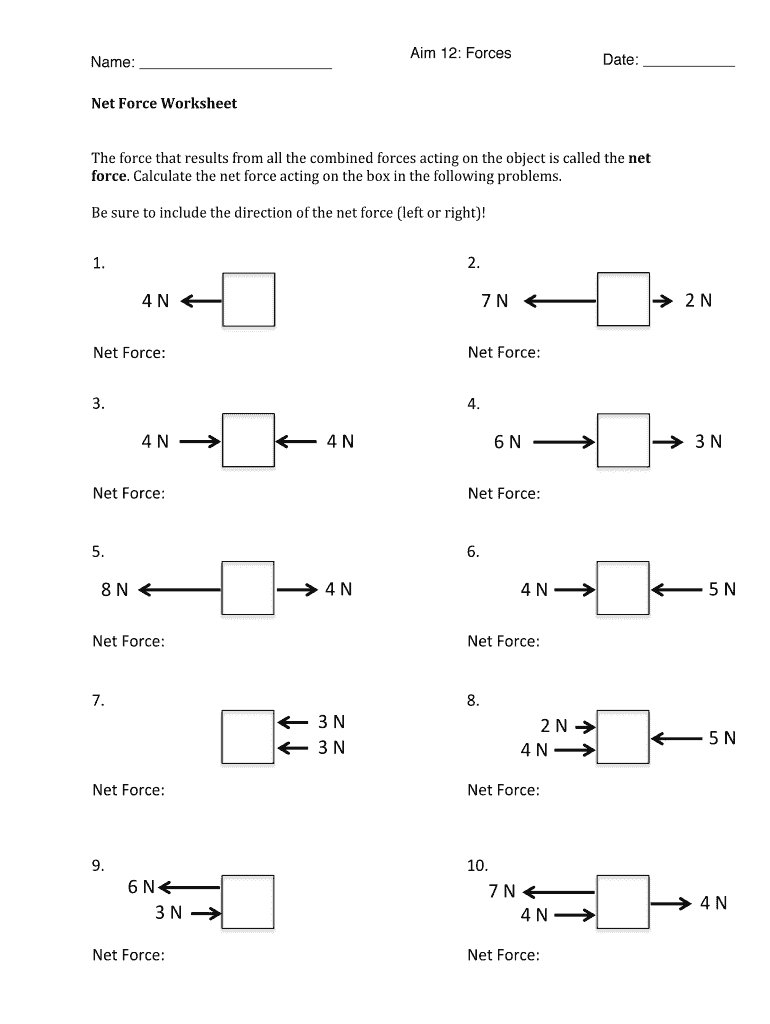
Understanding how to calculate net force in physics is essential for anyone delving into mechanics and the study of motion. Whether you're a student tackling these concepts for the first time or someone revisiting the fundamentals, mastering net force calculations can elevate your understanding of physical phenomena. Here, we'll take you through five simple steps to accurately compute net force, ensuring you can apply these principles effectively in various scenarios.
Step 1: Identify All Forces Acting on the Object

Before you can calculate the net force, you must know all the forces acting upon the object in question. These forces can be:
- Gravity: The force exerted by gravity, which pulls objects downwards.
- Normal Force: The perpendicular reaction force from a surface when an object is placed on it.
- Friction: A force opposing the motion or intended motion between two surfaces.
- Applied Force: Any external force applied by humans or machines.
- Drag: Air resistance or fluid drag acting in the opposite direction of motion.
Once identified, draw a free-body diagram to visually represent these forces, making it easier to analyze their interactions.
🚦 Note: Forces that cancel each other out (like opposing forces in the same direction) can be omitted if their resultant is zero.
Step 2: Determine the Direction and Magnitude of Each Force

Each force has a direction (vector) and a magnitude. Here’s how to deal with them:
- Direction: Assign an arbitrary positive direction. For example, in vertical motion, upwards might be positive, and downwards negative. Horizontally, right could be positive, and left negative.
- Magnitude: Use known formulas or given data to calculate or determine the magnitude of each force. For instance:
- Gravity (F_g): F_g = m * g, where g is approximately 9.8 m/s².
- Friction (F_f): F_f = μ * F_n, where μ is the coefficient of friction and F_n is the normal force.
Remember to account for the angle if forces are not acting along the chosen axis.
⚙️ Note: If an object is at equilibrium, the sum of forces in each direction must equal zero.
Step 3: Sum Up Forces in the Same Direction

With all the forces’ directions and magnitudes known, you can now sum up the forces acting in each direction:
| Force | Direction | Magnitude (N) | Sum (N) |
|---|---|---|---|
| Applied Force | +x | 10 | 30 |
| Gravity (F_g) | -y | 100 | |
| Normal Force (F_n) | +y | 100 | |
| Friction (F_f) | -x | 20 |

Calculate the resultant force for each axis:
- Horizontal (x): F_x = F_applied + (-F_friction) = 10 + (-20) = -10 N (Negative because friction opposes motion)
- Vertical (y): F_y = F_n + (-F_g) = 100 + (-100) = 0 N
Step 4: Calculate the Net Force

Net force is the vector sum of all forces, calculated using Pythagorean theorem in two dimensions:
- If forces act in one direction, the net force is simply the sum or difference of those forces.
- If forces are in two or more dimensions, use: [ F_{\text{net}} = \sqrt{F_x^2 + Fy^2} ] In our example, F{net} = (\sqrt{(-10)^2 + 0^2} = 10) N
Step 5: Apply Newton’s Second Law

The final step integrates the net force with the object’s acceleration:
F{net} = m * a
Where m is the mass of the object and a is its acceleration. Rearranging this formula allows you to find acceleration (a = F{net} / m) or even the mass (m = F{net} / a) if the other two variables are known.
✏️ Note: If the object is not accelerating (at rest or moving at a constant velocity), F{net} equals zero.
In wrapping up, the journey to mastering net force calculations involves identifying forces, understanding their directions and magnitudes, summing up these forces, calculating the net force, and applying it through Newton's laws of motion. Remember, every step builds on the last, forming a comprehensive understanding of how forces interact and affect the motion of objects. Keep practicing, and soon, these calculations will become second nature.
How do I know if a force is positive or negative?

+
Forces in physics are vector quantities, meaning they have both magnitude and direction. You assign a direction as positive (e.g., upwards or to the right), and any force acting in the opposite direction becomes negative in your calculations.
Can net force be negative?

+
Yes, net force can be negative when the direction of the force is opposite to the arbitrarily chosen positive direction for calculation.
What happens if there is no net force?
+
If the net force on an object is zero, the object will either remain at rest or continue moving at a constant velocity according to Newton’s first law of motion.
Why is it important to draw a free-body diagram?

+
Drawing a free-body diagram helps visualize all the forces acting on an object, which is crucial for understanding their interactions, identifying directions, and ensuring accuracy in force calculations.



