Angle Pair Identification: Worksheet Answers Revealed

In the realm of geometry, understanding angle pairs is fundamental for not just solving mathematical problems but also for comprehending the logic behind spatial relationships. This blog post is dedicated to unraveling the mystery of angle pair identification. We'll explore various types of angle pairs, provide a thorough walkthrough on how to identify them, and offer worksheet answers to solidify your understanding.
Angle Pairs: The Basics
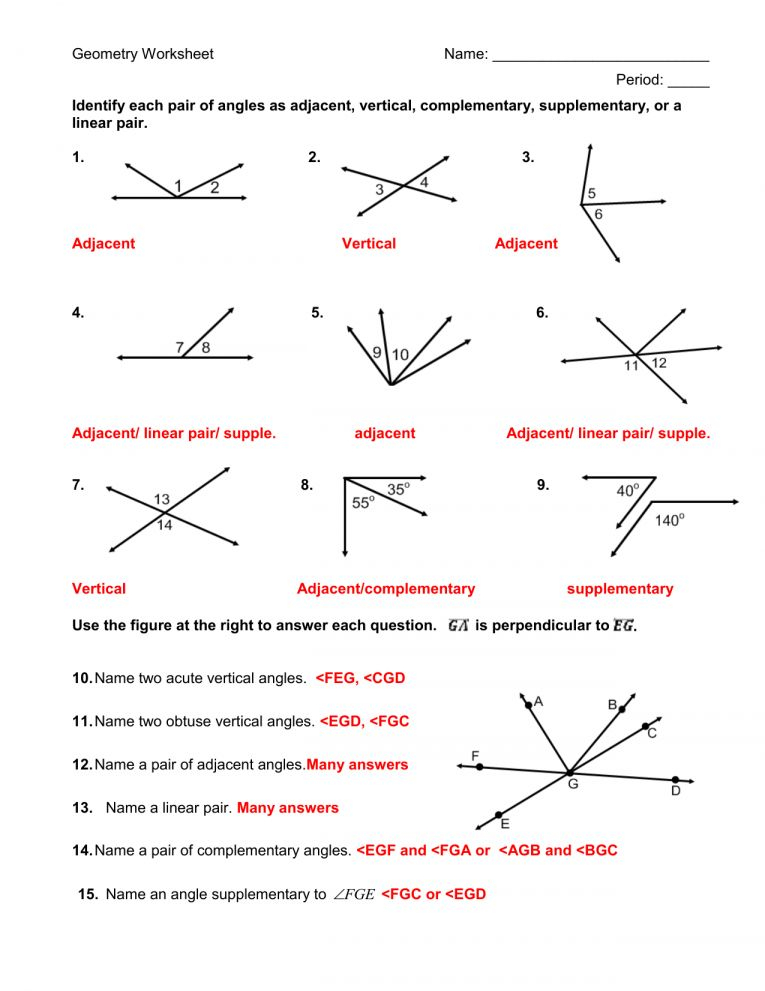
Angles can form various relationships with one another when lines intersect or are placed at certain orientations. Here are the most common types of angle pairs:
- Adjacent Angles - Two angles are adjacent if they share a common vertex, a common side, and do not overlap.
- Vertical Angles - When two lines intersect, the pairs of angles directly across from each other are called vertical angles. They are congruent.
- Linear Pairs - Adjacent angles whose non-common sides are opposite rays (forming a straight line). These sum up to 180 degrees.
- Complementary Angles - Angles whose measures add up to 90 degrees.
- Supplementary Angles - Angles whose measures add up to 180 degrees.
- Alternate Interior Angles - Angles on opposite sides of the transversal but inside the parallel lines.
- Corresponding Angles - Angles in the same relative position at each intersection where a straight line crosses two others.
- Same-Side Interior Angles - Angles on the same side of the transversal but inside the parallel lines.

Identifying Angle Pairs

To effectively identify angle pairs, follow these steps:
- Identify the Lines - Recognize if the lines in question are intersecting, parallel, or if there's a transversal involved.
- Locate the Angles - Examine the position of the angles relative to each other and to any given lines or transversals.
- Determine the Type - Based on the relationship between the angles, decide what kind of angle pair they form. Use the list above as a reference.
💡 Note: Sometimes, more than one type of angle pair can be present at the same time; be sure to note all relevant pairs.
Worksheet Examples
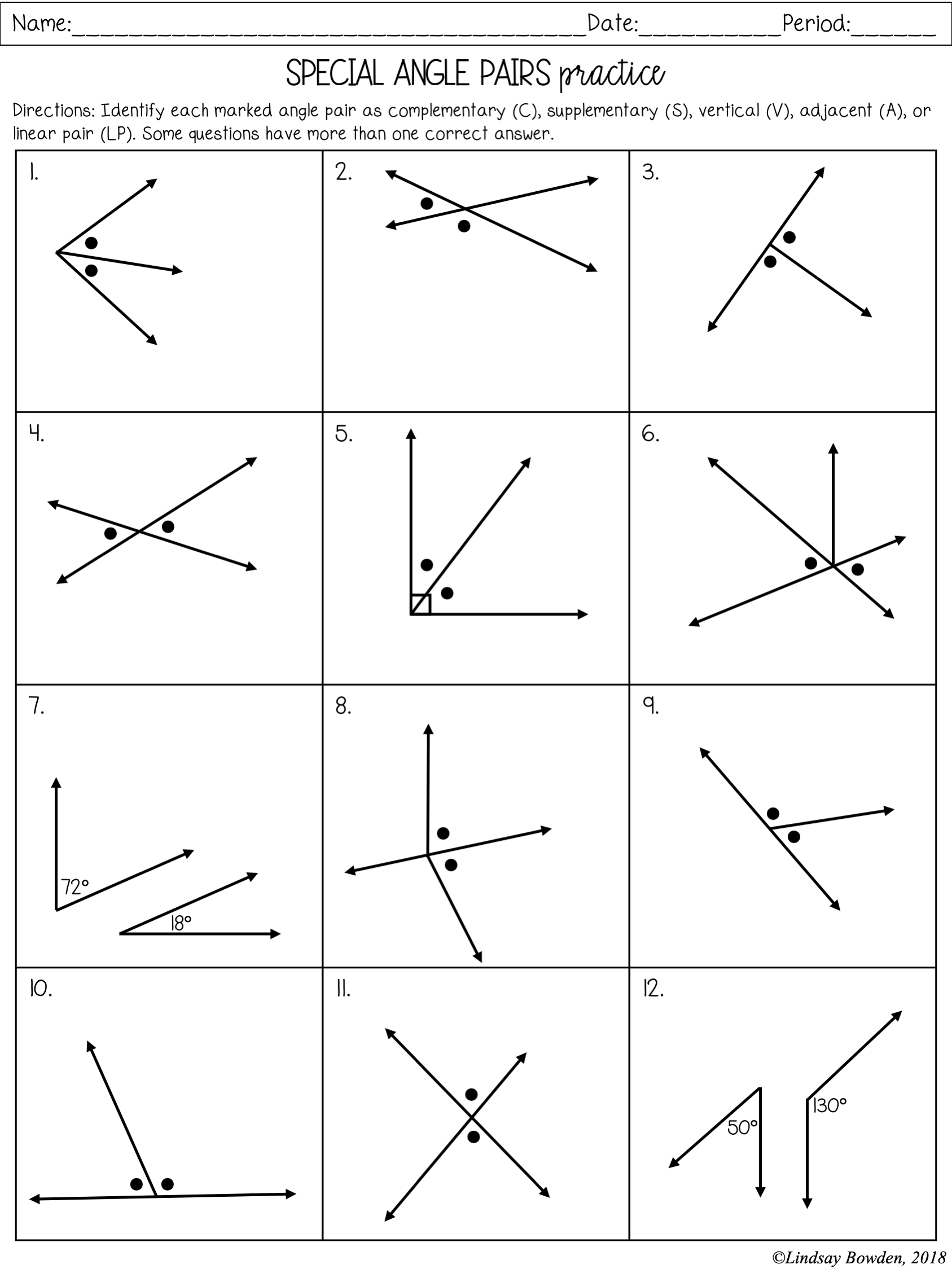
Let’s go through some worksheet examples to apply what we’ve learned:
Worksheet Example 1

Given the following diagram, identify the pairs of angles:

- Adjacent Angles: ∠1 and ∠2, ∠3 and ∠4
- Vertical Angles: ∠1 and ∠4, ∠2 and ∠3
- Linear Pair: ∠1 + ∠2, ∠3 + ∠4
- Alternate Interior Angles: ∠2 and ∠7, ∠3 and ∠6
Worksheet Example 2

Given the following angles, identify if they are supplementary or complementary:
| Angle A | Angle B | Type |
|---|---|---|
| 60° | 120° | Supplementary |
| 45° | 45° | Complementary |

📌 Note: In the above table, the angles add up to 180° for supplementary and 90° for complementary, proving their respective identities.
In conclusion, identifying angle pairs is not just about knowing the definitions but understanding how angles interact within geometric figures. This knowledge aids in solving complex problems, designing structures, and even navigating daily life where spatial relationships are inherent. Hopefully, this detailed walkthrough has demystified angle pairs for you, providing both theoretical understanding and practical application through worksheet examples. Whether you're studying for an exam or just curious about geometry, mastering angle pairs enriches your spatial perception and problem-solving skills.
Why is it important to understand angle pairs?

+
Understanding angle pairs is essential in various fields like architecture, engineering, and art where spatial relationships and angles play a key role. It also simplifies geometric problem-solving and enhances spatial awareness.
Can two angles be both vertical and complementary?

+
Yes, two angles can be both vertical and complementary. For instance, if two intersecting lines form a right angle, the vertical angles would each be 45° which also adds up to 90° making them complementary as well.
How can I use this knowledge in real life?

+
Angle pair identification can be applied in construction for accurately placing beams or in carpentry for cutting wood at precise angles. It’s also used in navigation, when you need to understand angles to determine direction and distance.