Multiplying Rational Expressions Worksheet - Simplifying Algebraic Fractions

Understanding Rational Expressions

Let’s dive into the world of rational expressions, an intriguing part of algebra where we deal with fractions of polynomials. Just like how numbers can be fractions, algebraic expressions can also take the form of fractions. These are known as rational expressions, where the numerator and denominator are polynomials. Understanding how to simplify these expressions is key for advancing in algebraic operations and solving complex problems. In this comprehensive guide, we’ll learn the art of multiplying rational expressions and simplifying them to their most basic forms.
What Are Rational Expressions?

A rational expression consists of polynomials in the numerator and denominator, like so:
[ \frac{P(x)}{Q(x)} ]Where ( P(x) ) and ( Q(x) ) are polynomials and ( Q(x) \neq 0 ). They might include variables like x, y, or z and could be simple like (\frac{3}{x+2}) or complex such as (\frac{x^2 + 2x + 1}{x^3 - 8}).
Why Simplifying is Crucial

The primary reason for simplifying rational expressions is to make them easier to work with. Here are a few reasons:
- Readability: A simplified expression is much easier to read and understand.
- Operations: Simplification makes addition, subtraction, multiplication, and division of expressions more straightforward.
- Solving: It can reveal solutions that might not be immediately apparent in their unsimplified form.
- Accuracy: By eliminating common factors, we reduce the chance for errors in further operations.
Multiplying Rational Expressions
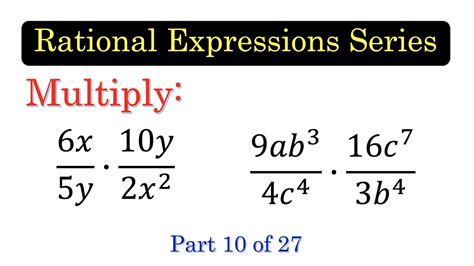
The process of multiplying rational expressions follows a logical step-by-step method. Here’s how you can simplify rational expressions when multiplying them:
Step 1: Factorize All Polynomials

Begin by completely factorizing both the numerator and the denominator of each fraction involved. This means breaking down each polynomial into its irreducible factors.
Step 2: Multiply Numerators Together

After factorizing, multiply the numerators of all the expressions. Keep these factors as you would with regular multiplication of fractions.
Step 3: Multiply Denominators Together

Similarly, multiply the denominators together. Now you have the product of two polynomials in the numerator and the denominator.
Step 4: Simplify by Cancelling Out Common Factors

Here’s where the simplification really happens. Look for any common factors between the numerator and the denominator. Cancel them out to get the simplest form of your expression.
🔍 Note: Remember to only cancel out common factors after factoring, and not the terms in an unfactorized polynomial. For example, x + 1 cannot be canceled with x^2 + 1 .
Example

Let’s work through an example. Suppose you need to multiply these rational expressions:
[ \frac{2x}{x+1} \times \frac{x^2+x-2}{3x} ]- Factorization: \[ \frac{2x}{(x+1)} \times \frac{(x-1)(x+2)}{3x} \]
- Multiplying: \[ \frac{(2x)(x-1)(x+2)}{(x+1)(3x)} \]
- Simplification: \[ \frac{2(x-1)(x+2)}{3(x+1)} \] Here, we have canceled the common factor x in the numerator and denominator.
| Step | Operation | Result |
|---|---|---|
| 1 | Factorize | \frac{2x}{(x+1)} \times \frac{(x-1)(x+2)}{3x} |
| 2 | Multiply | \frac{(2x)(x-1)(x+2)}{(x+1)(3x)} |
| 3 | Simplify | \frac{2(x-1)(x+2)}{3(x+1)} |
Key Points to Remember

- Factor completely: Always factor the polynomials as much as possible. Missing a factor can lead to missing simplification opportunities.
- Cancellation: Cancel only common factors, not just terms that might resemble each other. Be thorough and cautious.
- Order of Operations: The rule of operations applies here. First, do the factorization and multiplication, then simplification.
In conclusion, multiplying and simplifying rational expressions is an essential skill in algebra. It not only makes solving complex equations easier but also helps in understanding the relationships between variables in polynomial forms. As we’ve seen, the process involves meticulous factorization, multiplication, and careful simplification. By mastering this technique, you’ll be well-equipped to tackle more advanced algebraic expressions and problems.
Why do we need to simplify rational expressions?

+
Simplifying rational expressions makes them easier to work with by reducing their complexity, which aids in understanding, solving, and comparing expressions more efficiently.
Can any factor from the numerator be canceled with any factor from the denominator?

+
Only common factors that are identical can be canceled out. The expressions must be the same for simplification to occur.
What if the denominator of the product is zero?

+
If the denominator equals zero at any point, the rational expression is undefined at that value, indicating a discontinuity in the function.