Master Multiplying Positive and Negative Numbers Worksheet
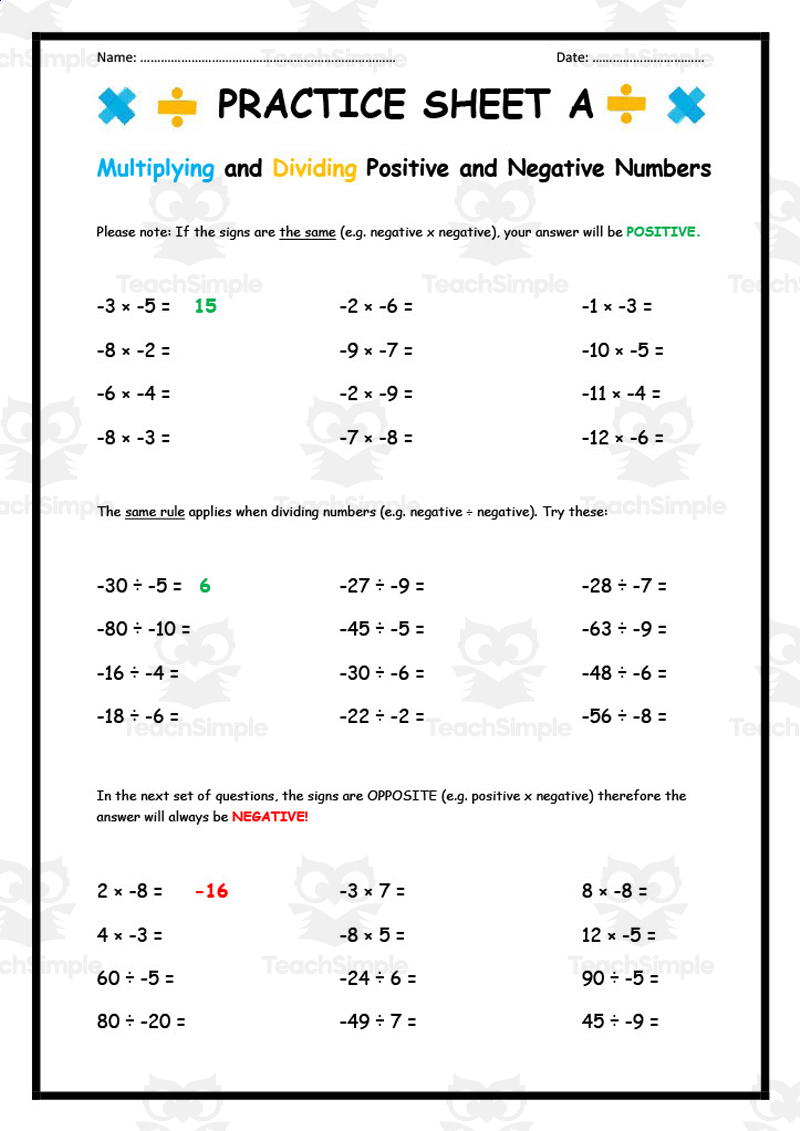
Positive and negative numbers are fundamental to algebra, real numbers, integers, and practically every quantitative field you can think of. Multiplying these numbers involves following specific rules to ensure the results are correct. This guide will delve into the principles, methods, and common pitfalls when multiplying positive and negative numbers. By the end, you'll have a comprehensive understanding of how to navigate these mathematical landscapes, particularly through worksheets designed to reinforce these skills.
Understanding the Rules for Multiplying Positive and Negative Numbers

When multiplying numbers, the rule is straightforward:
- The product of two positive numbers is always positive.
- The product of a positive and a negative number is always negative.
- The product of two negative numbers is always positive.
These rules can be a bit counterintuitive, especially for beginners, because everyday experiences don't usually involve negative quantities in multiplication. Let's explore each rule with examples:
Example: Multiplying Two Positive Numbers

Consider 3 * 4:
- The product of two positive numbers (3 and 4) results in 12, which is positive.
Example: Multiplying a Positive and a Negative Number

Now, let’s look at 3 * (-4):
- When multiplying a positive number (3) by a negative number (-4), the result is -12.
Example: Multiplying Two Negative Numbers

Take -3 * (-4):
- Multiplying two negative numbers yields a positive result: 12.
Understanding these principles is vital as they form the basis for more complex mathematical operations. Here's where worksheets come into play, providing students with exercises to practice and internalize these rules.
Worksheet Design for Multiplying Positive and Negative Numbers

Worksheets for multiplying positive and negative numbers should be structured in a way that:
- Begins with basic examples to warm up the brain.
- Gradually increases in difficulty to challenge the learner.
- Includes word problems for real-world applications.
- Provides immediate feedback through answer keys or self-check methods.
Creating Your Own Worksheet

Here’s a suggested format for designing an effective multiplication worksheet:
| Problem | Example | Answer |
|---|---|---|
| Easy | 3 * 4 = ? | 12 |
| Medium | -3 * 4 = ? | -12 |
| Hard | -6 * (-7) = ? | 42 |
| Word Problem | If you lose $5 for each mistake and you make 3 mistakes, how much money do you lose? | $15 |

💡 Note: When designing worksheets, ensure the problems are varied enough to cover all aspects of the rules. You might want to use random number generators for large sets of problems to ensure each sheet is unique.
Common Mistakes and How to Avoid Them

Mistakes when multiplying positive and negative numbers often stem from:
- Not remembering or applying the rules correctly.
- Confusing subtraction with multiplication when dealing with negatives.
- Overlooking signs, especially in multi-step problems.
To mitigate these:
- Reinforce the rules: Use mnemonic devices like "positive * negative = negative" or rhymes.
- Practice with different formats: Switch between positive and negative numbers in different arrangements to make the rules intuitive.
- Use visual aids: Number lines or counters can help visualize the multiplication process.
Practical Applications

Understanding how to multiply positive and negative numbers isn't just an abstract skill; it has practical applications:
- Finance: Calculating losses and gains or managing debt.
- Physics: Understanding force direction, velocity, and acceleration with vector quantities.
- Statistics: Dealing with z-scores and standard deviations in data sets.
- Computer Science: In programming, handling signed integers and data manipulation.
Wrapping Up

Multiplying positive and negative numbers, while seemingly straightforward, involves an understanding of rules that can trip up even experienced mathematicians if not fully internalized. By using well-designed worksheets, practicing with real-world problems, and being aware of common mistakes, one can become adept at navigating the world of integers with confidence. Remember, the key to mastering these operations lies not only in knowing the rules but in practicing them regularly to make them second nature.
What are the basic rules for multiplying positive and negative numbers?

+
The rules are:
- The product of two positive numbers is positive.
- The product of a positive and a negative number is negative.
- The product of two negative numbers is positive.
How can I remember which sign to use in multiplication?

+
Try to use mnemonic devices like “positive times negative equals negative” or rhymes like “Two negatives make a positive.” Associating these rules with simple catchphrases can help reinforce the concept.
Are there any real-life scenarios where multiplying negative numbers is important?

+
Yes, for instance:
- In finance, when calculating profit or loss over time or across investments.
- Physics, where understanding the direction of forces, velocities, or electrical charges can involve multiplying negative numbers.