Multiplying Polynomials Worksheet: Master Math with Fun

In the vast world of algebra, understanding how to multiply polynomials stands as a fundamental skill, essential for tackling more advanced mathematical concepts. While it might seem daunting at first, multiplying polynomials can be both an engaging and rewarding activity. This post aims to demystify this process, provide practical exercises, and illustrate why this knowledge is invaluable. Whether you're a student, teacher, or a curious mind, let's delve into this topic with enthusiasm and practical understanding.
What Are Polynomials?

Polynomials are expressions composed of variables (often denoted as ( x )) and coefficients, joined by operations like addition, subtraction, and multiplication. They can also involve constants. Here’s a basic definition:
- Monomial: A polynomial with just one term like ( 3x ).
- Binomial: A polynomial with two terms like ( x^2 + 5 ).
- Trinomial: A polynomial with three terms like ( x^2 - 3x + 2 ).
The FOIL Method

When multiplying binomials, the FOIL method stands out as a simple yet powerful technique:
- First: Multiply the first terms.
- Outer: Multiply the outer terms.
- Inner: Multiply the inner terms.
- Last: Multiply the last terms.

Let's apply this method to a simple example: (x+2)(x-3) .
- First: x \cdot x = x^2
- Outer: x \cdot -3 = -3x
- Inner: 2 \cdot x = 2x
- Last: 2 \cdot -3 = -6
Combining these results, we get:
\[ (x+2)(x-3) = x^2 + 2x - 3x - 6 = x^2 - x - 6 \]
Multiplying Larger Polynomials

When dealing with polynomials that are larger than binomials, we extend our multiplication method:
- First, distribute each term of one polynomial to each term of the other.
- Combine like terms after distribution.
Consider multiplying ( (x^2 + x + 1) ) by ( (x - 2) ):
[ (x^2 + x + 1)(x - 2) = x^2(x) + x^2(-2) + x(x) + x(-2) + 1(x) + 1(-2) ]
Distributing and combining like terms gives:
[ = x^3 - 2x^2 + x^2 - 2x + x - 2 ]
[ = x^3 - x^2 - x - 2 ]
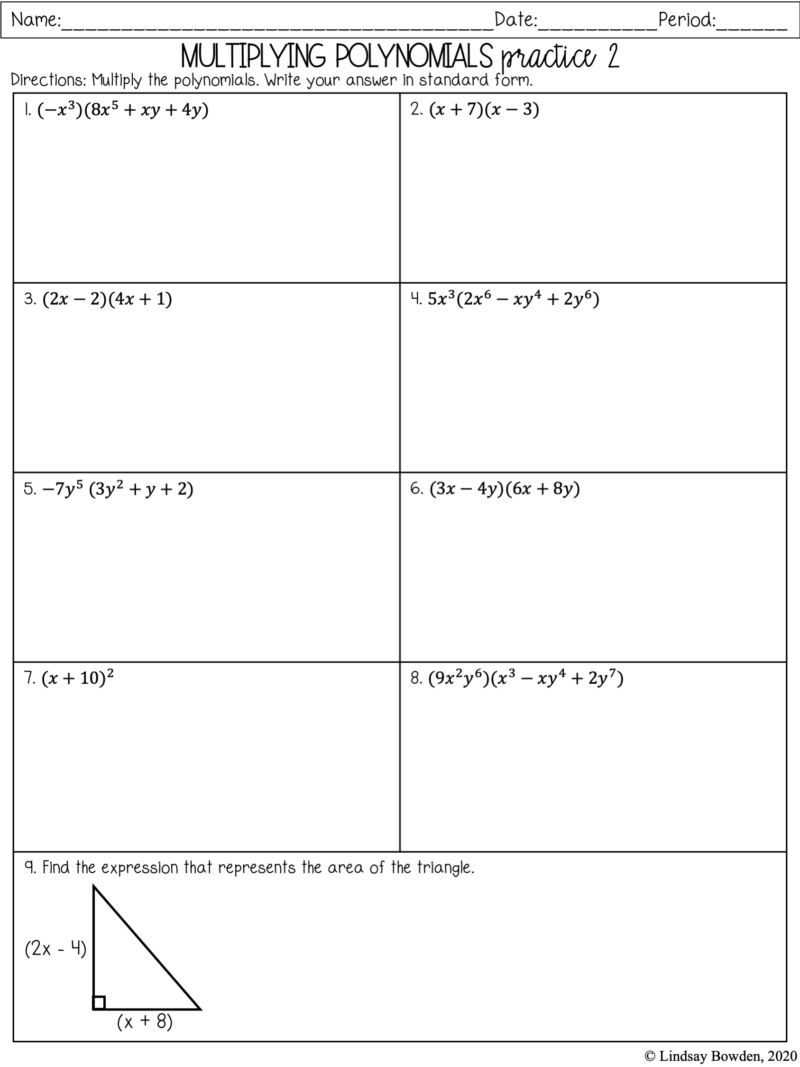
Worksheet for Practice

To enhance your skill, here’s a worksheet you can practice with:
| Problem | Solution |
|---|---|
| ( (x+3)(x-2) ) | ( x^2 + x - 6 ) |
| ( (2x-1)(x+4) ) | ( 2x^2 + 8x - x - 4 = 2x^2 + 7x - 4 ) |
| ( (x^2 + 3x + 2)(x-1) ) | ( x^3 + 3x^2 + 2x - x^2 - 3x - 2 = x^3 + 2x^2 - x - 2 ) |
| ( (x+2)(x^2 - 2x + 1) ) | ( x^3 + x^2 - 2x^2 - 2x + x + 2 = x^3 - x^2 - x + 2 ) |

📝 Note: Always check your work by comparing your results to the solutions. If your answers differ, review your steps carefully.
Understanding how to multiply polynomials opens the door to higher algebra, calculus, and various applications in real life. This skill helps in:
- Developing critical thinking.
- Understanding the behavior of functions.
- Solving complex equations.
As we wrap up this exploration into multiplying polynomials, remember that mastering this skill requires practice. The exercises provided are just the beginning; with continued effort, you'll find polynomial multiplication becoming second nature. Keep at it, challenge yourself with progressively harder problems, and enjoy the journey of mathematical discovery.
Why should I learn how to multiply polynomials?

+
Multiplying polynomials is a fundamental algebraic operation, crucial for understanding more complex mathematical concepts like factoring, polynomial division, and the behavior of functions in calculus.
Can the FOIL method be used for all polynomials?

+
The FOIL method is primarily used for multiplying binomials. For trinomials or higher, you’d extend this method by distributing each term.
Are there any shortcuts for multiplying polynomials?

+
Shortcuts like the box method or grid method for visualizing multiplication can help, but remember that these are just organizational tools to simplify the process. The underlying principle remains the same.
How can I check if my polynomial multiplication is correct?
+
One effective method is to use the distributive property again to check the original expression against your result. Alternatively, computer algebra systems or graphing calculators can provide instant feedback.