Multiplying Fractions by Whole Numbers

Multiplying Fractions by Whole Numbers: A Comprehensive Guide

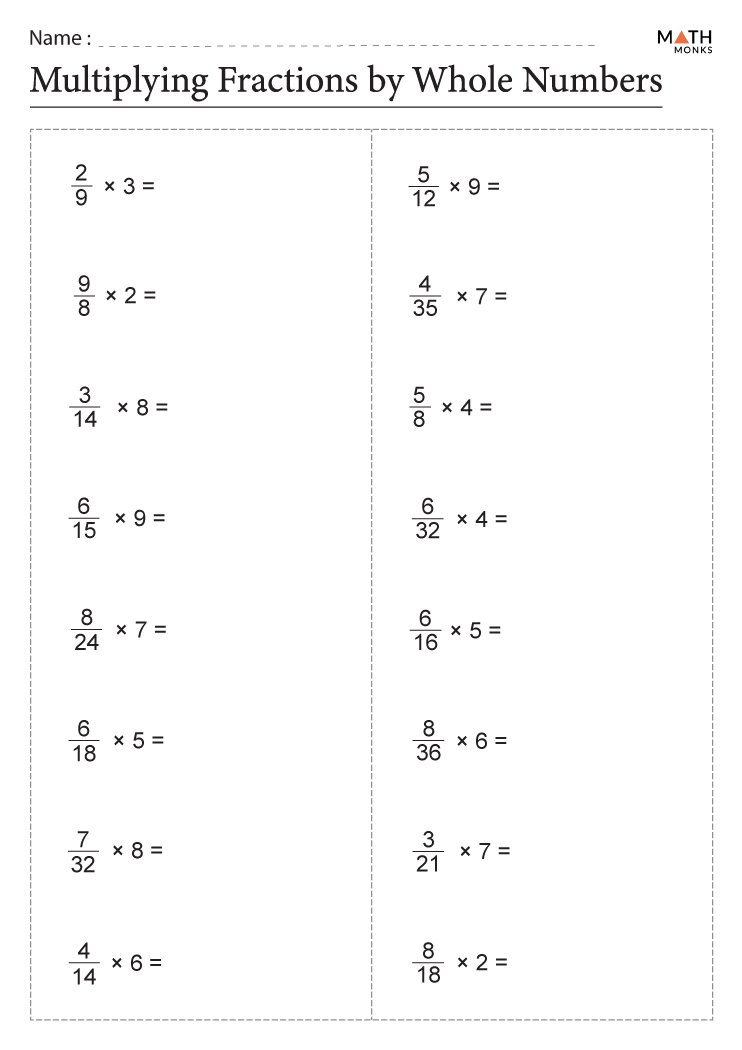
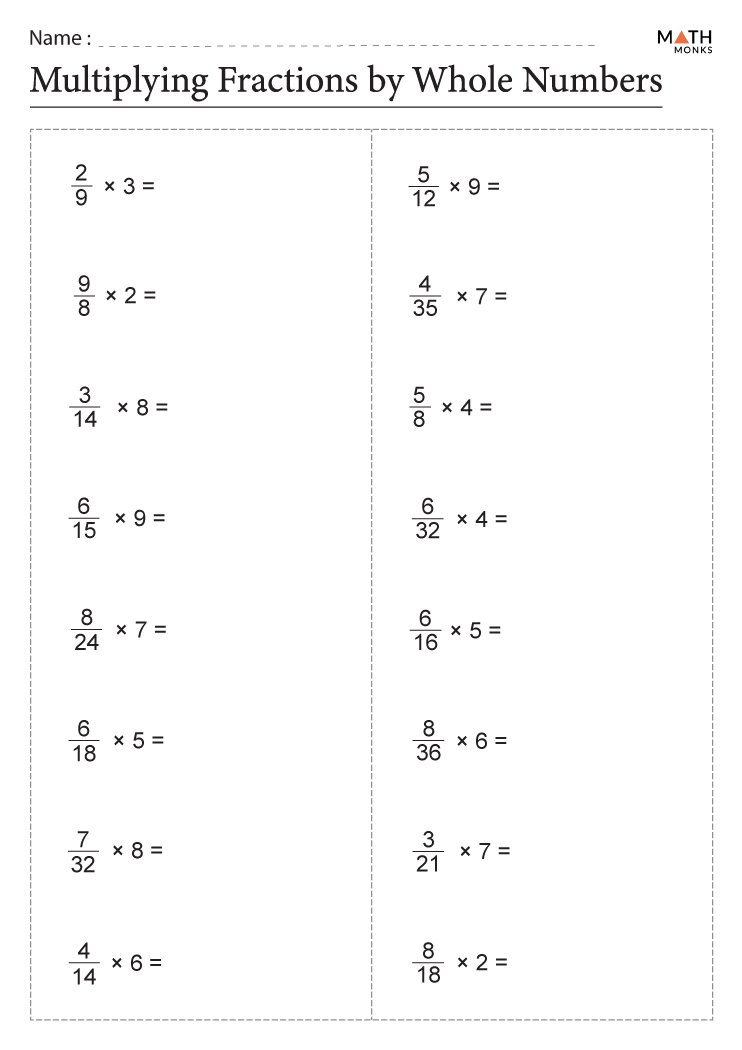
When dealing with fractions and whole numbers, it’s essential to understand how to multiply them correctly. In this article, we’ll delve into the world of multiplying fractions by whole numbers, exploring the concepts, rules, and examples to help you grasp this fundamental math operation.

Understanding Fractions and Whole Numbers

Before we dive into the multiplication process, let’s review the basics of fractions and whole numbers. A fraction represents a part of a whole, consisting of a numerator (the top number) and a denominator (the bottom number). For example, ³⁄₄ represents three equal parts out of a total of four. Whole numbers, on the other hand, are integers without fractions, such as 1, 2, or 3.

Multiplying Fractions by Whole Numbers: The Rule

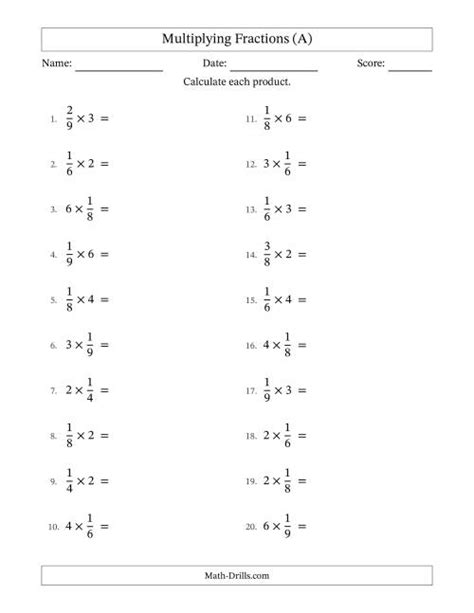
To multiply a fraction by a whole number, you simply multiply the numerator (the top number) by the whole number, while keeping the denominator (the bottom number) the same. The formula for this operation is: (numerator × whole number) / denominator For instance, if you want to multiply ³⁄₄ by 2, you would calculate it as follows: (3 × 2) / 4 = ⁶⁄₄ You can then simplify the resulting fraction, if possible. In this case, ⁶⁄₄ can be simplified to ³⁄₂ by dividing both the numerator and denominator by 2.

Examples and Applications

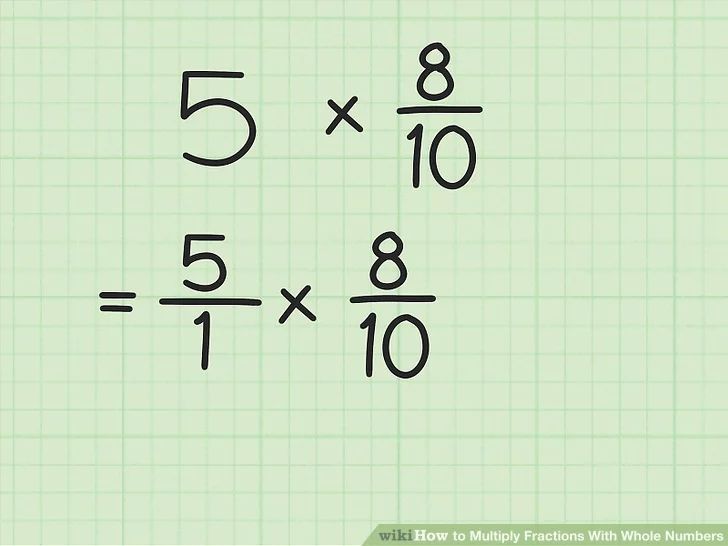
Let’s explore some examples to illustrate the concept: * Multiply ¹⁄₂ by 3: (1 × 3) / 2 = ³⁄₂ * Multiply ²⁄₃ by 4: (2 × 4) / 3 = ⁸⁄₃ * Multiply ³⁄₄ by 5: (3 × 5) / 4 = ¹⁵⁄₄ These examples demonstrate how to apply the rule to different fractions and whole numbers.

Real-World Applications

Multiplying fractions by whole numbers has numerous real-world applications, such as: * Cooking and recipes: When scaling up or down a recipe, you may need to multiply ingredients by a fraction or whole number. * Finance and budgeting: Understanding how to multiply fractions by whole numbers can help you calculate interest rates, investments, or expenses. * Science and engineering: In various scientific and engineering contexts, such as measurement conversions or calculations, multiplying fractions by whole numbers is a common operation.

Common Mistakes to Avoid

When multiplying fractions by whole numbers, be cautious of the following common mistakes: * Forgetting to multiply the numerator: Remember to multiply only the numerator by the whole number, leaving the denominator unchanged. * Not simplifying the result: After multiplying, simplify the resulting fraction, if possible, to express it in its simplest form.

💡 Note: Always double-check your calculations to ensure accuracy and avoid errors.

Conclusion and Final Thoughts

In conclusion, multiplying fractions by whole numbers is a fundamental math operation that requires understanding the rule and applying it correctly. By following the formula and practicing with examples, you’ll become proficient in this essential skill. Remember to avoid common mistakes and simplify your results to ensure accuracy. With this knowledge, you’ll be better equipped to tackle various real-world applications and problems that involve multiplying fractions by whole numbers.