5 Key Rules for Multiplying Exponents Easily

When dealing with the multiplication of exponents, there are a few key rules you can follow to simplify the process. Whether you're solving algebra equations or just refreshing your math skills, understanding these rules will make your mathematical journey a lot smoother. Here's a deep dive into these 5 essential rules for multiplying exponents.
Rule 1: Same Base, Add the Exponents

If you're multiplying exponents with the same base, you simply add the exponents together. For example:
- Expression: x^2 \times x^3
- Simplified: x^{(2+3)} = x^5
This rule holds because multiplication of exponents with the same base effectively counts the total number of times the base is multiplied by itself.
Rule 2: Power to a Power

When an exponent is raised to another exponent, you multiply the exponents. Consider this example:
- Expression: (x^2)^3
- Simplified: x^{(2 \times 3)} = x^6
This rule comes in handy when simplifying expressions or dealing with fractional exponents.
Rule 3: Product to a Power

This rule allows you to distribute a power to each base in a product:
- Expression: (2x)^3
- Simplified: 2^3 \times x^3 = 8x^3
Distributing the exponent to each term in the product ensures that all parts of the expression are raised to the power in question.
Rule 4: Quotient of Powers
If you're dividing exponents with the same base, you subtract the exponents:
- Expression: \frac{x^6}{x^2}
- Simplified: x^{(6-2)} = x^4
Subtracting exponents mirrors the process of canceling out common factors in a division.
Rule 5: Zero and Negative Exponents

Understanding zero and negative exponents can be a bit tricky, but they follow these rules:
- Zero Exponent: Any non-zero number raised to the power of zero is 1.
- Example: 7^0 = 1
- Negative Exponent: Move the base to the denominator if the exponent is negative, or vice versa.
- Example: 2^{-3} = \frac{1}{2^3} = \frac{1}{8}
These rules help us deal with different scenarios in exponent multiplication and division.
When Do These Rules Not Apply?

There are scenarios where these rules do not apply:
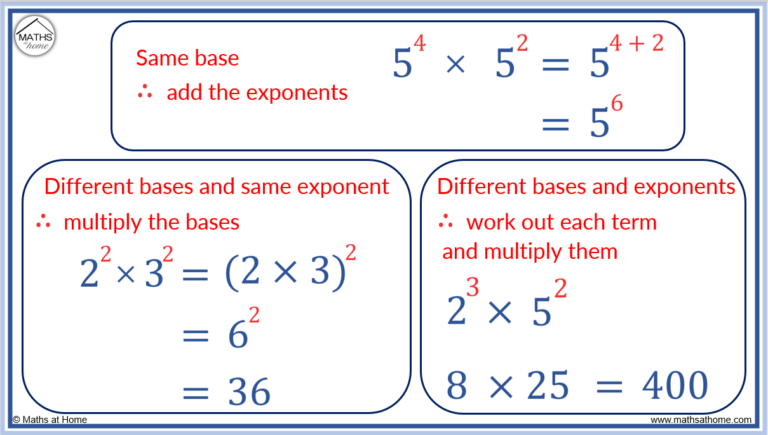
- Different Bases: When multiplying or dividing exponents with different bases, you can't simply add or subtract the exponents.
- Exponent of Zero: When any base is raised to the zero power, it becomes 1, but this rule doesn't apply if the base itself is zero (0).
🧮 Note: Understanding these exceptions will prevent potential errors in your calculations.
By mastering these fundamental rules, you'll unlock the ability to simplify complex expressions quickly, making your journey through algebra smoother. Remember that practice makes perfect, so the more you use these rules, the more fluent you'll become in exponent manipulation.
To wrap up, we've explored five essential rules for multiplying exponents, each with its own unique application. From adding exponents of the same base to handling negative or zero exponents, these rules pave the way for clear understanding and proficiency in algebra. Keep practicing, and the rules will become second nature, helping you navigate even the most challenging algebraic equations with ease.
What if I have different bases?

+
When bases are different, you cannot simply add or subtract the exponents. You’ll need to evaluate each base separately or look for common factors.
Why does a zero exponent equal one?

+
Any number raised to the power of zero is essentially dividing that number by itself, which equals 1 for all non-zero numbers.
How do negative exponents work?

+
Negative exponents mean that you flip the base to the denominator. For example, ( x^{-2} ) becomes ( \frac{1}{x^2} ).