Multiplying Decimals Made Easy With Area Models

If you've ever found yourself staring at a decimal multiplication problem, wondering where to even begin, you're not alone. Multiplying decimals can seem like a daunting task if traditional methods don't click for you. But what if there was a visual, intuitive way to understand and perform decimal multiplication? Enter area models, a method that breaks down complex arithmetic into manageable visual chunks. Let's dive into how area models can simplify multiplying decimals, making the process not only easier but also more educational.
What Are Area Models?

Area models are visual aids used to represent mathematical problems geometrically. Originally designed for whole number multiplication, they translate seamlessly to decimals. The fundamental concept involves:
- Partitioning the numbers into their components.
- Arranging these components into rectangles or squares.
- Calculating the area of these geometric shapes to find the product.
Why Use Area Models for Multiplying Decimals?

There are several compelling reasons to use area models when multiplying decimals:
- Visual Learning: Many learners grasp concepts better when they can see them. Area models provide a spatial representation that can aid understanding.
- Breaking Down Complexity: By splitting decimals into parts, area models make even the most complex multiplication look simple.
- Error Reduction: Visual aids help prevent common mistakes by offering a step-by-step approach to the problem.
- Learning Aid: For students learning the process, area models serve as a scaffold that gradually leads to a conceptual understanding of decimal multiplication.
Step-by-Step Guide to Multiplying Decimals Using Area Models
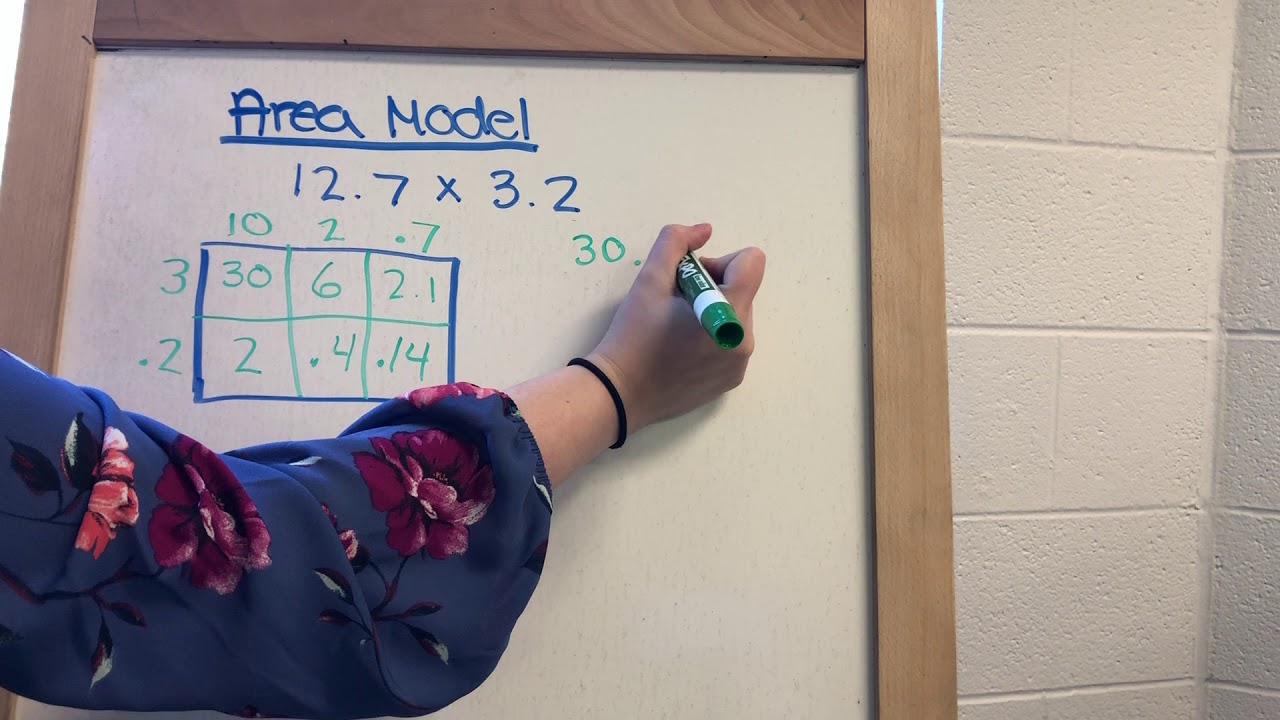
Here's how to use area models to multiply decimals:
Step 1: Identify and Prepare the Numbers

- Take the numbers you want to multiply, for example, 3.6 and 1.2.
- Ignore the decimal points for now; you'll be working with 36 and 12 initially.
Step 2: Create the Model

- Draw a rectangle divided into sections that represent your numbers.
- For 36 and 12, you'd have a rectangle split into:
- 10 units by 10 units = 100 square units
- 10 units by 2 units = 20 square units
- 3 units by 10 units = 30 square units
- 3 units by 2 units = 6 square units
| 10 | 2 | |
|---|---|---|
| 30 | 300 | 60 |
| 6 | 60 | 12 |

Step 3: Calculate Total Area

- Add all the areas together: 100 + 20 + 30 + 6 = 156 square units.
Step 4: Reintroduce the Decimal Points

- Count the total number of decimal places in the original problem. In our example, there are two decimal places (3.6 and 1.2).
- Place the decimal point in the result, counting from right to left.
So, the result of 3.6 multiplied by 1.2 using an area model is 4.32.
⚠️ Note: Ensure all sections of the rectangle are labeled correctly to avoid confusion when calculating the total area.
Practical Tips for Teaching Decimal Multiplication with Area Models

- Color Coding: Use different colors to distinguish between the parts of the number. This visual differentiation can help students keep track of where each number's part belongs in the rectangle.
- Gradual Difficulty Increase: Start with easy problems like multiplying a decimal by a whole number or even multiplying by a unit (10, 100). Gradually introduce more complex examples.
- Link with Real Life: Use real-world examples like the area of a garden or the size of a piece of fabric to contextualize the mathematics.
Overall, area models not only facilitate learning but also foster a deeper understanding of decimals and the concept of multiplication itself. Students can visually see how each part of a decimal contributes to the final product, leading to a more intuitive grasp of arithmetic operations.
Common Challenges and How to Overcome Them

Like any teaching method, area models come with their own set of challenges:
- Misalignment: Ensure that the rectangle's dimensions are set up correctly. Misalignment can lead to confusion.
- Overcomplication: Sometimes students might overcomplicate the process by adding too many divisions. Simplify the steps.
- Decimal Placement: Always double-check where the decimal point should be placed in the final product.
To address these issues, teachers and parents can:
- Regularly practice setting up the area models with a focus on correct alignment.
- Explain why the simplification of the process works, avoiding unnecessary complexity.
- Use mnemonic devices or consistent methods for remembering where to place the decimal point.
The Educational Benefits of Using Area Models

Integrating area models into teaching decimal multiplication has numerous educational advantages:
- Visual Problem Solving: They provide a visual representation of abstract mathematical problems, aiding in the transition from concrete to abstract thinking.
- Flexibility: Area models can be used for addition, subtraction, and even division, making them a versatile tool in mathematics education.
- Conceptual Understanding: Students aren't just learning the steps; they're understanding the 'why' behind the steps, which is crucial for mathematical literacy.
Over time, as students become more comfortable with area models, they can transition to using them for more complex operations, enhancing their numerical flexibility and confidence.
As we've explored, area models offer a visually intuitive approach to understanding decimal multiplication, which can unlock the door to mathematical understanding for many students. By visualizing the problem, the abstract becomes concrete, and arithmetic operations become less intimidating. When learners grasp the connection between numbers, their spatial relationship, and their geometric representation, they develop a solid foundation in not just the mechanics of multiplication but in the core principles of mathematics.
Can area models be used for other arithmetic operations?

+
Yes, area models can be applied to other operations like addition, subtraction, and division. They help visualize how these operations work by breaking numbers down into parts and showing how these parts interact spatially.
What if my students struggle with visualizing decimals?

+
Start with simple visual aids like grids or hundred squares. Use manipulatives or digital tools that allow students to manipulate visual representations of decimals, which can make the abstract concept more tangible.
How can I ensure my students are correctly placing the decimal point?

+
Practice counting the number of decimal places in the factors before multiplying, then after multiplication, count from right to left to place the decimal point. Repetition and visual cues will help in reinforcing this rule.
Is there a risk of over-reliance on area models?

+
The goal is not to replace standard methods but to support conceptual understanding. As students become comfortable, gradually introduce and transition to mental calculations or other methods alongside area models.