5 Easy Methods to Multiply Binomials Effectively

Multiplying binomials is a fundamental skill in algebra and calculus, crucial for solving equations and understanding polynomial behavior. This article provides a comprehensive guide on five effective methods to multiply binomials, ensuring students and enthusiasts can tackle these calculations with confidence. Let's dive into these methods and explore how they work.
1. The FOIL Method
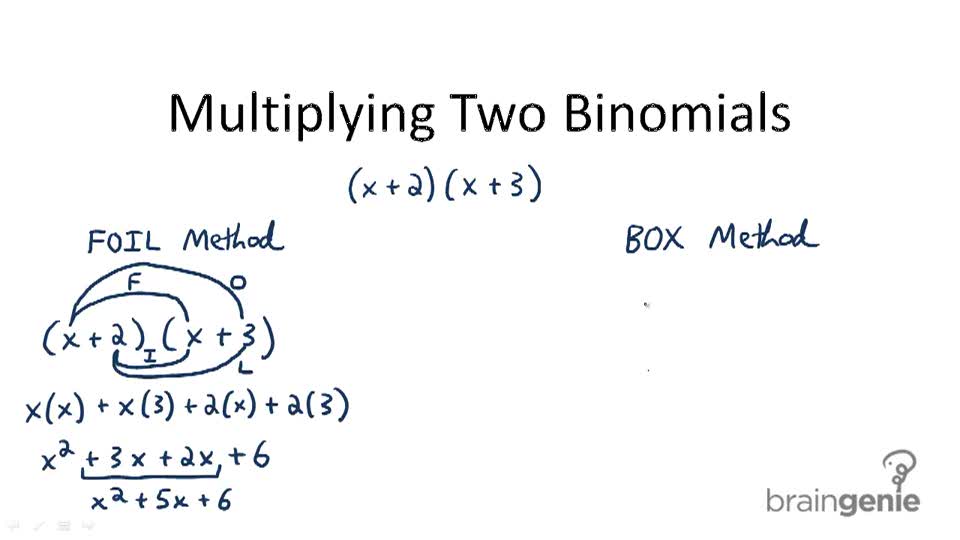

The FOIL method, which stands for First, Outer, Inner, Last, is a mnemonic to help remember the steps for multiplying two binomials. Here’s how it works:
- First: Multiply the first terms in each binomial.
- Outer: Multiply the outer terms in the product.
- Inner: Multiply the inner terms.
- Last: Multiply the last terms in each binomial.
📝 Note: FOIL is particularly effective for simple binomials like (x + 3)(x + 2).
2. The Grid or Table Method


For those who are visual learners, the grid method can be particularly helpful. Here’s how you do it:
| (x + a) | (y + b) | |
|---|---|---|
| (x + a) | x^2 | x * y |
| (y + b) | x * b | a * y + ab |
This method visually organizes the terms to be multiplied, making it easier to add up the products.
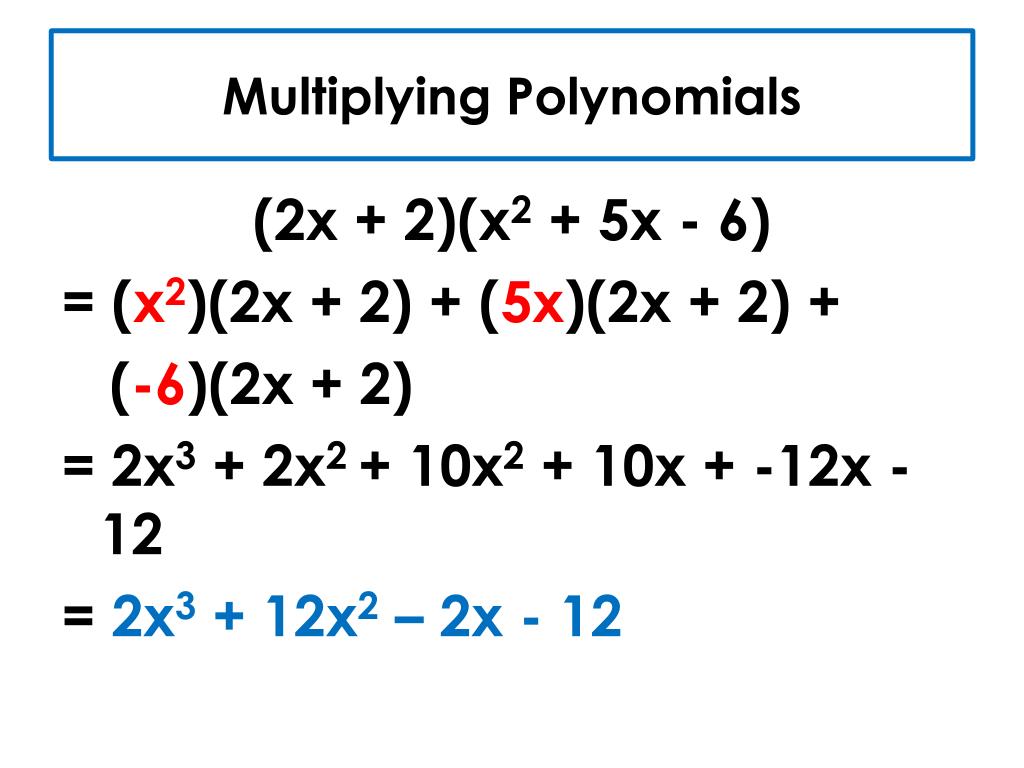
3. The Distributive Property

The distributive property allows you to expand binomials by multiplying each term in one binomial by each term in the other. Here’s the process:
- Distribute each term in the first binomial to each term in the second binomial.
- Add all the resulting products.
This method is versatile and can be applied to more complex expressions beyond simple binomials.
💡 Note: Remember, (a + b)(c + d) = ac + ad + bc + bd.
4. Squaring a Binomial

When you’re multiplying a binomial by itself, there’s a special formula known as the binomial square formula:
(a + b)^2 = a^2 + 2ab + b^2
This formula simplifies the process by directly giving you the expanded form without going through the multiplication steps.
5. Using Pascal’s Triangle
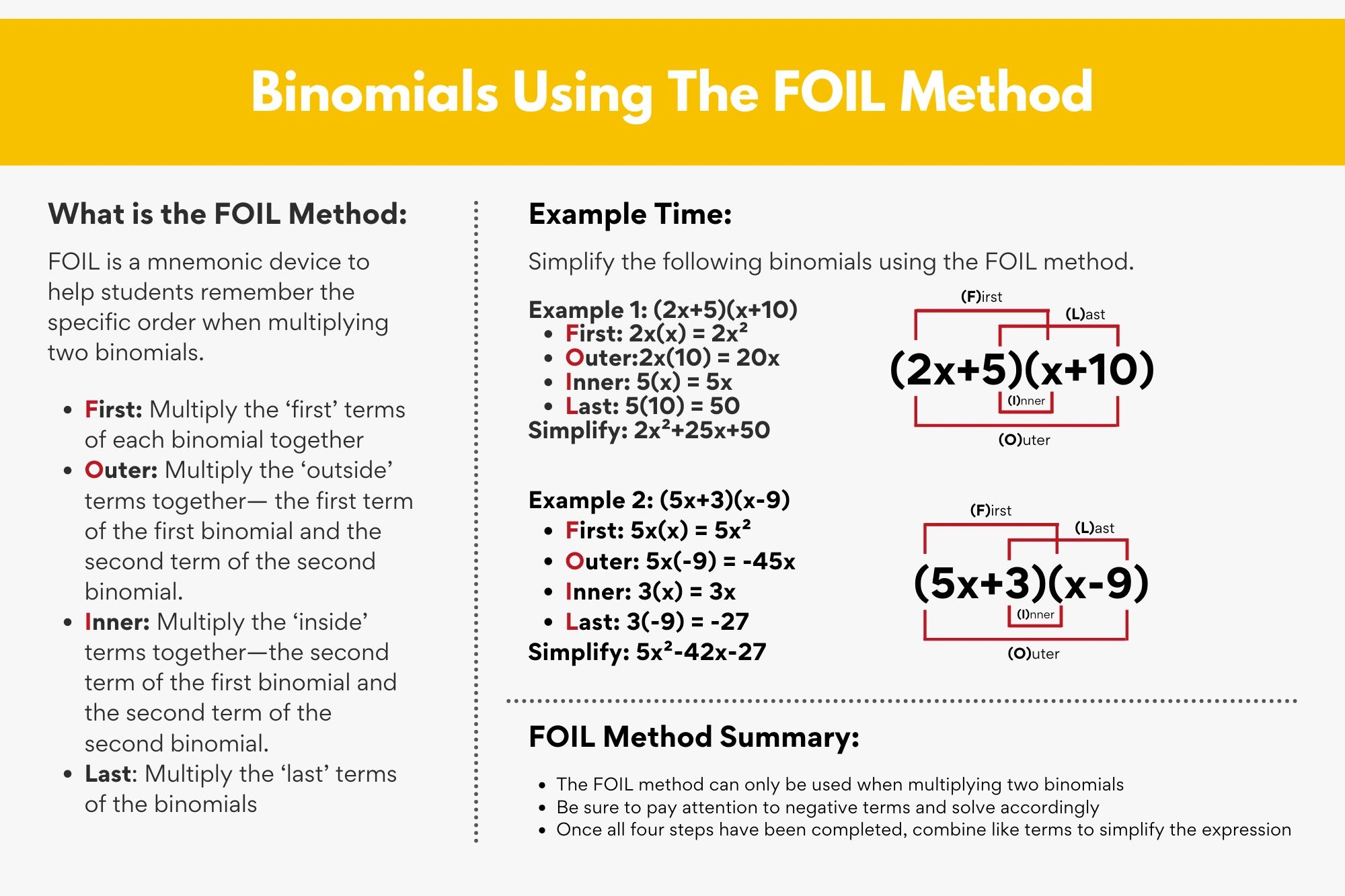

Pascal’s Triangle isn’t just a fun pattern; it’s a powerful tool for binomial expansion:
- Each row in Pascal’s Triangle corresponds to the coefficients of binomial expansions.
- Start from the second row for simple binomials (n=1), where the expansion would be 1, 2, 1.
- Multiply the numbers from Pascal’s Triangle by the respective powers of ‘a’ and ‘b’ in the binomial.
This method is beneficial when dealing with higher powers of binomials.
As we conclude, it's clear that mastering the multiplication of binomials opens up a plethora of possibilities in algebra and beyond. Each method has its unique advantages, from the structured approach of the FOIL method to the visual aid of the grid, the versatility of the distributive property, the direct application of squaring binomials, and the mathematical elegance of Pascal's Triangle. By understanding these methods, you empower yourself to handle binomial expressions with ease, aiding your progression in mathematical problem-solving. Here's to applying these techniques in real-world problems, enhancing your mathematical prowess!
Can I use the FOIL method for any binomial expression?

+
Yes, the FOIL method works for any binomial expression, but it is most effective for simple cases where the terms are easy to identify and multiply.
What is the advantage of using Pascal’s Triangle for binomial expansion?

+
Pascal’s Triangle provides an immediate visual reference for the coefficients in binomial expansions, especially useful when expanding binomials to higher powers without the need for extensive calculation.
Is there any method that is better for visual learners?

+
The grid method or the table method can be particularly beneficial for visual learners as it breaks down the multiplication into a structured grid format, making it easier to understand the process.
Why would you use the squaring a binomial method?

+
It’s quicker for squaring binomials because it directly gives you the expanded form using the formula, eliminating the need to go through multiplication manually.
How can these methods help in real-world applications?

+
These methods aid in solving equations in fields like engineering, physics, finance, and computer science where polynomials are used to model various phenomena and systems.