Foil Method Made Easy: Multiplying Binomials Practice Worksheet
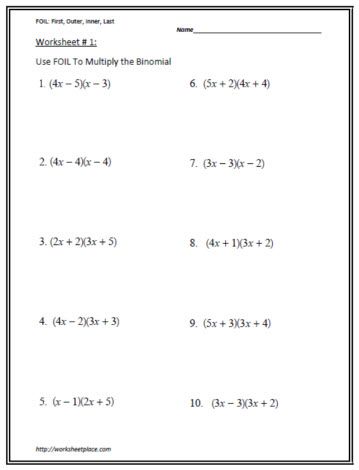
The Foil Method is a fantastic tool for multiplying binomials, helping students effortlessly handle algebra equations with just a simple mnemonic device. Whether you're a student grappling with algebra or a teacher looking for an effective way to explain it, understanding the FOIL method can greatly simplify multiplying two binomials. This article delves deep into the FOIL method, offering step-by-step instructions, practice worksheets, and important tips to ensure you master the concept of multiplying binomials with ease.
Understanding the FOIL Method

The FOIL method is an acronym that stands for First, Outer, Inner, Last. This mnemonic guides you through the process of multiplying two binomials:
- F: Multiply the first terms together.
- O: Multiply the outer terms together.
- I: Multiply the inner terms together.
- L: Multiply the last terms together.
Step-by-Step Guide to the FOIL Method

To demonstrate how FOIL works, let’s consider the example of multiplying two binomials like (x + 3)(x - 2):
- F: First terms – Multiply (x) with (x), resulting in x².
- O: Outer terms – Now, multiply (x) with (-2), getting -2x.
- I: Inner terms – Next, take (3) times (x), giving us 3x.
- L: Last terms – Finally, multiply (3) with (-2), which equals -6.
Adding all these products together:
[x² + (-2x) + 3x + (-6) = x² + x - 6.]⚠️ Note: When performing the FOIL method, remember to group like terms in the final result.
Why the FOIL Method Works

The FOIL method breaks down the distributive property of multiplication over addition in a way that’s easy to remember and apply. Here’s why it works:
- It uses the distributive law to ensure every term in one binomial multiplies with every term in the other binomial.
- The commutative property ensures that the order in which we multiply does not matter, so FOIL gives us the correct solution despite the specific sequence it suggests.
- Memorization - FOIL provides a structure for remembering how to approach binomial multiplication, making it easier for beginners to handle more complex problems.
Practice Worksheets
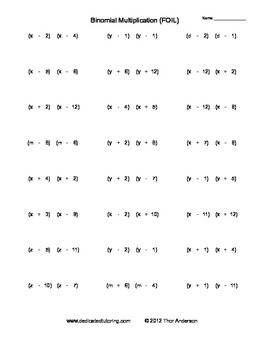
Here’s a practice table to help you solidify your understanding of the FOIL method:
| Problem | Solution |
|---|---|
| (x + 2)(x - 3) | x² + (-3x) + 2x + (-6) = x² - x - 6 |
| (a + 5)(a - 1) | a² + (-a) + 5a + (-5) = a² + 4a - 5 |
| (y - 4)(y + 7) | y² + (7y) + (-4y) + (-28) = y² + 3y - 28 |
| (2b - 3)(b + 4) | 2b² + (8b) + (-3b) + (-12) = 2b² + 5b - 12 |

✏️ Note: Try solving these problems on your own before checking the solutions. It's essential practice for mastering the FOIL method.
Common Mistakes and How to Avoid Them

While using the FOIL method, here are some common mistakes to watch out for:
- Forgot to Distribute – Ensure you distribute the multiplication throughout both binomials.
- Mixed Signs – Pay attention to the signs when multiplying. If you see a minus sign, it affects the term following it.
- Incorrect Grouping – Group like terms together correctly to avoid confusion or missed terms.
- Omitted Steps – Remember all four steps: First, Outer, Inner, Last. Skipping any can lead to incomplete results.
🛑 Note: Be methodical and take your time. The FOIL method is straightforward, but haste can lead to errors.
Advanced Techniques Beyond FOIL

Once you’re comfortable with the FOIL method, you might want to explore other methods for polynomial multiplication:
- Box Method or Grid Method - This visual method sets up a grid to multiply terms, offering an alternative for those who are more visual learners.
- Vertical Multiplication - Similar to how you multiply whole numbers vertically, this method can be applied to polynomials for easier calculation.
Here's a final summary to help you remember:
The FOIL method is a convenient tool for simplifying the multiplication of binomials. Its structured approach makes algebra more manageable, providing a clear path for beginners and a quick check for more seasoned students. With practice, you'll find that what once seemed complex becomes second nature, allowing you to solve polynomial multiplication problems with confidence.
What does the acronym FOIL stand for?

+
FOIL stands for First, Outer, Inner, Last. It’s a mnemonic to help remember how to multiply two binomials together.
Is the FOIL method limited to multiplying two binomials?

+
Yes, FOIL is specifically designed for the multiplication of two binomials. For trinomials or more complex polynomials, other methods like the distributive property or polynomial long division might be more suitable.
Can I use FOIL to expand trinomials?

+
The FOIL method works best with binomials. For trinomials or larger polynomials, consider using the distributive property or other polynomial multiplication methods.
Do I need to remember the FOIL method if there are other methods?
+
While there are alternative methods, FOIL is a quick, easy-to-remember way to handle binomial multiplication. It’s beneficial, especially for those starting with algebra.



