Master Multiplying 2 Digits by 1 Digit with Ease
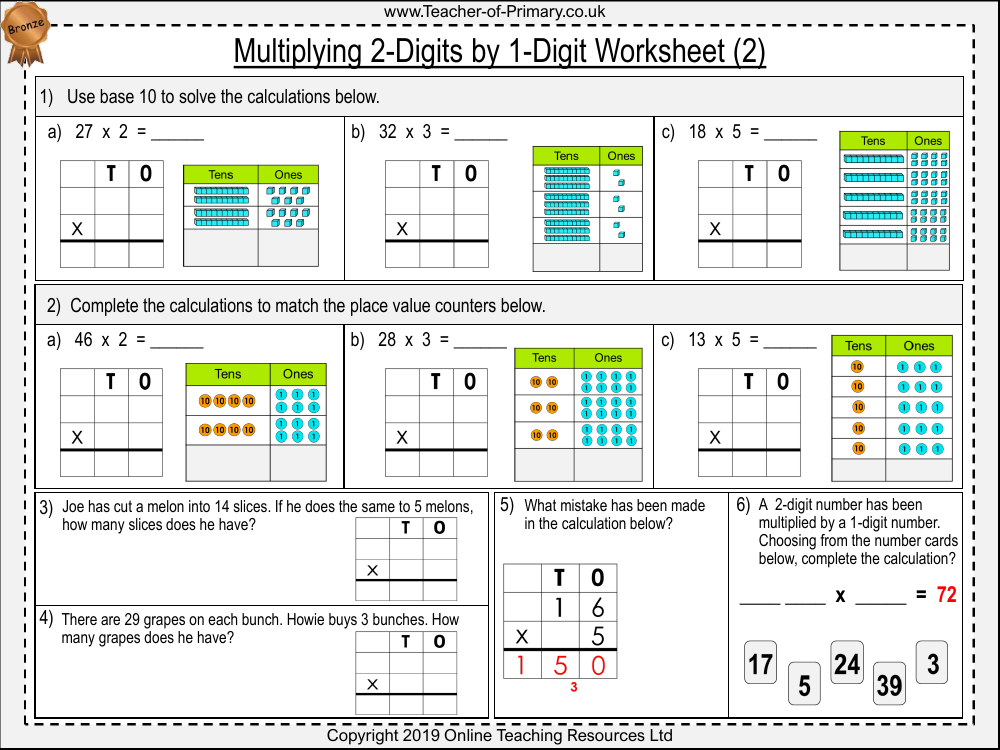
Whether you're a student learning basic arithmetic or an adult brushing up on your math skills, understanding how to efficiently multiply two-digit numbers by single-digit numbers is a fundamental skill. This skill not only speeds up your mental math but also lays the groundwork for tackling more complex multiplication problems. In this comprehensive guide, we'll explore various strategies to master multiplying two digits by one digit with ease.
Understanding the Basics

Before diving into complex strategies, let's ensure we have a solid understanding of basic multiplication:
- The concept of multiplication as repeated addition.
- Place value and how digits interact when multiplied.
- The distributive property of multiplication over addition, which can break problems into simpler steps.
Quick Recap of Multiplication Principles

| Place Value | Example | Result |
|---|---|---|
| Units | 5 x 3 | 15 |
| Tens | 30 x 3 | 90 |

📝 Note: Understanding the basic principles is crucial for developing efficient mental calculation strategies.
Strategies for Multiplying 2 Digits by 1 Digit

1. The Traditional Long Multiplication Method

This method involves:
- Writing down the two-digit number below the single digit number.
- Multiplying each digit of the two-digit number by the single digit, carrying over if necessary.
- Summing the results for the final answer.
Example: Let’s multiply 56 by 7.
- 5 * 7 = 35. Write down 5 and carry 3 over.
- 6 * 7 = 42. Add the carried 3 for a total of 45.
So, 56 * 7 = 392.
2. The Break-apart Strategy

This method leverages the distributive property to break the two-digit number into tens and units:
- 56 * 7 can be broken into (50 * 7) + (6 * 7).
- 50 * 7 = 350
- 6 * 7 = 42
Adding these together gives us 392.
3. The Double-Digit Times Tables
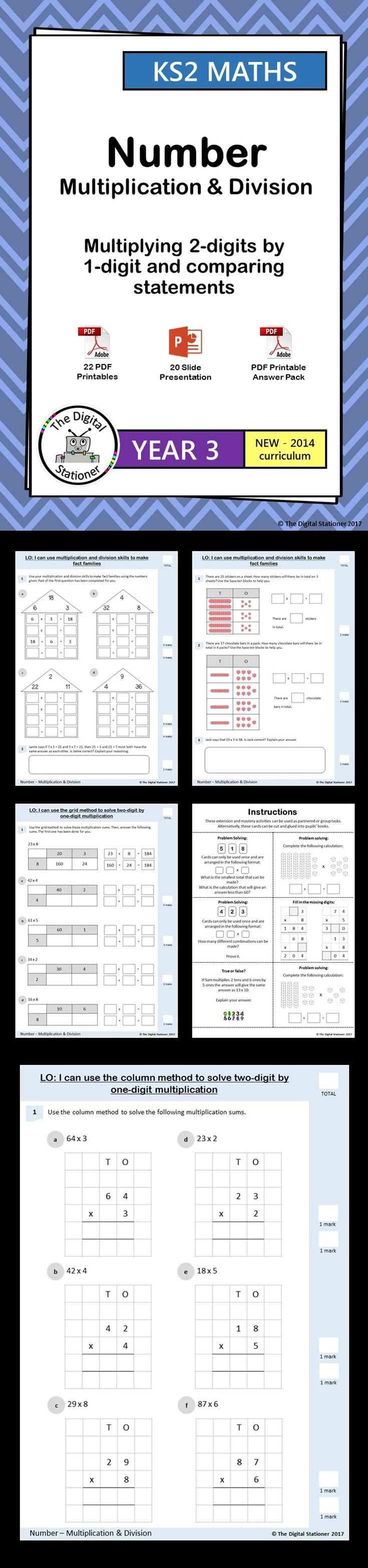
Memorizing double-digit times tables can be a game-changer. If you know the tables up to 20, you can:
- Quickly perform calculations without breaking numbers down.
- Use these tables as building blocks for larger calculations.
4. Multiplication Through Addition

This method involves:
- Adding the two-digit number to itself a number of times equal to the single digit.
Example: 56 * 7 would be 56 added 7 times.
5. Using Finger Multiplication

For quick, small multiplications, some people find finger multiplication techniques helpful:
- Assign digits to your fingers and perform multiplication steps using your fingers.
🚨 Note: While finger multiplication can be fun, it’s not always the most efficient for larger or frequent calculations.
Maximizing Your Multiplication Skills
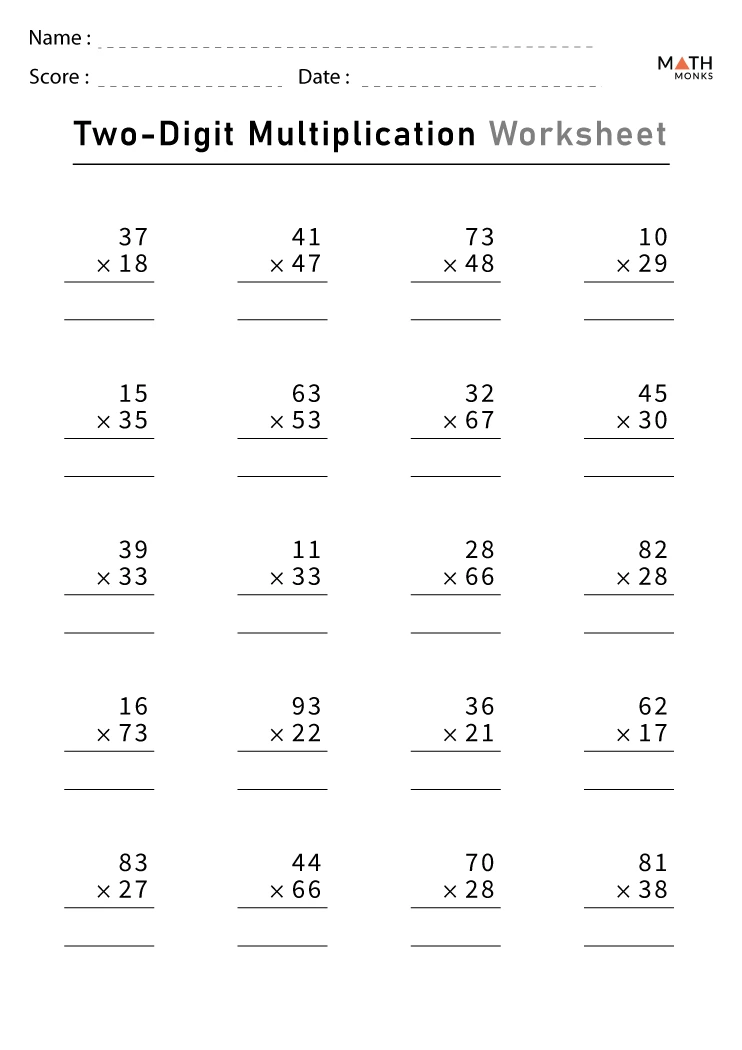
1. Practice Regularly

Repetition is key in mastering mental math. Here are some tips:
- Use flashcards or apps for daily practice.
- Challenge yourself with real-life calculations, like multiplying prices when shopping.
2. Utilize Technology

There are numerous online tools and apps designed to:
- Offer timed multiplication tests.
- Provide adaptive learning paths based on your skill level.
- Help track your progress and identify areas for improvement.
3. Understand and Apply Mental Math Tricks

Learning tricks like “casting out nines” can help you verify calculations or perform quick checks:
- Casting out nines is a quick way to check multiplication results by summing digits.
By mastering these strategies, you'll not only get quicker at basic multiplication but also more confident in your overall math abilities.
In the journey of mastering multiplication, we've covered essential strategies for multiplying two-digit numbers by a single-digit number. These techniques, from traditional methods to mental math tricks, provide a solid foundation for arithmetic proficiency. Whether you're calculating everyday prices, preparing for a test, or simply enjoying the challenge of numbers, these methods enhance speed, accuracy, and mental agility in mathematics. Remember, the key to mastery is consistent practice and understanding the underlying principles of multiplication. Keep these strategies in mind, and numbers will become your friends.
Why is it important to learn how to multiply?

+
Multiplication is fundamental in mathematics for calculating quantities, understanding concepts like area and volume, and forms the basis for advanced math like algebra and calculus. It’s also crucial for everyday life tasks involving calculations.
Are there any apps to practice multiplying?
+Yes, numerous apps like Math Bingo, Times Table Rockstars, and Squeebles offer interactive and engaging ways to practice multiplication tables and problem-solving skills.
How can I improve my mental multiplication speed?
+Practice regularly using flashcards, games, or timed tests. Understand patterns in multiplication (like doubles), use mental shortcuts, and continuously challenge yourself with larger numbers or more complex problems.
What’s the best strategy for multiplying for someone who struggles with math?
+The break-apart strategy can be particularly helpful as it divides the problem into simpler parts. Also, using visual aids like a multiplication table or chart can provide a reference to build confidence and understanding.
Can learning multiplication help in other areas of math?
+Absolutely. Multiplication is integral in algebra for solving equations, in geometry for calculating areas and volumes, and even in basic statistics for understanding probability and distributions.