Fun Worksheet: Multiplying Fractions and Whole Numbers Easily

Multiplying fractions and whole numbers is a fundamental skill in arithmetic, one that many students encounter early in their mathematical education. It's not just a matter of following a rote procedure; understanding why and how these operations work can greatly simplify the process and enhance conceptual learning. This blog post will delve into why understanding this multiplication process is essential, provide a step-by-step guide to multiplying fractions with whole numbers, and offer practical examples, tips, and tricks to make this mathematical operation as fun and easy as possible.
Understanding Multiplication of Fractions and Whole Numbers

Before diving into the mechanics, let’s grasp the underlying principle. A whole number can be thought of as a special fraction where the denominator is implicitly understood to be one (1). Hence, when you multiply a fraction by a whole number, you are essentially multiplying by this ‘whole number fraction’. Here’s the mathematical insight:
- Multiplication as Repeated Addition: Think of 4 times 1⁄3. This can be viewed as adding 1⁄3 four times. So, 4 × (1⁄3) = 1⁄3 + 1⁄3 + 1⁄3 + 1⁄3.
- Area Model: Imagine a rectangle. If one side is of length 1⁄3 and the other side is 4 units long, the area of this rectangle would be 4⁄3 or 1 and 1⁄3 when you multiply them.
💡 Note: The multiplication of a fraction by a whole number essentially scales the fraction, expanding its value proportionally.
Step-by-Step Guide to Multiplying Fractions by Whole Numbers

Here is how you can multiply a fraction by a whole number:
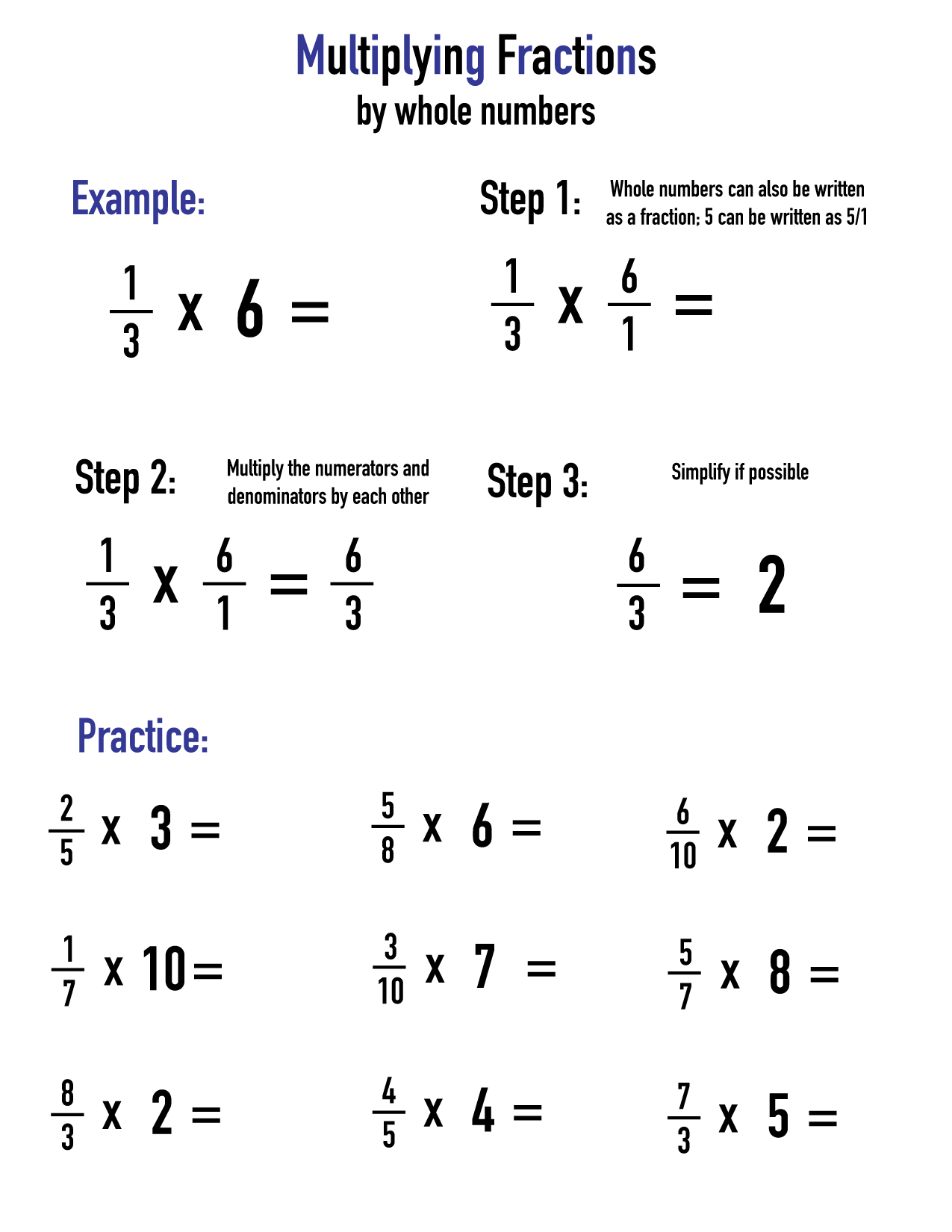
- Convert the Whole Number to a Fraction: Make the whole number into a fraction by giving it a denominator of 1. For instance, the whole number 5 becomes 5⁄1.
- Multiply Numerators Together: Take the numerator of the original fraction and multiply it by the numerator of the whole number fraction.
- Multiply Denominators Together: Now do the same for the denominators.
- Reduce the Result: If the resultant fraction can be simplified, simplify it to its lowest terms.
Let's walk through an example:
- Multiply 3/4 by 5
- Convert 5 to a fraction: 5 = 5/1
- Multiply numerators: 3 × 5 = 15
- Multiply denominators: 4 × 1 = 4
- Result: 15/4 or 3¾
Practical Examples and Exercises
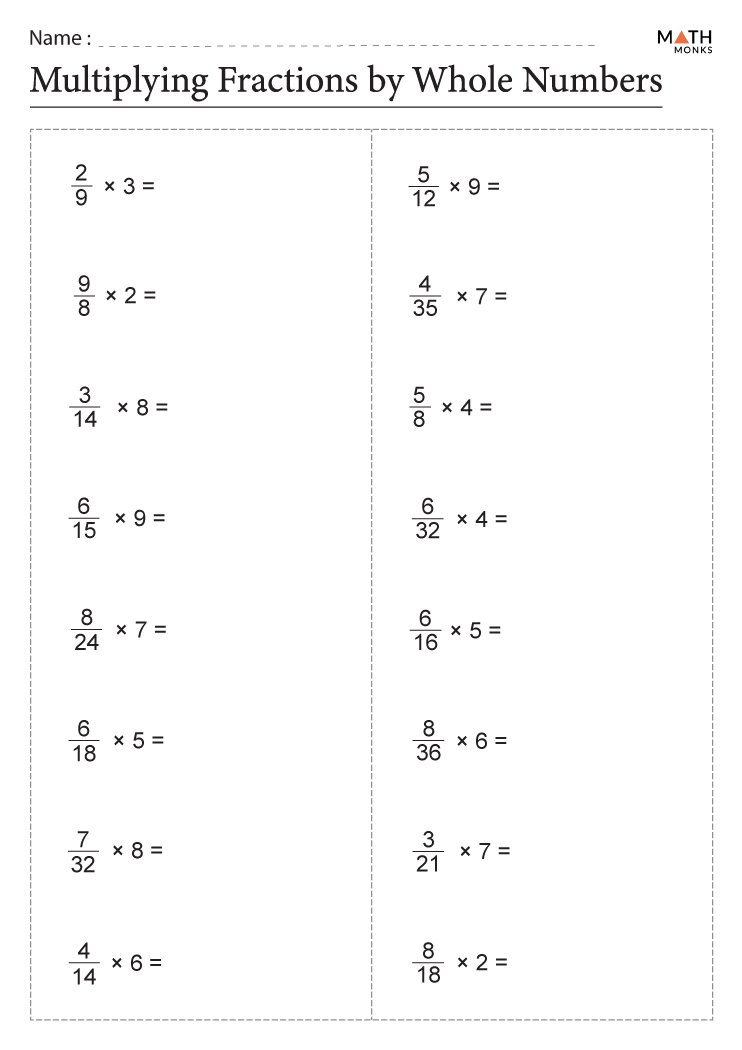
Here are some practical examples to work through:
- Multiply 2/5 by 3
- Multiply 7/9 by 6
- Multiply 5/8 by 4
| Original Fraction | Whole Number | Step-by-Step | Result |
|---|---|---|---|
| 2/5 | 3 | (2 * 3)/(5 * 1) = 6/5 | 1 and 1/5 |
| 7/9 | 6 | (7 * 6)/(9 * 1) = 42/9 | 4 and 2/3 |
| 5/8 | 4 | (5 * 4)/(8 * 1) = 20/8 | 2 and 1/2 |
Tips for Making Multiplying Fractions Fun and Easy

- Use Visual Aids: Draw fractions and multiply them visually to help understand the concept better.
- Practice with Real-life Scenarios: Incorporate multiplication into everyday activities like cooking or dividing items.
- Play Games: There are numerous math games online that can make multiplication an enjoyable activity.
- Memorize Shortcuts: For instance, multiplying by 1/2 or 1/3 can be taught with simple division tricks.
📝 Note: Understanding multiplication of fractions with whole numbers is not just about the arithmetic but also about the conceptual understanding that can apply to more complex mathematical scenarios.
As we move towards the end of our exploration into the world of fraction and whole number multiplication, let's recap some of the essential takeaways:
The beauty of mathematics lies in its simplicity when we grasp the underlying principles. Multiplying fractions and whole numbers is not only a fundamental skill but also a stepping stone to understanding more advanced mathematical concepts like algebra and calculus. By viewing whole numbers as fractions, the process becomes seamless, and understanding why the steps work allows for greater proficiency and enjoyment in math. Engaging with real-life problems and visual aids not only makes the learning process more fun but also deeply ingrains these mathematical ideas into our everyday thinking.
Why do we convert the whole number into a fraction before multiplying?

+
Converting a whole number to a fraction with a denominator of 1 allows for a uniform approach to multiplication, making the operation consistent with the rules for multiplying any two fractions.
Can we multiply fractions by whole numbers directly?

+
Yes, you can skip the conversion step if you understand the concept behind it. Multiplying the numerator by the whole number and keeping the denominator the same is essentially the same as converting the whole number to a fraction and multiplying numerator with numerator.
Is there a real-life application for multiplying fractions by whole numbers?

+
Absolutely! In scenarios like cooking, where you might need to scale a recipe up or down; in construction, for calculating materials; or even in daily chores like dividing a chore into equal parts, multiplying fractions by whole numbers becomes practical and useful.