5 Ways to Multiply Fractions by Whole Numbers

Multiplying fractions by whole numbers is a fundamental concept in mathematics, and it’s essential to understand the different ways to do it. In this article, we’ll explore five ways to multiply fractions by whole numbers, along with examples and notes to help you master this skill.
Method 1: Multiply the Numerator by the Whole Number

One way to multiply a fraction by a whole number is to multiply the numerator (the top number) by the whole number. This method is straightforward and easy to understand.
Example:
Multiply 3⁄4 by 5
To solve this problem, multiply the numerator (3) by the whole number (5):
3 × 5 = 15
So, the answer is 15⁄4.
📝 Note: When multiplying a fraction by a whole number, the denominator (the bottom number) remains the same.
Method 2: Convert the Whole Number to a Fraction

Another way to multiply a fraction by a whole number is to convert the whole number to a fraction by putting it over 1. This method is helpful when you need to multiply a fraction by a large whole number.
Example:
Multiply 2⁄3 by 6
To solve this problem, convert the whole number (6) to a fraction (6⁄1):
2⁄3 × 6⁄1 = 12⁄3
Simplify the fraction by dividing both numbers by 3:
12 ÷ 3 = 4 3 ÷ 3 = 1
So, the answer is 4⁄1, which is equal to 4.
Method 3: Use Visual Models

Visual models, such as diagrams or drawings, can help you understand the concept of multiplying fractions by whole numbers. This method is especially helpful for visual learners.
Example:
Multiply 1⁄2 by 3
Draw a diagram to represent the fraction 1⁄2:
Imagine you have 1⁄2 of a pizza, and you want to multiply it by 3. You can draw 3 identical pizzas, each representing 1⁄2 of a pizza:
Since each pizza represents 1⁄2, you can combine the 3 pizzas to get 3⁄2.
So, the answer is 3⁄2.
Method 4: Use Real-World Examples
Using real-world examples can make it easier to understand the concept of multiplying fractions by whole numbers. This method is helpful when you need to apply math to everyday situations.
Example:
You have 1⁄4 of a tank of gas in your car, and you want to know how much gas you’ll have if you multiply it by 4.
Since you have 1⁄4 of a tank, multiplying it by 4 means you’ll have 4 times as much gas:
1⁄4 × 4 = 4⁄4
Simplify the fraction by dividing both numbers by 4:
4 ÷ 4 = 1 4 ÷ 4 = 1
So, the answer is 1, which means you’ll have a full tank of gas.
Method 5: Use the Multiplication Property of Equality
The multiplication property of equality states that if you multiply both sides of an equation by the same number, the equation remains true. This method is helpful when you need to multiply a fraction by a whole number in an equation.
Example:
Solve for x: 1/2x = 3
To solve for x, multiply both sides of the equation by 2 (the denominator):
2 × 1/2x = 2 × 3
This simplifies to:
x = 6
So, the answer is x = 6.
As you can see, there are several ways to multiply fractions by whole numbers. By understanding these different methods, you’ll become more confident and proficient in your math skills.
Remember, practice makes perfect, so be sure to try out these methods with different examples to reinforce your understanding.
Multiplying fractions by whole numbers is an essential skill in mathematics. By mastering this skill, you’ll be able to solve a wide range of problems and apply math to real-world situations.
We hope this article has helped you understand the different ways to multiply fractions by whole numbers. With practice and patience, you’ll become proficient in this skill and be able to tackle more challenging math problems.
What is the easiest way to multiply fractions by whole numbers?
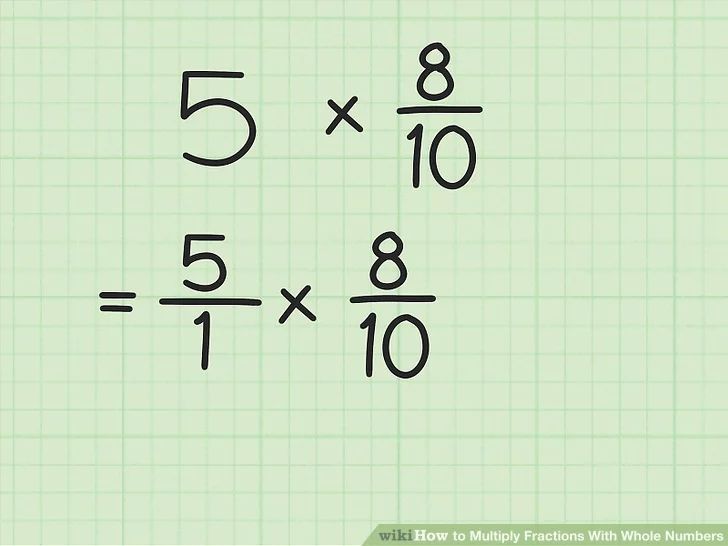
+
The easiest way to multiply fractions by whole numbers is to multiply the numerator (the top number) by the whole number.
Can I use visual models to multiply fractions by whole numbers?

+
Yes, visual models can be a helpful way to understand the concept of multiplying fractions by whole numbers, especially for visual learners.
What is the multiplication property of equality?

+
The multiplication property of equality states that if you multiply both sides of an equation by the same number, the equation remains true.
Related Terms:
- multiplying fractions with answer key
- multiplying fractions worksheets with answers
- multiplying fractions worksheets grade 4



