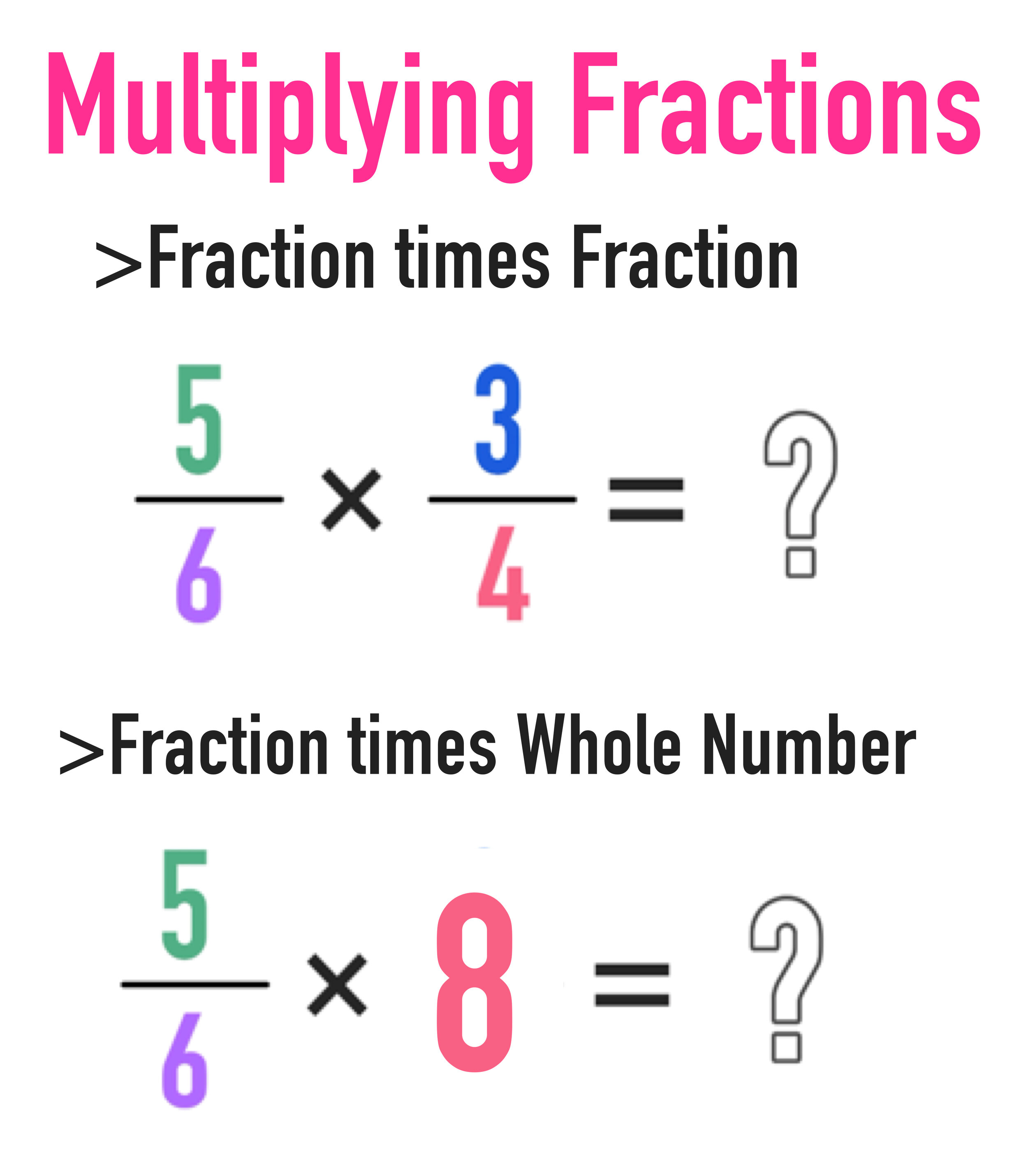
5 Ways to Multiply Fractions by Whole Numbers Easily

Fractions are a foundational concept in mathematics, and understanding how to manipulate them, especially when it comes to operations involving whole numbers, can significantly enhance your mathematical proficiency. Multiplying fractions by whole numbers might seem daunting at first, but with the right strategies, it becomes straightforward and even intuitive. Let's dive into five effective ways to multiply fractions by whole numbers, making this arithmetic task much easier.
Method 1: Convert the Whole Number to a Fraction

The simplest approach to multiplying fractions by whole numbers is to convert the whole number into a fraction. Here’s how:
- Take the whole number and put it over 1, e.g., 3 becomes 3⁄1.
- Multiply this new fraction by the given fraction. For instance, if you are multiplying 2⁄5 by 3, you would calculate 2⁄5 × 3⁄1.
- Multiply the numerators together and the denominators together to get a new fraction: 2 × 3⁄5 × 1 = 6⁄5.
💡 Note: Converting the whole number into a fraction ensures you are working with the same mathematical structure, simplifying the multiplication process.
Method 2: Use the Dot Method

The dot method, or the lattice method, can make the multiplication of fractions visually clear and is particularly useful when dealing with complex fractions:
- Draw two lines perpendicularly and place the fraction at the intersection. If multiplying 2⁄5 by 3, write '2' and '5' on one line and '3' and '1' on the other.
- Multiply the numbers at the intersection of each line. So you'll get 2 × 3⁄5 × 1.
- The products are written in the quadrants formed by the intersection.
This visual representation can help understand the process for those who are more visually oriented.
Method 3: Simplify Before Multiplying
Before you start multiplying, simplifying the fraction or even the whole number can make your calculations easier:
- If you're multiplying 2⁄10 by 5, simplify 2⁄10 to 1⁄5 first.
- Now multiply 1⁄5 by 5, which simplifies to 1 since any number divided by itself is 1.
- This method reduces the complexity of the numbers involved in the multiplication.
Method 4: Use a Number Line

For a more tangible understanding, especially for younger students or visual learners, using a number line can be effective:
- Draw a number line from 0 to the whole number you are multiplying by.
- Divide each segment into the number of parts as denoted by the denominator of the fraction.
- Move along the number line by the numerator of the fraction for each whole number jump. For example, to multiply 2⁄3 by 6, you'd jump 2/3 for each of the 6 segments.
This method visually demonstrates the concept of adding fractions repeatedly.
Method 5: Break Down the Problem

Breaking down the multiplication into simpler steps can also be an effective strategy:
- Consider 3⁄4 by 7. You could think of this as:
- Breaking it down into smaller multiplications, e.g., 3⁄4 × 4 (which equals 3), and then:
- Multiplying that result by the remaining part of the whole number (in this case, 3 by 7).
- This method can help manage larger numbers more easily.
By employing these methods, you'll find that multiplying fractions by whole numbers can be approached in various ways, each with its own merits depending on the context and personal preference. Whether you choose to convert the whole number into a fraction, use visual aids like the dot method or a number line, simplify first, or break down the problem, these techniques offer flexibility and understanding that cater to different learning styles.
Each method has been chosen for its ability to break down complex mathematical operations into manageable steps or to present the problem in a way that resonates with visual, logical, or kinesthetic learning preferences. As you become more comfortable with these methods, you'll find that you can switch between them seamlessly, choosing the one that best suits the particular fraction or whole number combination at hand.
In mathematical learning, it's essential not just to solve problems but to understand the underlying principles. These multiplication techniques not only provide practical solutions but also reinforce the fundamental concepts of fractions and whole numbers, providing a deeper comprehension of number theory and arithmetic.
Ultimately, the ability to multiply fractions by whole numbers confidently opens doors to more complex mathematical concepts, such as algebra, calculus, and beyond, where such foundational skills play a pivotal role in solving real-world problems. Whether you're a student, educator, or just someone brushing up on their math skills, mastering these techniques will certainly prove beneficial in your mathematical journey.
What if the fraction is improper when multiplying with a whole number?

+
Multiply the whole number by the improper fraction as usual, then simplify or convert the result back to a mixed number if necessary.
Can these methods be applied to negative fractions or whole numbers?

+
Absolutely! Just follow the same rules for multiplying negatives as with positive numbers, keeping in mind the product of two negative numbers is positive.
Is there a quick method for fractions where both the numerator and denominator are the same?
+
Yes, when the numerator equals the denominator (e.g., 1⁄1), multiplying by any number results in that number itself.



