7 Ways to Solve Multiple Step Equations
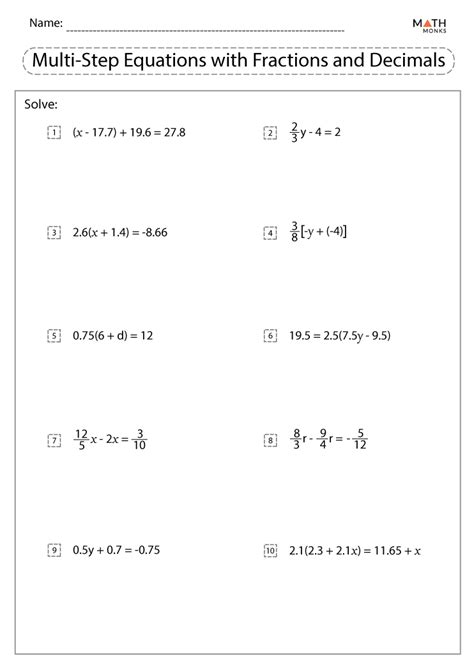
Understanding Multiple Step Equations
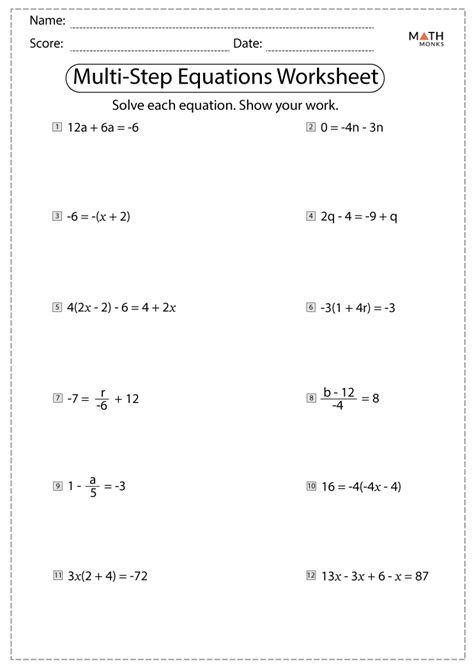
Multiple step equations are a type of mathematical equation that requires more than one step to solve. These equations involve a combination of addition, subtraction, multiplication, and division operations, and often require the use of inverse operations to isolate the variable. In this article, we will explore seven ways to solve multiple step equations, with a focus on practical examples and step-by-step explanations.
Method 1: Simplifying the Equation
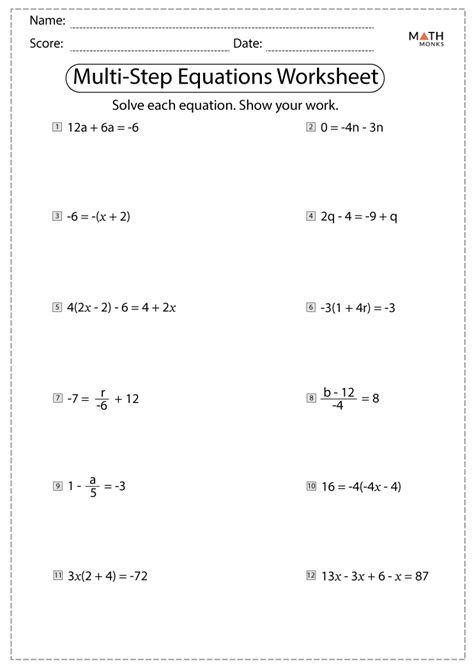
The first method for solving multiple step equations is to simplify the equation by combining like terms. This involves adding or subtracting the same variables or constants on both sides of the equation.
📝 Note: Always simplify the equation before attempting to solve it.
For example, consider the equation:
2x + 5 = 11
To simplify this equation, we can combine the constant terms on the right-hand side:
2x = 11 - 5 2x = 6
Now, we can solve for x by dividing both sides by 2:
x = 6 ÷ 2 x = 3
Method 2: Using Inverse Operations

The second method for solving multiple step equations is to use inverse operations to isolate the variable. Inverse operations are opposite operations that “undo” each other, such as addition and subtraction, or multiplication and division.
For example, consider the equation:
x - 3 = 7
To solve this equation, we can use the inverse operation of addition to isolate x:
x = 7 + 3 x = 10
Method 3: Working with Fractions
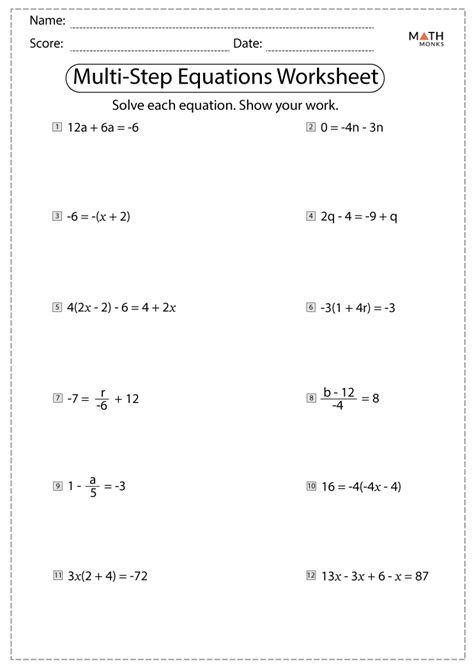
The third method for solving multiple step equations is to work with fractions. This involves multiplying or dividing both sides of the equation by a fraction to eliminate the fraction.
For example, consider the equation:
x/4 = 9
To solve this equation, we can multiply both sides by 4 to eliminate the fraction:
x = 9 × 4 x = 36
Method 4: Using the Distributive Property

The fourth method for solving multiple step equations is to use the distributive property. The distributive property states that a single term can be distributed to multiple terms inside parentheses.
For example, consider the equation:
2(x + 3) = 12
To solve this equation, we can use the distributive property to expand the parentheses:
2x + 6 = 12
Now, we can solve for x by subtracting 6 from both sides and then dividing by 2:
2x = 12 - 6 2x = 6 x = 6 ÷ 2 x = 3
Method 5: Solving Equations with Decimals

The fifth method for solving multiple step equations is to work with decimals. This involves multiplying or dividing both sides of the equation by a power of 10 to eliminate the decimal.
For example, consider the equation:
x - 2.5 = 4.8
To solve this equation, we can add 2.5 to both sides to isolate x:
x = 4.8 + 2.5 x = 7.3
Method 6: Using Algebraic Properties

The sixth method for solving multiple step equations is to use algebraic properties, such as the commutative, associative, and identity properties.
For example, consider the equation:
x + 2 = 9
To solve this equation, we can use the commutative property to rearrange the terms:
x = 9 - 2 x = 7
Method 7: Checking Your Solution

The seventh and final method for solving multiple step equations is to check your solution. This involves plugging your solution back into the original equation to ensure that it is true.
📝 Note: Always check your solution to ensure that it is correct.
For example, consider the equation:
x - 3 = 7
If we solve for x and get x = 10, we can plug this solution back into the original equation to check:
10 - 3 = 7 7 = 7
This confirms that our solution is correct.
What is a multiple step equation?

+
A multiple step equation is a type of mathematical equation that requires more than one step to solve.
What is the distributive property?

+
The distributive property states that a single term can be distributed to multiple terms inside parentheses.
Why is it important to check your solution?

+
Checking your solution ensures that it is correct and helps to avoid errors.
Solving multiple step equations requires a combination of mathematical operations, algebraic properties, and critical thinking. By following these seven methods, you can develop a deeper understanding of how to solve these types of equations and become more confident in your mathematical abilities.
Related Terms:
- Multiple step equations worksheet pdf
- multi step equations free worksheets
- multi step equation practice pdf
- printable multi step equation practice
- multi step equations work sheet
- multi step equation worksheet pdf