Momentum Worksheet Answers: Boost Your Physics Skills

Understanding momentum is crucial for anyone delving into the realm of physics, be it students in high school or those at university. This fundamental concept lies at the heart of mechanics, a part of physics that deals with the motion of objects under the influence of forces. The concept of momentum, along with its conservation, provides insights into how objects move, interact, and why they behave in certain predictable ways. In this blog post, we'll explore various aspects of momentum, providing not only momentum worksheet answers but also a deep dive into why momentum matters.
What is Momentum?

At its core, momentum, denoted by p, is a measure of the motion of an object. It is calculated using the formula:
[ p = m \times v ]
- m stands for mass, measured in kilograms (kg).
- v stands for velocity, measured in meters per second (m/s).
This equation shows us that momentum is a vector quantity - it has both magnitude and direction. Here are some key points about momentum:
- Momentum depends not just on how fast something is moving but also on how much mass it has. A small object moving very fast can have the same momentum as a large object moving slowly.
- The direction of momentum is the same as the direction of the object's velocity.
Conservation of Momentum
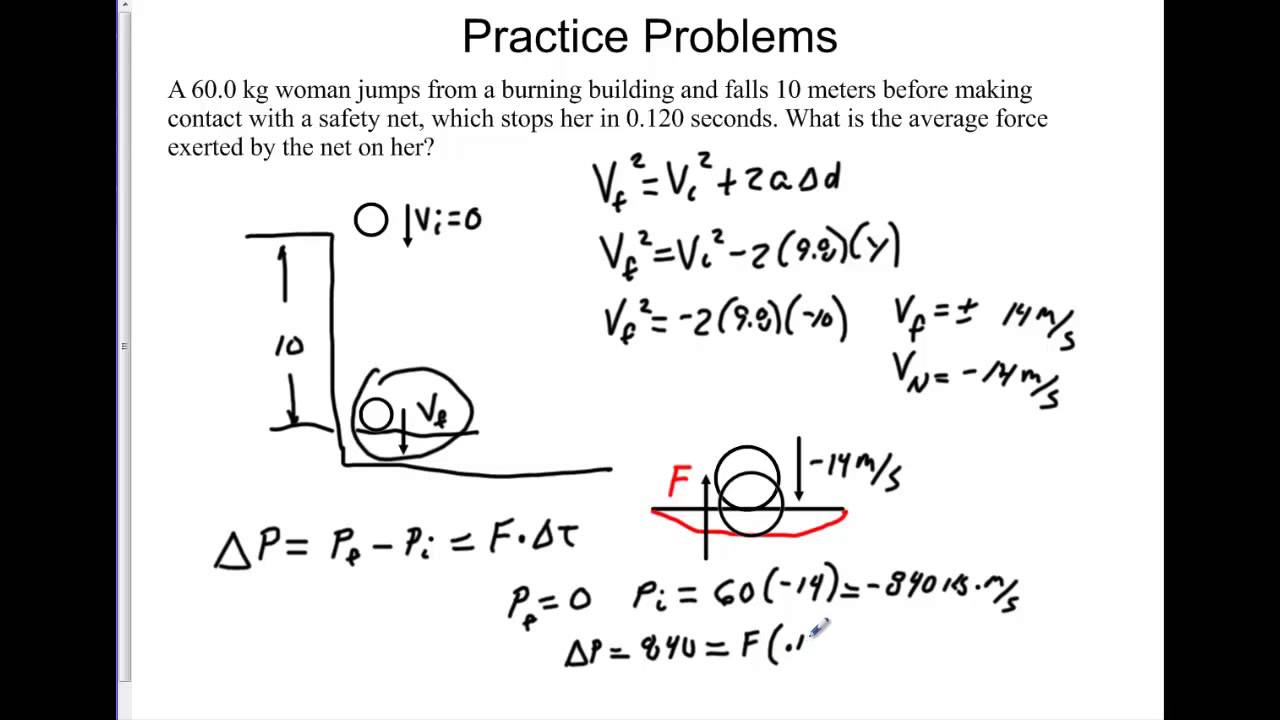
One of the most compelling aspects of momentum is its conservation. The law of conservation of momentum states that the total momentum of a closed system of interacting objects remains constant provided no external forces act on the system. Here are some applications:
- Collisions: In a collision, the total momentum before the collision equals the total momentum after the collision.
- Explosions: If a system initially at rest explodes, the pieces will move in such a way that their total momentum sums to zero.
Let's delve into an example:
| Scenario | Initial Momentum (kg·m/s) | Final Momentum (kg·m/s) |
|---|---|---|
| Two cars collide head-on | +200 | -200 |
| A bomb explodes in a stationary vehicle | 0 | 0 |

Notice how, in both cases, the total momentum before and after the event is conserved.
🔍 Note: While momentum is conserved in an isolated system, kinetic energy might not be, especially in inelastic collisions where energy can be converted into other forms like heat or sound.
Momentum Worksheet Answers

Let's tackle some typical momentum problems you might find on a worksheet or exam:
1. Calculating Momentum

- A 50 kg object moves with a velocity of 10 m/s. What is its momentum?
The answer:
[ p = m \times v = 50 \times 10 = 500 \text{ kg·m/s} ]
2. Conservation of Momentum in Collisions
- Two skaters, initially at rest, push off from each other. Skater A of mass 70 kg moves left at 3 m/s. What is the velocity of Skater B with a mass of 60 kg?
The answer:
- Initial momentum is zero (both skaters are at rest).
- Conservation of momentum states: m_A \times v_A + m_B \times v_B = 0
- Substituting the given values: 70 \times 3 + 60 \times v_B = 0
- Solving for v_B: v_B = -\frac{70 \times 3}{60} = -3.5 \text{ m/s}
- Skater B moves to the right at 3.5 m/s.
Here's a breakdown of the calculation:
| Skater | Mass (kg) | Velocity (m/s) | Momentum (kg·m/s) |
|---|---|---|---|
| A | 70 | -3 | -210 |
| B | 60 | 3.5 | 210 |
| Total | 0 |
3. Explosions and Conservation of Momentum

- A stationary object of mass 100 kg explodes into two pieces, one of which moves left at 5 m/s. If the mass of this piece is 60 kg, what's the velocity of the other piece?
The answer:
- The initial momentum of the system is zero.
- The mass of the second piece would be 100 - 60 = 40 kg.
- Conservation of momentum dictates: m_{left} \times v_{left} + m_{right} \times v_{right} = 0
- Substituting values: 60 \times (-5) + 40 \times v_{right} = 0
- Solving for v_{right}: v_{right} = \frac{60 \times 5}{40} = 7.5 \text{ m/s}
Practical Applications of Momentum

Understanding momentum isn't just about solving problems on paper; it has many real-world applications:
- Transportation: From the design of airbags that increase the time of impact to reduce the force on a person, to the stopping of trains which require momentum considerations for safety.
- Sports: Players often use their understanding of momentum when blocking or tackling in contact sports, or when hitting a ball with a racquet or bat to maximize the transfer of momentum.
- Engineering: Engineers calculate momentum for designing structures that can withstand impacts or for systems where momentum transfer must be managed, like in crash tests or space missions.
Wrapping Up

Momentum plays a pivotal role in our understanding of the physical world. It helps explain phenomena ranging from simple everyday events to complex interactions in the cosmos. Through this post, we've covered what momentum is, its conservation, and provided momentum worksheet answers to practical problems. It's not just about the answers but about developing a physical intuition for how and why things move the way they do. Whether you're studying momentum for an exam, out of curiosity, or to enhance your work, the principles of momentum offer valuable insights into the mechanics of motion.
🚀 Note: Momentum isn't just a classroom concept; it's woven into the fabric of our daily lives, influencing technology, sports, and safety mechanisms.
Why is momentum conserved?

+
Momentum is conserved due to Newton’s third law, which states that for every action, there is an equal and opposite reaction. When two objects interact, the forces they exert on each other are equal in magnitude but opposite in direction, resulting in the total momentum before and after the interaction being the same.
Can momentum be negative?

+
Yes, momentum can be negative. Momentum is a vector quantity, so its direction is significant. A negative sign indicates that the momentum is in the opposite direction to the positive direction chosen in the coordinate system.
How does momentum relate to kinetic energy?

+
Momentum and kinetic energy are related but different. Momentum ((p = m \times v)) depends on mass and velocity, while kinetic energy ((KE = \frac{1}{2}m v^2)) depends on mass and the square of velocity. Momentum is conserved in all collisions, while kinetic energy is only conserved in elastic collisions; in inelastic collisions, some kinetic energy is converted into other forms of energy.



