5 Momentum and Collision Worksheet Answers You Need
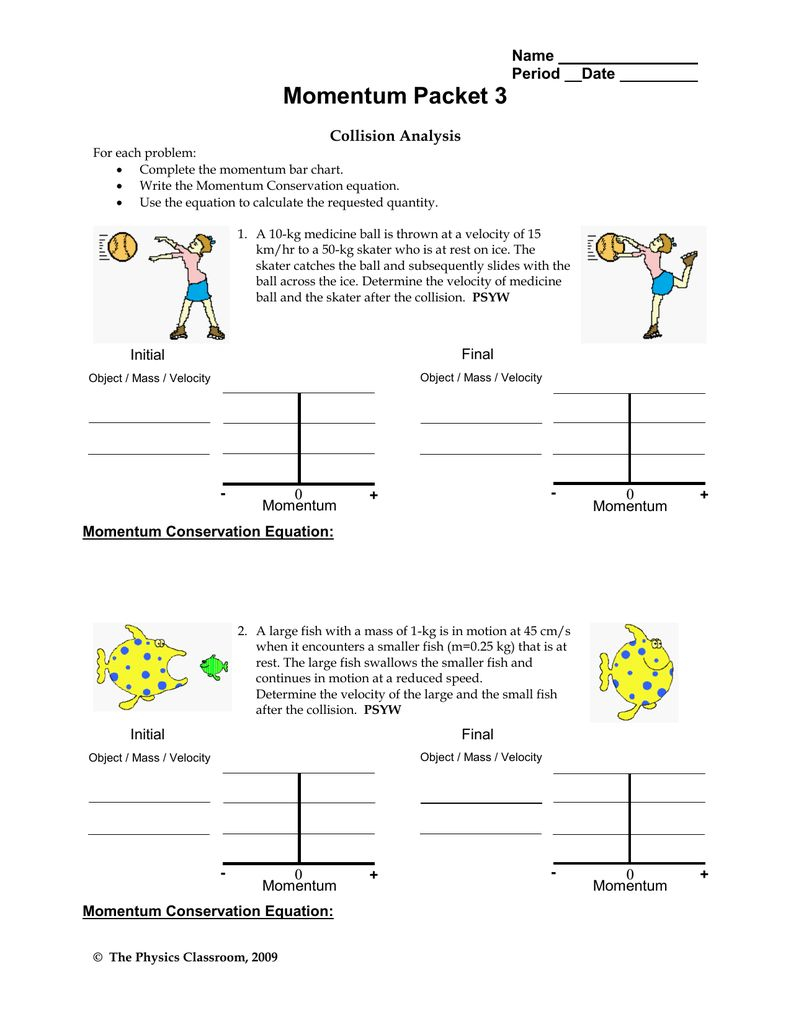
Hey there, physics enthusiasts! Whether you're trying to ace your high school physics exam or you're just looking to sharpen your understanding of one of physics' most fundamental topics—momentum and collisions—we've got you covered. In this post, we're diving deep into a variety of exercises, complete with answers, to help you master the principles of momentum and collision.
The Basics of Momentum

Before we delve into complex problems, let's first understand the basics:
- Momentum (p) is defined as the product of an object's mass (m) and its velocity (v): p = m * v. It's a vector quantity, meaning it has both magnitude and direction.
- Momentum is conserved in isolated systems, which forms the backbone of our study of collisions.
Here's a simple table to recap:
| Concept | Formula | Description |
|---|---|---|
| Momentum | p = m * v | Mass times velocity |
| Conservation of Momentum | ∑pinitial = ∑pfinal | Total momentum remains constant in an isolated system |

Understanding Collisions

Collisions can be categorized into:
- Elastic Collisions: Both momentum and kinetic energy are conserved.
- Inelastic Collisions: Momentum is conserved, but kinetic energy is not.
- Perfectly Inelastic Collisions: Objects stick together after collision, still conserving momentum but losing kinetic energy to other forms like heat or sound.
Worksheet Solutions

1. Conservation of Momentum in a Linear Collision

Let's take a look at an example where two carts are moving towards each other on a frictionless track:
Suppose Cart A with a mass of 3 kg is moving at 2 m/s towards Cart B, which has a mass of 2 kg and is moving at 4 m/s in the opposite direction. After the collision, Cart B is observed to move at 3 m/s in the direction initially taken by Cart A. Calculate the final velocity of Cart A.
Here's how we solve it:
- Use the conservation of momentum: mA * vAinitial + mB * vBinitial = mA * vAfinal + mB * vBfinal
- Plug in the known values: (3 kg * 2 m/s) + (2 kg * -4 m/s) = 3 kg * vAfinal + (2 kg * 3 m/s)
- Solve for vAfinal: 6 kg⋅m/s - 8 kg⋅m/s = 3 kg * vAfinal + 6 kg⋅m/s
- vAfinal = -2 m/s (Negative indicates opposite direction)
📌 Note: Remember that velocity is a vector, so direction matters!
2. Elastic Collision: Using the Elastic Collision Equations

Consider two billiard balls with equal masses colliding head-on. One ball comes in with a velocity of 5 m/s, and the other is at rest. After the collision, both balls rebound with velocities opposite their initial directions. Calculate the post-collision velocities.
Here's how to find the answer:
- Apply the elastic collision equations: v1final = (m1 - m2)/(m1 + m2) * v1initial + (2 * m2)/(m1 + m2) * v2initial and v2final = (2 * m1)/(m1 + m2) * v1initial + (m2 - m1)/(m1 + m2) * v2initial
- Since both balls have the same mass and one was at rest:
- v1final = (-5 + 0) / 2 = -2.5 m/s
- v2final = (2 * 5) / 2 = 5 m/s
So, the ball that was at rest now moves with a velocity of 5 m/s, and the incoming ball rebounds at -2.5 m/s.
3. Energy in Collisions
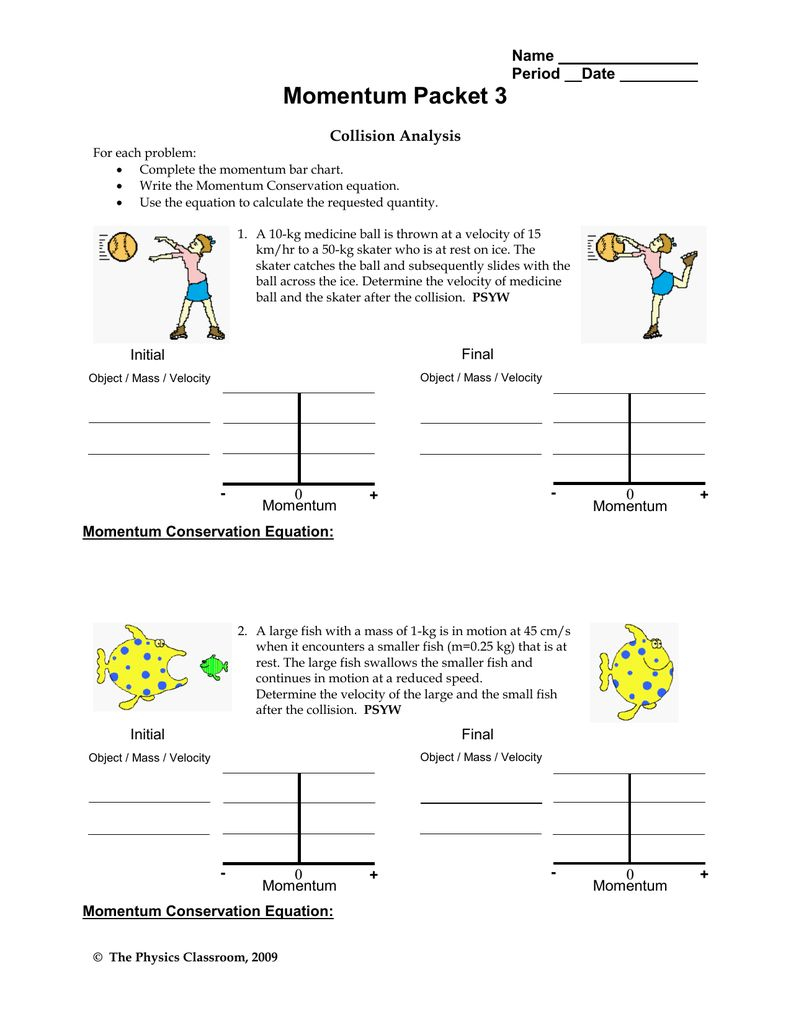
Let's look at how energy plays out in collisions:
Two cars (Car 1 and Car 2) collide head-on. Car 1 (1000 kg) is traveling at 10 m/s, and Car 2 (1500 kg) is traveling at -8 m/s. After a perfectly inelastic collision, they stick together. Calculate their common velocity after the collision and discuss the energy transformation.
To solve this:
- Use the conservation of momentum: m1 * v1initial + m2 * v2initial = (m1 + m2) * vfinal
- vfinal = (1000 kg * 10 m/s + 1500 kg * -8 m/s) / (1000 kg + 1500 kg)
- vfinal = 10000 kg⋅m/s - 12000 kg⋅m/s / 2500 kg = -0.8 m/s (negative sign means direction)
Regarding energy:
- The initial kinetic energy of Car 1 is: 0.5 * 1000 kg * (10 m/s)^2 = 50000 J
- The initial kinetic energy of Car 2 is: 0.5 * 1500 kg * (8 m/s)^2 = 48000 J
- After the collision, kinetic energy of the system: 0.5 * 2500 kg * (-0.8 m/s)^2 = 800 J
- Total initial kinetic energy = 98000 J, Final kinetic energy = 800 J
The large drop in kinetic energy suggests that a significant amount of energy has been converted into other forms such as sound, heat, and deformation energy.
In our journey through the mysteries of momentum and collisions, we've covered some key concepts:
- What momentum is, and how it's conserved in various types of collisions.
- How to calculate post-collision velocities in elastic and inelastic scenarios.
- Understanding the transformation of kinetic energy during collisions.
Remember, momentum is not just a concept to grasp for a physics exam; it's a principle that explains how objects interact with each other in the real world. Whether it's understanding how to maximize the safety features of vehicles or predicting the outcome of a sports game, these principles are foundational. Keep practicing with these worksheets, as they provide a framework for deepening your understanding of not only physics but also of natural phenomena around us.
What happens to kinetic energy in elastic vs. inelastic collisions?

+
In an elastic collision, both momentum and kinetic energy are conserved. The total kinetic energy after the collision equals that before the collision. In an inelastic collision, while momentum is conserved, kinetic energy is not; it gets converted into other forms of energy such as heat or deformation.
Can the final momentum of a system be greater than the initial momentum?

+
No, in an isolated system where no external forces act, the total momentum before a collision must equal the total momentum after the collision. The principle of conservation of momentum dictates this.
How does the mass of an object affect its momentum?

+
Momentum is directly proportional to mass. If an object has greater mass, it will have greater momentum for the same velocity, and vice versa.
Why is it important to know the direction in momentum calculations?

+
Momentum is a vector quantity, meaning it has both magnitude and direction. Correctly identifying the direction is crucial for accurately calculating the sum of momenta, especially in vector addition scenarios.
What role does kinetic energy play in collisions?

+
Kinetic energy gives insights into how energy is distributed and transformed in a collision. While momentum must be conserved, kinetic energy can either be conserved (elastic collisions) or partially converted to other forms of energy (inelastic collisions), which impacts how we analyze collision outcomes.



