5 Essential Tips for Solving Molarity Problems

The complexity of solving molarity problems can often seem daunting, but with a clear understanding of the foundational principles and application of a few essential strategies, these calculations become straightforward. Molarity, the measure of solute concentration in a solution, is central to chemistry, allowing us to quantify how much solute is dissolved in a solvent to form a solution. Here are five essential tips that will help you master solving these common yet perplexing chemistry problems.
1. Know Your Units


First and foremost, understanding and correctly using units is critical in solving molarity problems. Molarity (M) is defined as moles of solute per liter of solution:
M = moles of solute / Volume of solution in liters
Here are key points to keep in mind:
- Units of Moles: A mole is a unit of amount of substance, measuring 6.022 x 10^23 molecules or atoms. When converting grams to moles, you must use the molecular weight of the solute.
- Volume Units: Ensure that the volume of the solution is in liters. If the problem provides volume in milliliters (mL), remember that 1000 mL equals 1 liter.
Understanding the units helps in avoiding errors when performing calculations. Also, always check your final answer for the correct units; an answer with improper units is incorrect by default.
2. Use Dimensional Analysis

Dimensional analysis, or the factor-label method, can be a powerful tool in tackling molarity problems. This technique uses conversion factors to ensure that your units cancel out correctly, leading you to the desired quantity. Here’s how it works:
- Start with what you know: If you’re given grams of solute, convert it to moles using the molar mass of the compound.
- Convert the volume to liters if it isn’t already in liters.
- Set up your equation, ensuring that the units cancel out properly to leave you with the unit of molarity (M).

Example:
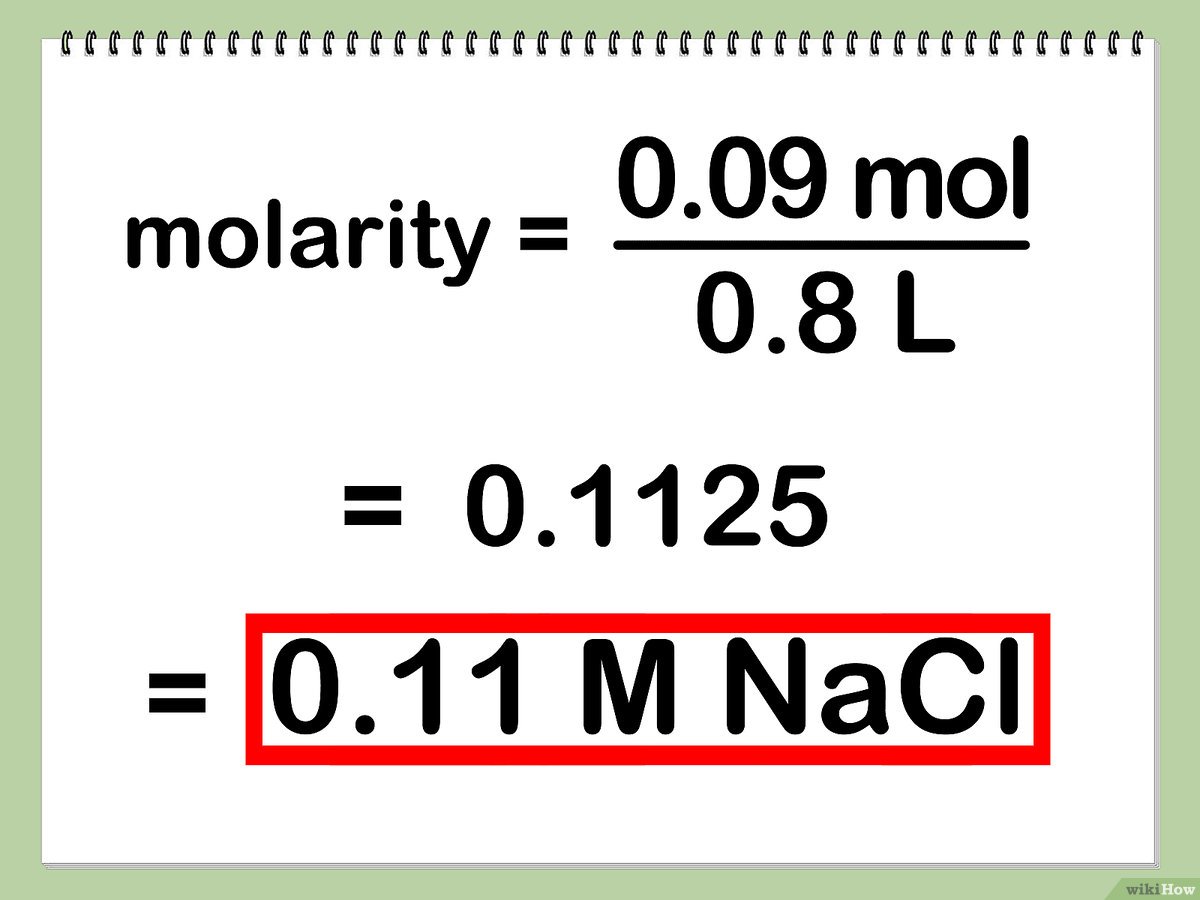
Given 58.44 grams of sodium chloride (NaCl) in 0.5 liters of solution:
58.44 g NaCl * (1 mol / 58.44 g) = 1 mol NaCl
Then, molarity is calculated as:
1 mol / 0.5 L = 2 M
Dimensional analysis ensures you’re always on track with your calculations.
3. Visualize the Problem

Visual aids can greatly enhance your understanding of the problem. When you’re dealing with a molarity problem:
- Draw the volume of the solution.
- Sketch the number of moles of solute.
- Visualize the relationship between the solute and solvent to better comprehend the concept of concentration.

These simple diagrams can make the abstract concept of concentration more tangible, helping you grasp how changes in either solute or solvent quantities affect molarity.
4. Practice Dimensional Analysis with Conversion Factors

Enhance your proficiency with dimensional analysis by practicing with various conversion factors:
- Convert moles to grams using the molar mass.
- Convert volume units (mL to L and vice versa).
- Use density to relate mass and volume for pure substances or solute concentrations in certain cases.
Here’s a simple table to help you remember common conversion factors:
| Quantity | Unit | Conversion Factor |
|---|---|---|
| Moles | Moles → Grams | 1 mole = Molar Mass in grams |
| Volume | Milliliters → Liters | 1 L = 1000 mL |
| Density | Grams → Volume | Density = mass / volume |

💡 Note: Familiarity with these factors will help you quickly adapt to different problem scenarios.
5. Utilize Dilution Calculations
Many molarity problems involve diluting a stock solution to create a new concentration. Here’s how to approach this:
- Use the dilution equation: M₁V₁ = M₂V₂, where:
- M₁ is the molarity of the initial solution.
- V₁ is the volume of the initial solution.
- M₂ is the molarity of the diluted solution.
- V₂ is the volume of the diluted solution.
This equation reflects the conservation of moles in the solution before and after dilution:

Example:
To make 100 mL of a 0.5 M solution from a 2 M solution:
M₁ = 2 M, V₁ = ?, M₂ = 0.5 M, V₂ = 100 mL
Solve for V₁:
V₁ = (M₂ * V₂) / M₁ = (0.5 M * 100 mL) / 2 M = 25 mL
💡 Note: You can also find V₁ using algebraic manipulation or by understanding that the total number of moles remains constant.
Summing up, mastering molarity problems hinges on understanding the fundamental units, using dimensional analysis effectively, visualizing the problem, practicing with different conversion factors, and knowing how to apply dilution calculations. These tips provide a structured approach to navigating these chemical calculations, making even complex molarity problems manageable and systematic.
What is the difference between molarity and molality?

+
Molarity (M) is the concentration of a solute in terms of moles per liter of solution, whereas molality (m) is the concentration in terms of moles of solute per kilogram of solvent.
Why do we need to convert units when solving molarity problems?

+
Unit conversion ensures all quantities are in consistent units, which is crucial for accurate calculations and avoiding errors in dimensional analysis.
How do I prepare a specific molarity solution?

+
To prepare a solution with a specific molarity, first determine the volume and concentration required, then calculate the required grams of solute using its molar mass and the molarity formula.