Unlocking Mechanical Advantage: Your Ultimate Worksheet Guide

In a world where efficiency reigns supreme, understanding mechanical advantage isn't just an academic pursuit; it's a cornerstone of practical engineering and everyday problem-solving. Whether you're a student grappling with physics homework, a hobbyist trying to build the perfect gadget, or an engineer fine-tuning complex machinery, a solid grasp of how simple machines amplify force or increase the distance over which work is done can propel your understanding to new heights. In this comprehensive worksheet guide, we'll delve into the intricacies of mechanical advantage, explore practical examples, and provide you with tools and worksheets to master this essential concept.
Understanding Mechanical Advantage

Mechanical advantage (MA) quantifies the amplification of force achieved by using a mechanical device, effectively reducing the input force required to perform work. This principle is pivotal in simple machines like levers, pulleys, gears, and inclined planes. Here's a brief overview:
- Levers: Represented as a rigid bar pivoted at a fulcrum, levers distribute force according to the distance from the fulcrum to where the force is applied and where the load is lifted.
- Pulleys: These systems can change the direction of the force and multiply the effort by using multiple wheels or sheaves.
- Gears: A simple machine where the gear ratio provides a mechanical advantage, affecting rotational speed and torque.
- Inclined Planes: Enable heavy loads to be moved up a slope with less force than lifting them vertically.
Mechanical Advantage in Different Machines

The beauty of mechanical advantage lies in its versatility. Each type of simple machine applies this principle in unique ways:
Levers

There are three classes of levers, each with its method of mechanical advantage:
- First Class: The fulcrum is between the effort and the load, like a seesaw or a pair of scissors.
- Second Class: The load is between the fulcrum and the effort, as seen in wheelbarrows or nutcrackers.
- Third Class: The effort is between the load and the fulcrum, like in tweezers or baseball bats, sacrificing force for increased range of motion.
⚠️ Note: In third-class levers, the mechanical advantage is less than 1, meaning the effort force must be greater than the load force to perform work.
Pulleys

Pulleys can be simple or compound, with mechanical advantage depending on:
- Number of pulleys in the system.
- How the rope or cable wraps around the pulleys.
Here’s a basic formula for a simple pulley system:
| Number of Strings Holding the Load | Mechanical Advantage |
|---|---|
| 1 | 1 |
| 2 | 2 |
| 3 | 3 |

Gears

When two gears mesh, their mechanical advantage is calculated by dividing the number of teeth on the output gear by the number on the input gear:
Mechanical Advantage = Number of Teeth on Output Gear / Number of Teeth on Input Gear
⚙️ Note: Larger gears turning smaller gears will increase speed but decrease torque, while smaller gears turning larger gears will increase torque but decrease speed.
Worksheet and Practical Applications

Now, let's bring this theory into practical usage:
Worksheet Components

- Definition and Formula: Explain what mechanical advantage is and how to calculate it for different machines.
- Levers Worksheet: Include calculations of mechanical advantage for each class of lever with diagrams.
- Pulley System Worksheet: Identify pulley configurations and calculate MA.
- Gears and Torque: Exercises to calculate gear ratios and understand their effects on force and speed.
- Practical Scenarios: Provide real-life scenarios for students to apply their knowledge.
Sample Questions and Answers

Here are a few sample questions you might include:
- Question: What is the mechanical advantage of a first-class lever with an effort arm length of 50 cm and a load arm length of 25 cm?
- Answer: MA = Effort Arm Length / Load Arm Length = 50 / 25 = 2
- Question: If a 50 kg load is raised by a pulley system with a mechanical advantage of 3, what effort is required?
- Answer: Effort = Load / MA = 50 / 3 ≈ 16.67 kg (in this case, effort is usually measured in force, like Newtons, but here it's simplified for clarity).
The final words on this intricate subject are both a reflection and an encouragement. We've journeyed through the realm of mechanical advantage, exploring its applications in various simple machines. The formulas, calculations, and practical scenarios we've discussed not only prepare you for academic challenges but also empower you with a deeper understanding of how the world around us functions. By mastering these principles, you're not just solving worksheets; you're equipping yourself with the knowledge to make mechanical systems work more efficiently, reducing effort and enhancing productivity. Remember, mechanical advantage isn't just about physics—it's about progress and practical applications in daily life, from the smallest tools to the largest structures. Keep exploring, keep applying, and let this guide be your stepping stone towards greater mechanical understanding and innovation.
What does a mechanical advantage greater than 1 signify?

+
A mechanical advantage greater than 1 means that the machine allows you to apply less effort to move a larger load, thus amplifying your input force.
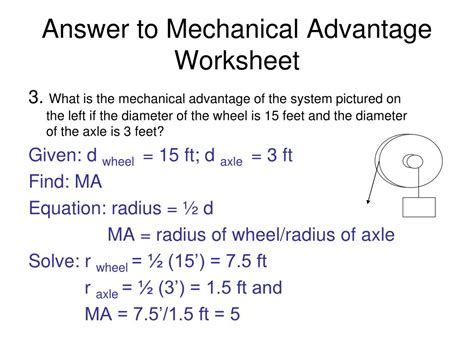
Why do third-class levers have a mechanical advantage of less than 1?
+
Third-class levers trade force for distance. The effort applied must be greater than the load because the load arm is longer than the effort arm, making it useful for tasks requiring a larger range of motion.
How can pulleys increase the mechanical advantage?

+
Pulleys can multiply your effort by creating multiple strands of the rope pulling on the load. The more strands, the higher the mechanical advantage.
Does a machine with a mechanical advantage always make work easier?

+
While mechanical advantage reduces the force needed, it often requires a longer distance of movement, meaning the trade-off can be in terms of speed or distance, not just effort.