Mean Value Theorem: Simplified Worksheet with Examples

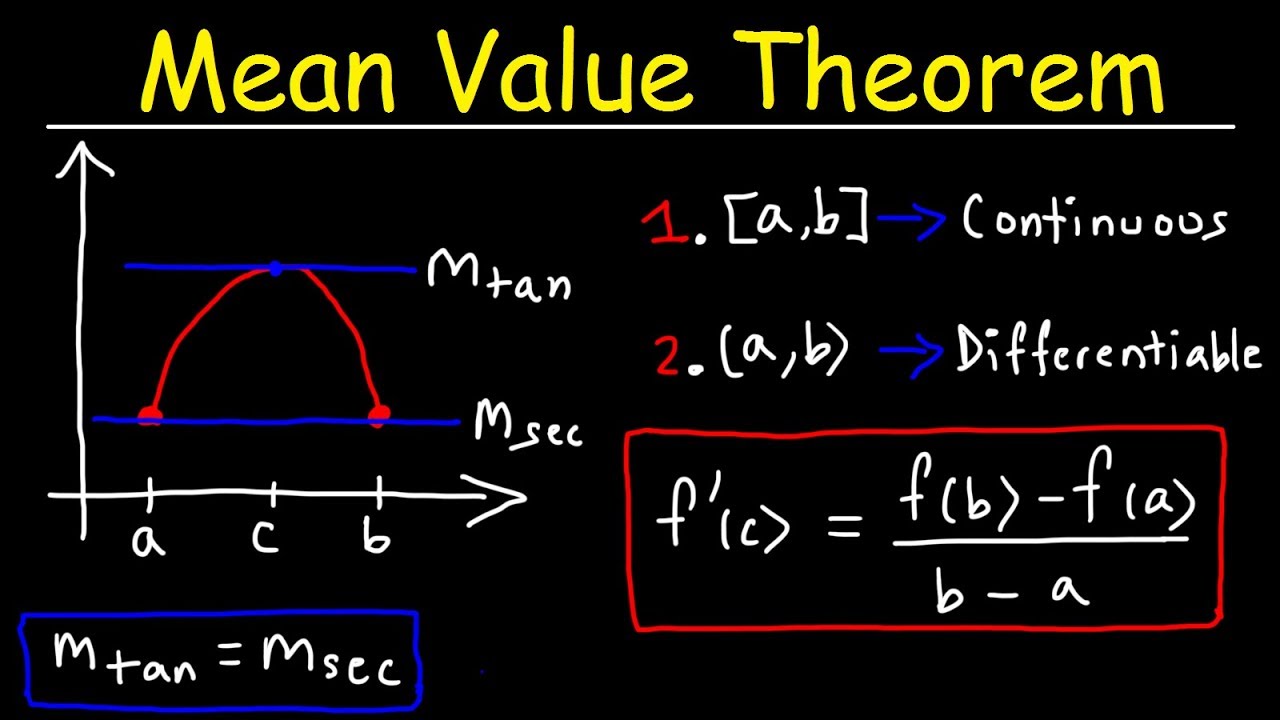
The Mean Value Theorem (MVT) is a cornerstone in calculus that connects the average rate of change of a function over an interval to its instantaneous rate of change at some point within that interval. This fundamental theorem not only provides a deep insight into the behavior of continuous and differentiable functions but also plays a pivotal role in proving many other theorems in calculus.

What is the Mean Value Theorem?

The Mean Value Theorem states that for any function ( f(x) ) which is:

• Continuous on the closed interval ([a, b])
• Differentiable on the open interval ((a, b))

There exists at least one point (c) in the open interval ((a, b)) where:

Understanding the Theorem with an Example

To better understand the MVT, let’s consider a real-world example. Imagine you are driving your car, and you set out for a 100 km trip. Suppose you leave your house at 2 PM and reach your destination at 6 PM, making the average speed for this journey 25 km/h.

However, your instantaneous speed at any given moment might differ from this average speed. According to the Mean Value Theorem, there must have been at least one point during your trip where your speedometer showed exactly 25 km/h. This point c is what MVT guarantees.

Mathematical Representation

Let’s express this example mathematically:

• If (f(x)) is the distance traveled at time (x), then (f(4)=100) (100 km at 6 PM).
• The slope of the secant line from (f(2)) to (f(6)) gives the average speed:
[ \frac{f(6) - f(2)}{6 - 2} = \frac{100 - 0}{4} = 25 \text{ km/h} ]
• By MVT, there exists (c) such that:
[ f’© = 25 ]

⚠️ Note: Remember, the point c is not unique. There could be more than one point where the instantaneous rate equals the average rate over the interval.

Application of the Mean Value Theorem

The MVT has numerous practical applications:

• Physics: It helps in understanding velocity and acceleration, particularly when analyzing motion with varying speeds.
• Economics: It can be used to understand the rate of change of revenue, costs, and profits over specific periods.
• Engineering: For optimizing paths or trajectories to ensure a uniform rate of change in a system.

Example of Application

Consider an economist studying the change in revenue over a quarter. If revenue function (R(t)) where (t) is time in months is continuous and differentiable:

Month

Revenue ()</th> </tr> <tr> <td>0 (Start of Quarter)</td> <td>10000</td> </tr> <tr> <td>3 (End of Quarter)</td> <td>16000</td> </tr> </table> <p>The average rate of change in revenue is:</p> \[ \frac{16000 - 10000}{3 - 0} = 2000 \text{ /month} ] By MVT, there exists some (c) where the instantaneous rate of change equals this average rate, indicating at least one month where the business experienced this particular growth rate. 💡 Note: MVT allows us to infer about the internal behavior of functions based on their boundary conditions. Proof of the Mean Value Theorem While the proof of MVT involves some intricate calculus, here’s a simplified outline: Apply Rolle’s Theorem to a specially constructed function. Construct a new function (F(x) = f(x) - g(x)), where (g(x)) is a straight line joining (f(a)) to (f(b)). If (F(a) = F(b) = 0), by Rolle’s theorem, there exists (c) in ((a, b)) such that (F’© = 0). Since (F(x) = f(x) - \left( f(a) + \frac{f(b) - f(a)}{b - a} (x - a) \right)), (F’© = 0) implies: [ f’© = \frac{f(b) - f(a)}{b - a} ] 📝 Note: This proof leverages the understanding that for a continuous and differentiable function, there's a local maximum or minimum where the derivative is zero, which matches our theorem’s requirement. Reflecting on the journey from understanding to applying the Mean Value Theorem, it's clear that this theorem not only simplifies our approach to function analysis but also bridges the gap between average and instantaneous rates of change. By using MVT, we unlock insights into the dynamics of how functions change over intervals, making it an invaluable tool for students and professionals alike in fields where calculus plays a crucial role. Whether you're studying velocity, cost functions, or optimizing trajectories, MVT provides the theoretical backbone to assert with confidence the existence of specific conditions within an interval. Can the Mean Value Theorem apply to non-continuous functions? + No, the Mean Value Theorem only applies to functions that are continuous on a closed interval and differentiable on the corresponding open interval. Why is the Mean Value Theorem important in calculus? + The MVT is fundamental because it provides a link between the derivative of a function (how it changes instantaneously) and the function itself (how it changes overall in an interval). This relationship is crucial for understanding and proving many other theorems and applications in calculus. Is there always one and only one point (c) where the Mean Value Theorem applies? + Not necessarily. There might be more than one point where the function’s derivative equals the average rate of change over the interval.