5 Math Properties Every Student Must Know

The world of mathematics is full of patterns, structures, and rules that help us understand the universe and solve practical problems. Whether you're diving into basic arithmetic, tackling algebra, or exploring complex theories in calculus, there are foundational properties you need to grasp. Here are five key math properties that every student must know:
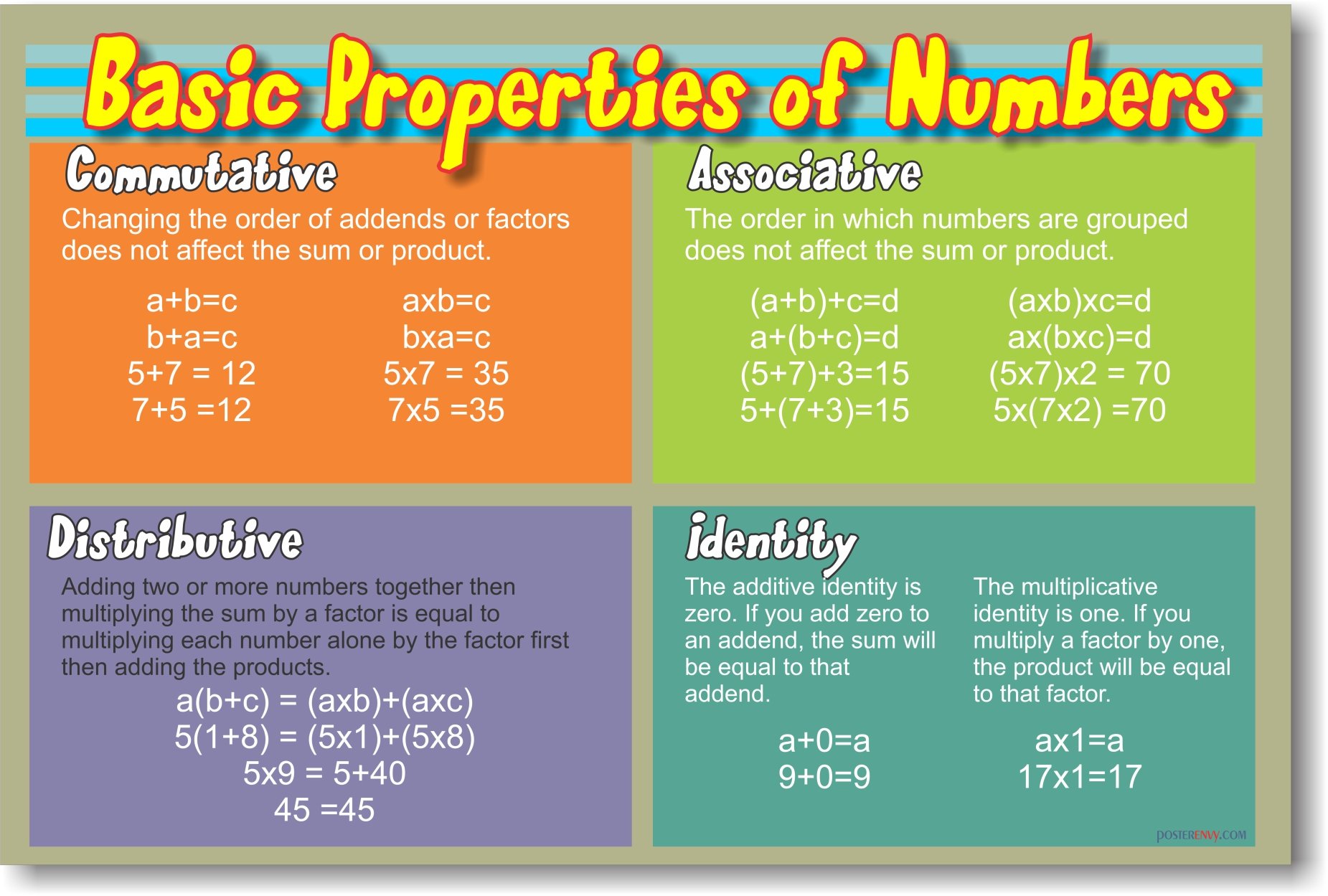
1. Commutative Property
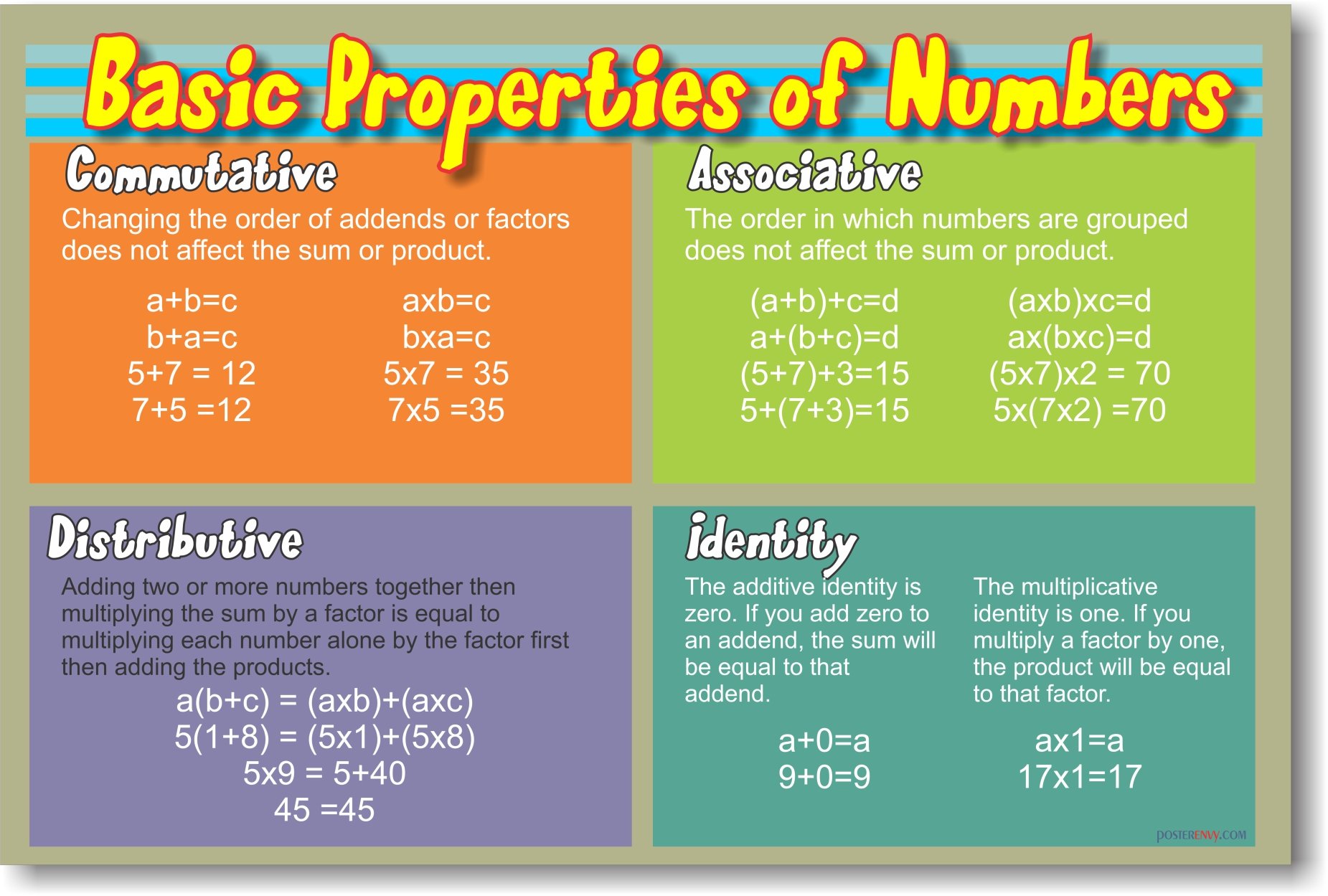
This property states that the order of numbers in an operation does not change the result, at least for addition and multiplication:
- Addition: a + b = b + a
- Multiplication: a x b = b x a
This property does not apply to subtraction or division, making it specific to addition and multiplication only.

2. Associative Property

The associative property allows regrouping of the numbers in an operation:
- Addition: (a + b) + c = a + (b + c)
- Multiplication: (a x b) x c = a x (b x c)
Like the commutative property, this one also does not apply to subtraction or division.
3. Distributive Property

This property links addition and multiplication, allowing us to distribute a multiplication across terms inside parentheses:
- Distribution over Addition: a x (b + c) = a x b + a x c
- Distribution over Subtraction: a x (b - c) = a x b - a x c
The distributive property is essential in solving equations, algebraic manipulation, and simplifying complex expressions.

4. Identity Property

The identity property describes elements in mathematics that, when used in an operation, result in the same value as the operand:
- Addition Identity: a + 0 = a (0 is the additive identity)
- Multiplication Identity: a x 1 = a (1 is the multiplicative identity)
Knowing the identity elements helps in solving equations, checking for errors, and understanding how numbers interact.
5. Inverse Property

The inverse property states that each number has an inverse that, when combined in an operation, results in the identity element:
- Additive Inverse: For any a, there exists an inverse -a such that a + (-a) = 0
- Multiplicative Inverse: For any a (excluding 0), there exists an inverse 1/a such that a x (1/a) = 1
Inverses are crucial in operations like division and are key in solving equations and simplifying expressions.
⚠️ Note: While these properties seem simple, their applications can be complex. Understanding when and how to use them can make solving problems in mathematics much more straightforward.
Understanding these fundamental properties provides a solid foundation for mathematical reasoning. They help in simplifying calculations, solving equations, and understanding the relationships between numbers and operations. These properties are not just isolated facts but are interconnected, providing a framework for all mathematical operations and manipulations. By mastering these properties, students can enhance their problem-solving skills, making complex math problems manageable and fostering a deeper understanding of mathematical concepts.
Why don’t subtraction and division have the commutative property?
+
Subtraction and division are not commutative because the order of the numbers matters. For example, 3 - 2 ≠ 2 - 3 and 6 ÷ 3 ≠ 3 ÷ 6. The structure of these operations does not allow for rearranging the numbers without changing the result.
How do these properties help in real-world applications?

+
Properties like the commutative, associative, and distributive ones are used in everyday calculations, budgeting, accounting, engineering, and science for quick and reliable computations. They simplify complex tasks by allowing you to group or order operations in a way that suits the context of the problem.
Can these properties help in simplifying algebraic expressions?

+
Absolutely! Algebra heavily relies on these properties. For instance, the distributive property is key in multiplying polynomials, while the associative property helps in grouping terms for easier simplification. Knowing when and how to use these properties can turn a complex expression into something much simpler to solve.



