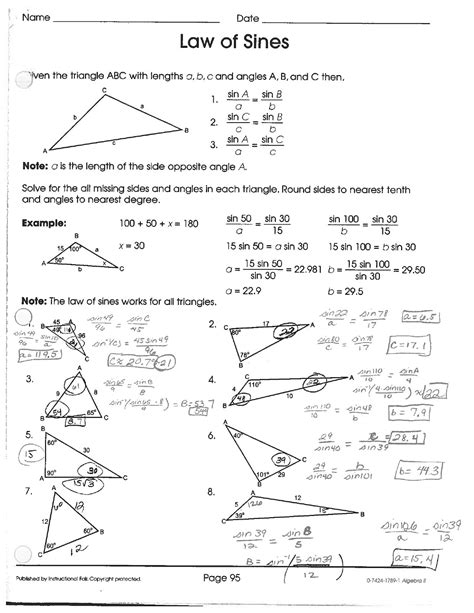
Mastering the Law of Sines and Cosines: Worksheet Guide

As a student diving into trigonometry, understanding the Law of Sines and Cosines can be a bit challenging. These fundamental concepts are essential for solving triangles and calculating distances in various fields like physics, engineering, and even navigation. This guide aims to simplify these laws through practical examples and exercises, offering you a robust foundation to tackle more advanced trigonometry problems.
Understanding the Law of Sines

The Law of Sines states that the ratio of the length of a side of a triangle to the sine of the opposite angle is the same for all three sides of the triangle. This can be expressed as:
- a/sin A = b/sin B = c/sin C
Here:
- a, b, and c are the lengths of the sides opposite to angles A, B, and C, respectively.
- Sin stands for sine, one of the fundamental trigonometric functions.
Consider a triangle where you know one side and two angles (AAS or ASA). The Law of Sines can help find the remaining side and angle:
| Given | To Find |
|---|---|
| Two angles (A, B) and one side (a) | Side c |
🌟 Note: Always verify if the data provided forms a valid triangle before using the Law of Sines or Cosines.
Applying the Law of Sines

Let’s solve for side b in a triangle where side a = 8 cm, angle A = 40°, and angle B = 60°:
- Step 1: First, find angle C:
- C = 180° - (A + B)
- C = 180° - (40° + 60°) = 80°
- Step 2: Use the Law of Sines:
- b/sin 60° = a/sin 40°
- b = (8 cm / sin 40°) * sin 60°
- b ≈ 10.2 cm
The Law of Cosines

When you have sides of a triangle or need to solve when you have an angle and the length of two sides, the Law of Cosines comes into play. The formula is:
- c² = a² + b² - 2ab cos C
- a² = b² + c² - 2bc cos A
- b² = a² + c² - 2ac cos B
These can be used in different scenarios, such as:
| Scenario | Application |
|---|---|
| Knowing all sides, find an angle | Use the formula where you can isolate cos |
| Knowing one angle and two sides, find the third side | Apply the corresponding formula directly |
Worksheet Example: Solving with the Law of Cosines

Let’s find the length of side c in a triangle where a = 5 cm, b = 7 cm, and angle C = 50°:
- Step 1: Use the Law of Cosines:
- c² = 5² + 7² - (2 * 5 * 7 * cos 50°)
- c² = 25 + 49 - (70 * 0.6428) [cos 50° = 0.6428]
- c² = 25 + 49 - 44.996
- c² ≈ 29.004
- c ≈ √29.004 ≈ 5.4 cm
📚 Note: Keep your calculator set to degree mode when solving triangle problems with angle measurements in degrees.
Combining Both Laws for Comprehensive Problem Solving

Some problems might require the use of both the Law of Sines and the Law of Cosines. Here’s how you can approach a mixed problem:
- Problem: In triangle ABC, a = 12 cm, b = 9 cm, and angle A = 45°. Find angle C.
- Solution:
- Use the Law of Cosines to find side c:
- c² = 9² + 12² - (2 * 9 * 12 * cos 45°)
- c² = 81 + 144 - (216 * 0.7071)
- c² = 225 - 153.148
- c² ≈ 71.852
- c ≈ 8.5 cm
- Then, use the Law of Sines to find angle C:
- sin C / 8.5 = sin 45° / 12
- sin C = (sin 45° / 12) * 8.5
- sin C ≈ 0.4988
- C = arcsin(0.4988) ≈ 30.6°
- Use the Law of Cosines to find side c:
Practical Applications and Real-World Scenarios

Trigonometry, particularly the Laws of Sines and Cosines, isn’t just a classroom exercise:
- Navigation: Determining the distance between two known points or finding the altitude of an aircraft.
- Engineering: Calculating the forces on structures or the length of supports in design and construction.
- Surveying: Mapping out property lines or terrain features where direct measurement isn't possible.
To wrap up our exploration, mastering the Law of Sines and Cosines enhances your problem-solving skills in trigonometry. These laws provide versatile tools for working with triangles in different configurations, whether you're solving for sides, angles, or understanding how elements of a triangle relate to one another. By practicing these laws through worksheets and real-world applications, you not only sharpen your mathematical skills but also prepare yourself for real-life challenges in various technical fields.
What’s the difference between the Law of Sines and Cosines?

+
The Law of Sines relates the sides of a triangle to the sine of their opposite angles, useful when you know two angles and one side (AAS or ASA). The Law of Cosines, on the other hand, helps you find sides or angles in triangles where you have three sides or two sides and an included angle (SSS, SAS).
Can I use the Law of Sines if I know all three sides?

+
No, if you know all three sides, you’ll need the Law of Cosines to find angles. However, if you find one angle with the Law of Cosines, you can then use the Law of Sines for the other angles.
When is it better to use the Law of Cosines instead of the Law of Sines?

+
When you’re given the sides of a triangle (SSS) or two sides and the included angle (SAS), the Law of Cosines is more efficient for finding angles or the third side directly. The Law of Sines requires at least one angle to start calculations.