Kepler's Laws Worksheet: Quick Planetary Motion Answers

Kepler's laws of planetary motion are the cornerstones of modern astrophysics, providing a framework for understanding how planets and other celestial bodies orbit around the sun. These laws were developed by Johannes Kepler based on meticulous observations of planetary positions, especially those of Mars, made by his predecessor, Tycho Brahe. In this comprehensive worksheet, we'll delve into each law, providing quick answers to commonly asked questions, explanations of their significance, and how they've shaped our understanding of the solar system.
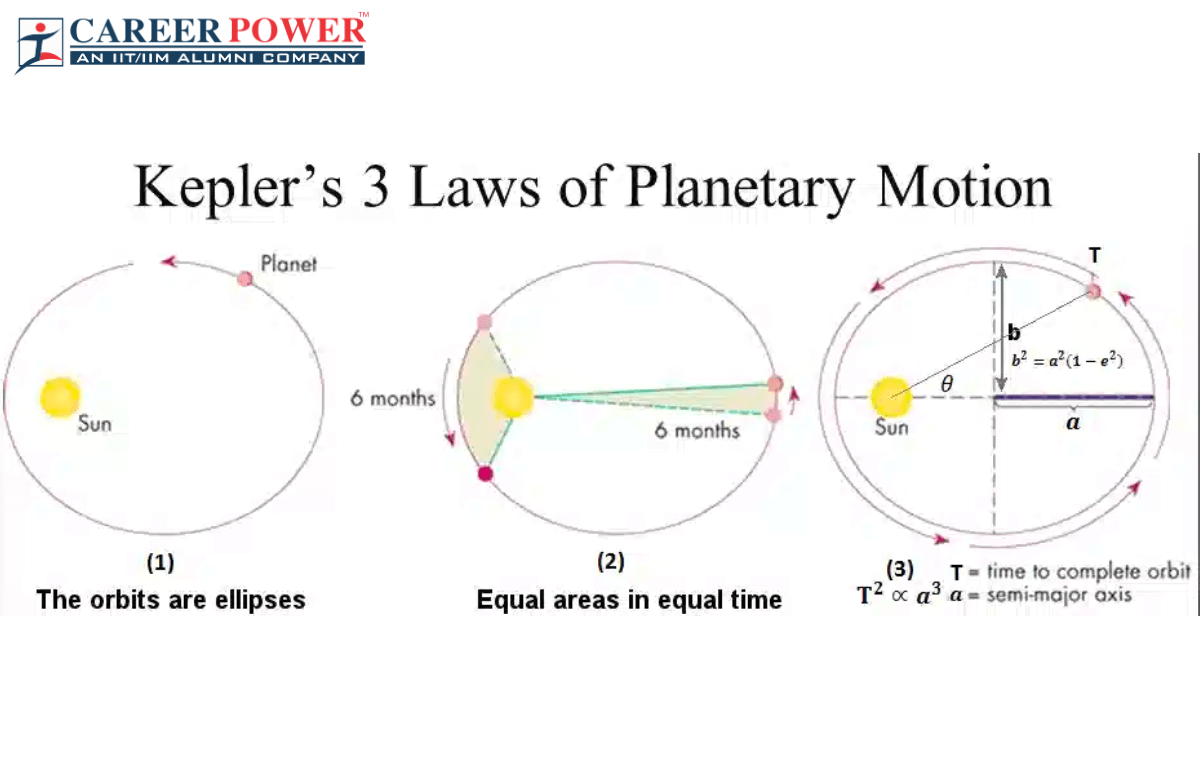
Kepler's First Law: The Law of Orbits

Kepler's first law, also known as the law of ellipses, states that:
- All planets move in elliptical orbits, with the sun at one of the two foci of the ellipse.
How Does It Affect Planetary Motion?

The elliptical orbit ensures that the distance of a planet from the sun varies, leading to:
- Changes in orbital speed due to the conservation of angular momentum.
- Variations in the amount of solar energy received by the planet, which influences climate.
🌍 Note: This law refuted the ancient belief in perfect circular orbits and introduced a more accurate model of planetary motion.

Kepler's Second Law: The Law of Areas

This law, often called the law of equal areas, states that:
- A line joining a planet and the sun sweeps out equal areas in equal intervals of time.
Why is this Law Significant?

- It explains the variation in a planet's speed as it orbits the sun:
- The planet moves faster when closer to the sun (perihelion) and slower when farther away (aphelion).
🕰️ Note: This law is a direct consequence of the conservation of angular momentum in orbital dynamics.
Kepler's Third Law: The Law of Periods

Also referred to as the law of harmonies, it states that:
- The square of the orbital period of a planet is directly proportional to the cube of the semi-major axis of its orbit.
Mathematical Expression

Here's the mathematical relationship:
T² = R³, where T is the orbital period and R is the semi-major axis of the orbit, measured in astronomical units (AU).
| Planet | Semi-Major Axis (AU) | Orbital Period (Earth Years) |
|---|---|---|
| Mercury | 0.39 | 0.24 |
| Venus | 0.72 | 0.62 |
| Earth | 1 | 1 |
| Mars | 1.52 | 1.88 |

Impact on Astronomy

- Helped in discovering new planets and celestial bodies by predicting their orbital periods based on observed distances.
- Kepler's work set the stage for Newton's law of universal gravitation.
📚 Note: Kepler's laws were not immediately accepted by all astronomers; they needed to be proven by empirical observations and later by Newtonian mechanics.
Applications in Modern Astronomy

Kepler's laws have applications far beyond the historical context:
- Orbital Mechanics: Essential for satellite technology, space missions, and understanding exoplanets.
- Astrodynamics: Helps predict the path of objects like comets, asteroids, and spacecraft.
- Planetary Habitability: Orbital parameters determine climate conditions, affecting potential habitability.
Final Thoughts

In wrapping up our exploration of Kepler's laws, we've seen how these principles have revolutionized our understanding of the cosmos. From the elliptical paths of planets to the regularity of their motions, Kepler's work has not only explained the mechanics of our solar system but also provided a foundation for further astronomical discoveries. The laws continue to be relevant in modern astronomy, helping us navigate space, understand other star systems, and push the boundaries of what we know about the universe.
What is the difference between perihelion and aphelion?
+
Perihelion is the point in a planet’s orbit where it is closest to the sun, while aphelion is the furthest point from the sun. A planet travels faster at perihelion due to Kepler’s second law.
Can Kepler’s laws be applied to moons or satellites?

+
Yes, Kepler’s laws apply to any two objects orbiting each other under gravitational influence, including moons and man-made satellites.
How do Kepler’s laws relate to Newton’s laws of motion?

+
Kepler’s laws describe the observed patterns of planetary motion, while Newton’s laws provide the physical explanation for these patterns through the law of universal gravitation and laws of motion.