Unlock Inverse Functions: Your Essential Worksheet Guide

Understanding inverse functions can be a pivotal step in mastering mathematical concepts, particularly in algebra and calculus. These functions not only allow us to reverse operations but also unlock doors to understanding deeper mathematical theories like symmetry, transformations, and more complex function analysis. This guide aims to demystify inverse functions, offering a comprehensive worksheet that covers everything from definitions to solving practical problems.
What Are Inverse Functions?

An inverse function undoes the operation of another function. If f(x) maps x to y , its inverse f^{-1}(y) maps y back to x . Here's a simple way to think about it:
- If f(a) = b , then f^{-1}(b) = a .
- The functions f and f^{-1} are mirrors of each other, reflecting across the line y = x .
Properties of Inverse Functions

- Existence: Not all functions have inverses, especially if they are not one-to-one. A function must pass the horizontal line test to have an inverse.
- One-to-One: A function must be one-to-one, or injective, to have a unique inverse.
- Domain and Range: The domain of the original function becomes the range of its inverse, and vice versa.
- Composition: If f(x) has an inverse, then f(f^{-1}(x)) = x and f^{-1}(f(x)) = x .

📚 Note: Functions like quadratic equations, where multiple x 's can map to the same y , generally do not have inverses unless restricted to a specific interval.
Finding the Inverse Function

The process of finding an inverse function involves these steps:
- Replace f(x) with y .
- Switch the roles of x and y .
- Solve for y to express x in terms of y .
- Replace y with f^{-1}(x) .
Examples

Example 1: Linear Function
Let's find the inverse of f(x) = 3x + 2 .
- Set y = 3x + 2 .
- Switch x and y : x = 3y + 2 .
- Solve for y :
- x - 2 = 3y
- y = \frac{x - 2}{3}
- Therefore, f^{-1}(x) = \frac{x - 2}{3} .
🔍 Note: Here, we've ensured that every step is reversible, confirming the existence of an inverse.
Example 2: Exponential Function
Let's find the inverse of g(x) = 5^x .
- Set y = 5^x .
- Switch x and y : x = 5^y .
- Use logarithms to solve for y :
- \log_5(x) = y
- Thus, g^{-1}(x) = \log_5(x) .
Worksheet: Inverse Function Practice

This worksheet provides step-by-step exercises to practice finding and verifying inverse functions:
Exercise 1: Linear Inverse
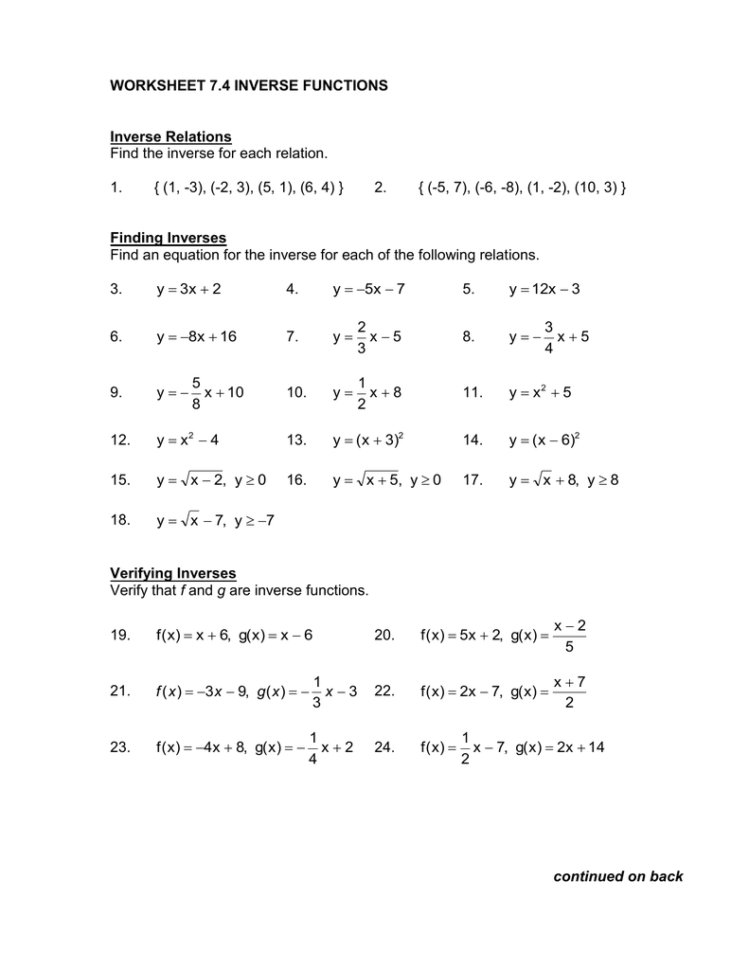
Find the inverse of the function h(x) = \frac{x - 1}{2} .
💡 Note: Remember to check if the function is one-to-one before proceeding.
Exercise 2: Quadratic Function

Find the inverse of k(x) = x^2 for x \geq 0 .
🔎 Note: Restricting the domain is necessary for quadratic functions to ensure a one-to-one relationship.
Exercise 3: Logarithmic Inverse

Find the inverse of l(x) = \log_3(2x) .
| Original Function | Inverse Function | Verification |
|---|---|---|
| h(x) = \frac{x - 1}{2} | h^{-1}(x) = 2x + 1 | h(h^{-1}(x)) = h(2x + 1) = \frac{(2x + 1) - 1}{2} = x |
| k(x) = x^2 | k^{-1}(x) = \sqrt{x} | k(k^{-1}(x)) = k(\sqrt{x}) = (\sqrt{x})^2 = x |
| l(x) = \log_3(2x) | l^{-1}(x) = \frac{3^x}{2} | l(l^{-1}(x)) = \log_3(\frac{3^x}{2} \times 2) = \log_3(3^x) = x |

📘 Note: Make sure to verify that both compositions yield the original argument for all functions.
Real-world Applications

Inverse functions have numerous applications:
- Decoding: Used in cryptography to reverse encryption.
- Physics and Engineering: Analyzing the effects of certain operations or transforming variables.
- Biometrics: In fingerprint matching, where an image is transformed back into a coordinate system.
Through this exploration of inverse functions, we've demystified their role in mathematical operations. From theoretical properties to practical exercises, we've equipped readers with the tools to not only understand but apply these concepts in various contexts. The journey of uncovering inverse functions helps us appreciate the structure and symmetry inherent in mathematics.
What if a function doesn’t have an inverse?

+
Not all functions have inverses, particularly if they fail the horizontal line test. Functions that are not one-to-one need to be restricted to specific domains to ensure an inverse exists.
How do you verify an inverse function?

+
You can verify an inverse by checking the composition of the function with its supposed inverse. If ( f(f^{-1}(x)) = x ) and ( f^{-1}(f(x)) = x ), then the inverse is verified.
Can a function have more than one inverse?

+
No, a function can only have one unique inverse. If multiple inverses exist, it would violate the definition of a function, as each element in the domain must map to exactly one element in the codomain.