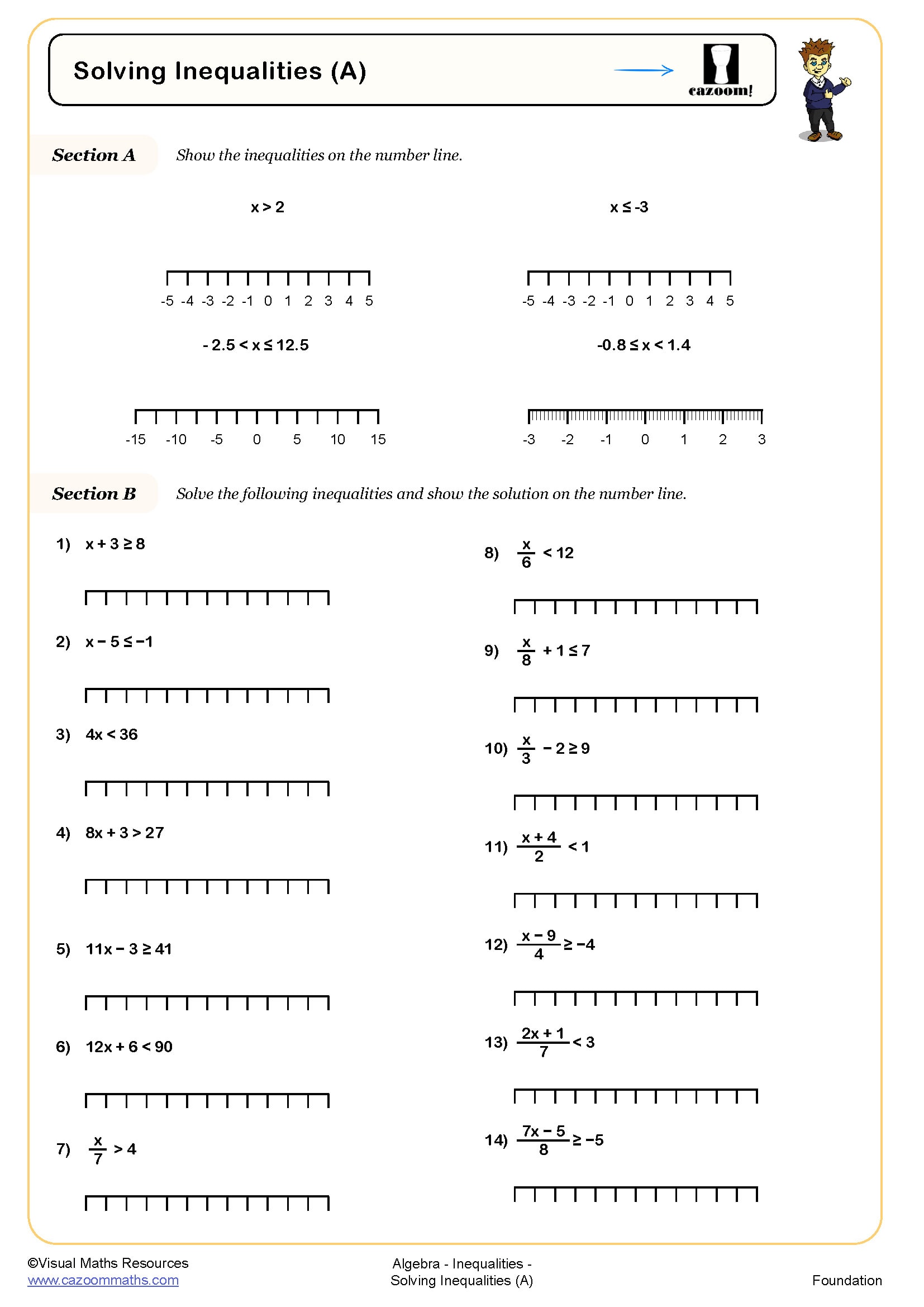
5 Effective Strategies for Mastering Inequalities Worksheets

If you're learning math, one of the essential skills you'll need to master is solving inequalities. These mathematical statements provide an array of solutions rather than a single answer, making them both challenging and rewarding to tackle. Here, we'll explore five effective strategies to help you excel in your inequalities worksheets and pave the way to mathematical success.
Understanding the Basics


Before diving into the nitty-gritty of complex inequalities, it’s crucial to grasp the fundamental concepts:
- Types of Inequalities: Learn about less than (<), greater than (>), less than or equal to (≤), and greater than or equal to (≥).
- Properties: Understand how to manipulate inequalities (addition, subtraction, multiplication by positive/negative numbers, etc.).
- Graphing: Get familiar with plotting inequalities on a number line or coordinate plane.
Strategy 1: Identify the Inequality Type

Every inequality comes with its own set of rules:
- Linear Inequalities: Solve using standard algebraic methods and remember to reverse the inequality sign if you multiply or divide by a negative number.
- Quadratic Inequalities: Factor the quadratic equation, solve the related equation, and then test points to determine where the inequality holds.
- Compound Inequalities: Break into separate inequalities, solve each one, and find the intersection or union of solutions as needed.
Strategy 2: Use Test Points

Testing points within intervals can be an incredibly effective technique:
- After solving an inequality, divide the number line into intervals using the critical points (where the inequality equals zero).
- Choose a test point in each interval, substitute it into the inequality, and if the result is true for that interval, then all points in that interval are solutions.
📌 Note: Remember to test boundary points if the inequality involves ‘≤’ or ‘≥’.
Strategy 3: Graphical Solutions


Graphical methods can provide an intuitive way to visualize solutions, particularly for linear inequalities in two variables:
- Convert the inequality to an equation, graph the line or parabola, and then determine which side of the line or curve fulfills the inequality condition.
- For linear inequalities, use the sign to decide whether to shade above or below the line; for strict inequalities, use a dashed line.
Strategy 4: Rearrange and Solve Methodically

When solving inequalities, follow a systematic approach:
- Isolate the variable on one side of the inequality.
- Follow the order of operations when simplifying.
- Be vigilant about reversing the inequality sign when multiplying or dividing by a negative number.
🛑 Note: Sometimes, simplifying an inequality can reveal that all real numbers or no real numbers are solutions.
Strategy 5: Practice, Practice, Practice


Nothing beats regular practice when it comes to mastering inequalities:
- Work through a variety of inequalities worksheets with increasing difficulty.
- Use online tools or apps for extra practice.
- Join a study group or seek help from a tutor if you find certain types of inequalities particularly challenging.
By applying these strategies, you'll find that inequalities become more manageable. Remember that each type of inequality has its own nuances, so mastering these approaches can help you solve problems confidently and accurately. Through consistent practice and understanding, you'll be equipped not only to excel in your inequalities worksheets but also to apply these skills in various real-world scenarios.
What are common mistakes when solving inequalities?
+
Frequent errors include forgetting to reverse the inequality sign when multiplying or dividing by a negative number, misreading the inequality symbol, and not considering all possible solutions when working with compound inequalities.
How can I check if my solution to an inequality is correct?

+
Substitute test points back into the original inequality to verify if they satisfy the condition, or graph the inequality to visually confirm the solution set.
Are there any shortcuts to solving complex inequalities?

+
While shortcuts can vary by problem, understanding and recognizing the structure of inequalities like absolute value or those with multiple terms can streamline the process. Also, memorizing key transformations and their effects on the inequality sign can save time.
How important is the role of graphing in solving inequalities?

+
Graphing is immensely helpful, especially for visualizing the solution set of linear inequalities in two variables. It provides a clear picture of where solutions lie on the coordinate plane, making it easier to understand the problem’s scope.