Mastering Inequalities in Two Triangles Worksheet
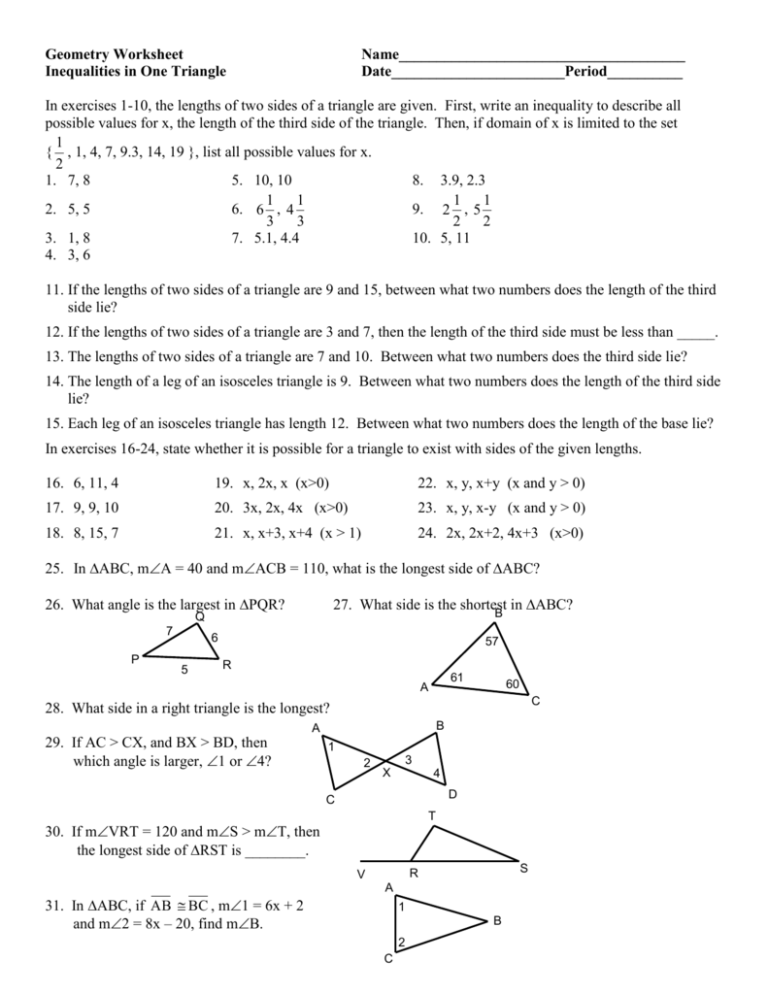
When it comes to mastering the intricacies of mathematics, particularly in geometry, understanding how to handle inequalities in two triangles is fundamental. This skill not only equips students with the capability to solve complex geometric problems but also hones their logical reasoning and analytical thinking. This long-form blog post will delve into the various facets of working with inequalities in two triangles, providing comprehensive insights through structured steps, key notes, and a touch of problem-solving elegance.
Understanding Inequalities in Two Triangles

Before diving into the worksheet, it’s crucial to grasp the basic principles of inequalities in triangles. These principles often involve:
- Triangle Inequality Theorem: The sum of the lengths of any two sides of a triangle must be greater than the length of the remaining side.
- Hinge Theorem (or SAS Inequality Theorem): If two sides of one triangle are congruent to two sides of another triangle, but the included angles are unequal, then the longer third side is opposite the larger included angle.
- External Angle Inequality: The external angle of a triangle is greater than either of the opposite interior angles.
The Worksheet Approach

The following steps outline how to approach and conquer the inequalities in two triangles worksheet:
Step 1: Analyze the Given Information

Always start by carefully reading the information provided in the problem or the diagram:
- Identify which sides are congruent or if any angles are known.
- Determine which theorems or properties you can apply based on the given information.
Step 2: Apply Triangle Inequality Theorem

One of the first tools you’ll use is the Triangle Inequality Theorem:
| Triangle | Side A | Side B | Side C | Inequalities |
|---|---|---|---|---|
| Triangle 1 | a | b | c | a + b > c, a + c > b, b + c > a |
| Triangle 2 | d | e | f | d + e > f, d + f > e, e + f > d |

💡 Note: Be sure to check all possible inequalities because you might need to use all of them to reach a conclusion.
Step 3: Use the Hinge Theorem

When the problem involves comparing two triangles where two sides are equal but the included angles are not:
- Determine which triangle has the larger included angle.
- Use this information to compare the third sides using the Hinge Theorem.
Step 4: External Angle Inequality

If the problem involves external angles, apply the External Angle Inequality:
- An external angle of a triangle is larger than either of its opposite interior angles.
Step 5: Combine the Results

After applying the theorems, combine the results to reach the desired conclusion or inequality:
- Use logical reasoning to connect your findings from different triangles or angles.
- Ensure your conclusion follows directly from the application of these theorems.
🔍 Note: When combining results, it's helpful to sketch or visualize the triangles if you're having trouble following the inequalities.
Concluding Thoughts

In summary, mastering inequalities in two triangles requires a systematic approach. By understanding the foundational theorems like the Triangle Inequality Theorem, Hinge Theorem, and External Angle Inequality, you can navigate through worksheet problems with confidence. The key is to carefully analyze the given information, apply the appropriate theorems, and use logical reasoning to deduce the necessary inequalities. Practice, patience, and a keen eye for detail will turn you into a geometry maestro, capable of dissecting complex problems into understandable parts.
What are the most common mistakes when solving inequalities in triangles?

+
The most common mistakes include overlooking the fact that the sum of any two sides must exceed the third side, misinterpreting the Hinge Theorem, and not recognizing when to use the External Angle Inequality. Regular practice and review can minimize these errors.
How can I check if my inequality solution is correct?

+
You can verify your solution by:
- Reapplying the theorems used to derive the inequality.
- Sketching the triangles and visually confirming the inequality.
- Substituting known values to check if the inequality holds true.
Can I use inequalities to prove other geometric properties?

+
Yes, inequalities in triangles are fundamental in proving various geometric properties like medians, altitudes, and even in the study of trigonometry and complex numbers in geometry.
What advanced topics build on the understanding of triangle inequalities?

+
Advanced topics include:
- Geometric Inequalities like the Cauchy-Schwarz Inequality or the Minkowski Inequality.
- Triangulation methods in geometry and computer science.
- Optimization problems in calculus that involve geometric shapes.