Increasing and Decreasing Percentages Practice Made Easy
Mastering Percentage Calculations: A Step-by-Step Guide

Percentages are a fundamental concept in mathematics, and understanding how to calculate them is crucial for various real-life applications. Whether you’re a student, a professional, or simply someone who wants to improve your math skills, this guide will walk you through the process of increasing and decreasing percentages with ease.
What is a Percentage?

Before we dive into the calculations, let’s quickly review what a percentage is. A percentage is a way to express a value as a fraction of 100. It’s often denoted by the symbol “%” and represents a proportion or a fraction of a whole.
Increasing Percentages

Increasing a percentage means adding a certain percentage of the original value to itself. To calculate an increase in percentage, follow these steps:
- Determine the original value: Identify the original number or value that you want to increase.
- Determine the percentage increase: Identify the percentage by which you want to increase the original value.
- Convert the percentage to a decimal: Divide the percentage by 100 to convert it to a decimal.
- Multiply the original value by the decimal: Multiply the original value by the decimal equivalent of the percentage increase.
- Add the result to the original value: Add the result from step 4 to the original value to get the new value.
Example: Increase 250 by 15%
- Original value: 250
- Percentage increase: 15% = 0.15 (decimal equivalent)
- Multiply original value by decimal: 250 × 0.15 = 37.5
- Add result to original value: 250 + 37.5 = 287.5
Decreasing Percentages

Decreasing a percentage means subtracting a certain percentage of the original value from itself. To calculate a decrease in percentage, follow these steps:
- Determine the original value: Identify the original number or value that you want to decrease.
- Determine the percentage decrease: Identify the percentage by which you want to decrease the original value.
- Convert the percentage to a decimal: Divide the percentage by 100 to convert it to a decimal.
- Multiply the original value by the decimal: Multiply the original value by the decimal equivalent of the percentage decrease.
- Subtract the result from the original value: Subtract the result from step 4 from the original value to get the new value.
Example: Decrease 300 by 20%
- Original value: 300
- Percentage decrease: 20% = 0.20 (decimal equivalent)
- Multiply original value by decimal: 300 × 0.20 = 60
- Subtract result from original value: 300 - 60 = 240
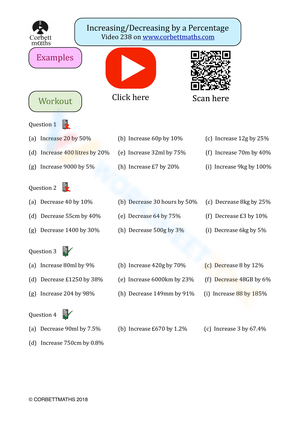
Practice Exercises

Now that you’ve learned the steps to increase and decrease percentages, practice with the following exercises:
Increasing Percentages:
- Increase 120 by 10%
- Increase 500 by 25%
- Increase 180 by 12%
Decreasing Percentages:
- Decrease 400 by 15%
- Decrease 250 by 8%
- Decrease 600 by 22%
Common Applications of Percentage Calculations

Percentage calculations have numerous real-life applications, including:
- Sales and discounts
- Interest rates and investments
- Statistics and data analysis
- Medical and scientific research
Conclusion

Mastering percentage calculations takes practice, but with this step-by-step guide, you’ll be well on your way to becoming a pro. Remember to practice regularly and apply your knowledge to real-life scenarios to reinforce your understanding.
Key Takeaways:
- Increasing percentages involves adding a certain percentage of the original value to itself.
- Decreasing percentages involves subtracting a certain percentage of the original value from itself.
- Convert percentages to decimals by dividing by 100.
- Practice regularly to become proficient in percentage calculations.
What is the difference between increasing and decreasing percentages?

+
Increasing percentages involves adding a certain percentage of the original value to itself, while decreasing percentages involves subtracting a certain percentage of the original value from itself.
How do I convert a percentage to a decimal?

+
To convert a percentage to a decimal, divide the percentage by 100.
What are some common applications of percentage calculations?

+
Percentage calculations have numerous real-life applications, including sales and discounts, interest rates and investments, statistics and data analysis, and medical and scientific research.
Related Terms:
- skills you need percentage calculator
- percentage increase decrease worksheet pdf
- percentage change worksheet year 9
- corbett maths % increase decrease
- percentage increase worksheet grade 7
- free decrease percentage problems worksheet