5 Ways to Master Improper Fractions with Worksheets
Unlocking the Secrets of Improper Fractions

Improper fractions can be a daunting concept for many students, but with the right approach and practice, mastering them can become a breeze. In this article, we will explore five ways to master improper fractions using worksheets, along with some valuable tips and tricks to help you along the way.
Understanding Improper Fractions

Before we dive into the ways to master improper fractions, let’s take a quick look at what they are. An improper fraction is a fraction where the numerator is greater than or equal to the denominator. For example, 3⁄2, 5⁄3, and 7⁄4 are all improper fractions. Improper fractions can be converted to mixed numbers, which consist of a whole number and a proper fraction.
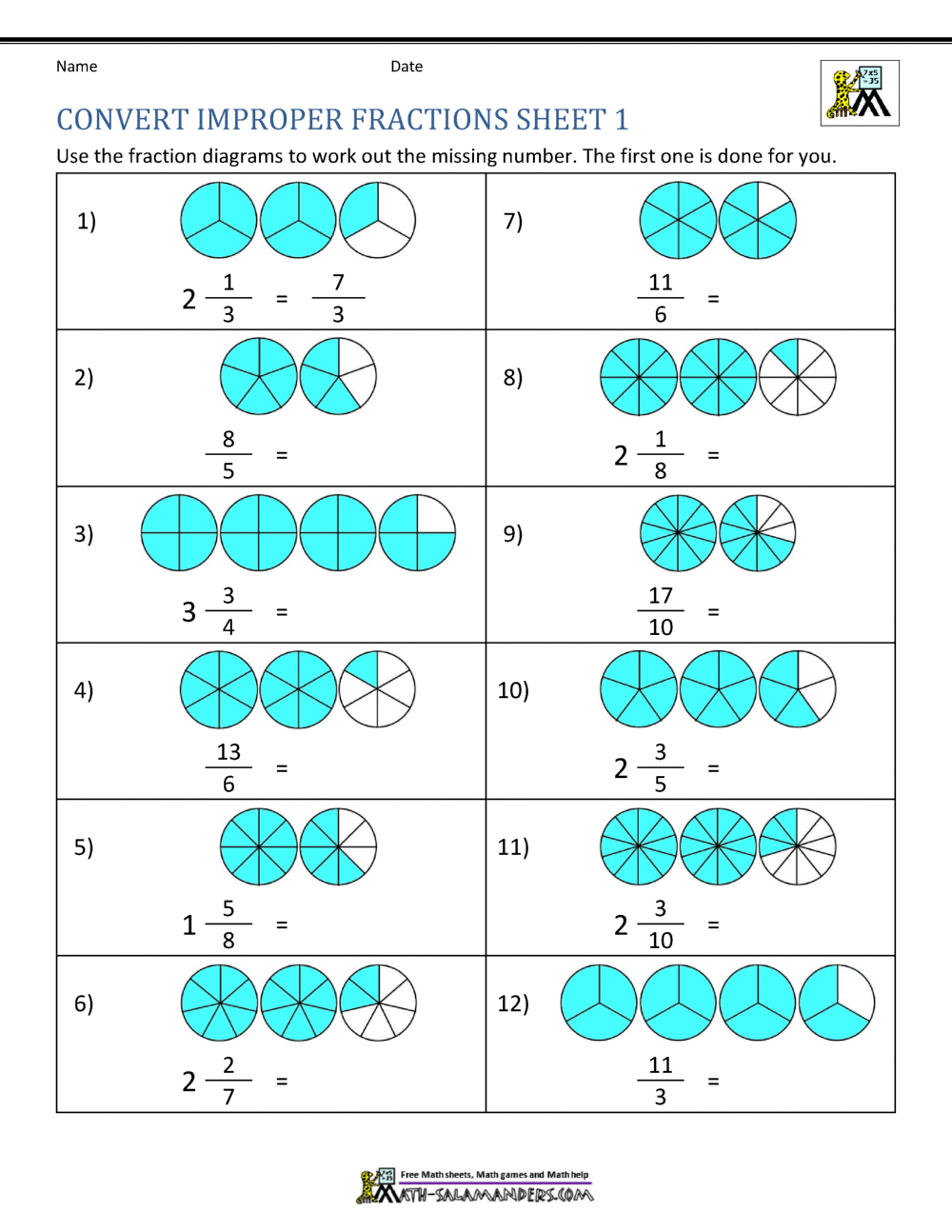
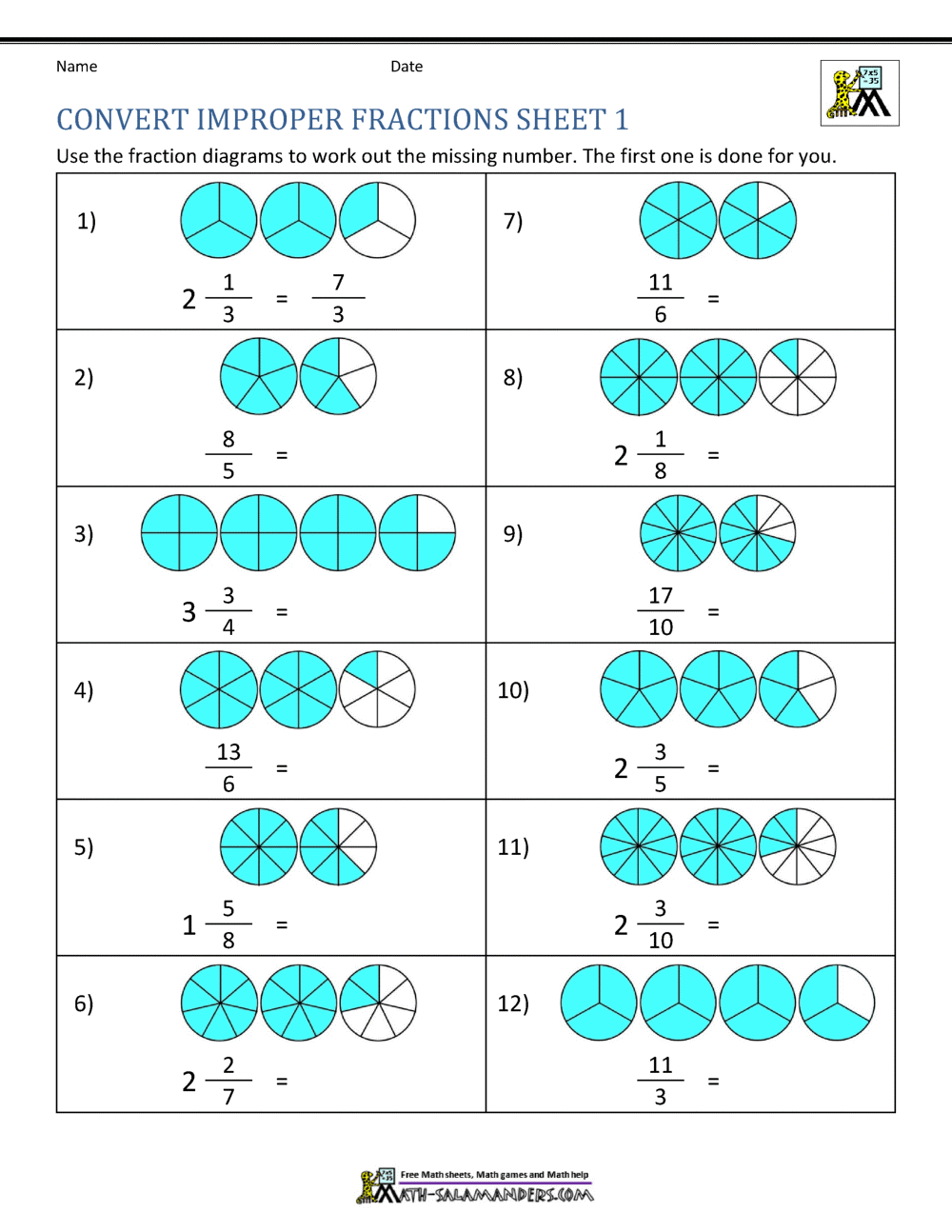
Way 1: Visualizing Improper Fractions

One of the best ways to understand improper fractions is to visualize them. You can use worksheets with diagrams to represent improper fractions. For example, you can draw a circle and divide it into equal parts to represent the denominator. Then, shade the parts that correspond to the numerator.
| Improper Fraction | Diagram |
|---|---|
| 3/2 |  |
| 5/3 |  |
| 7/4 |  |

Way 2: Converting Improper Fractions to Mixed Numbers
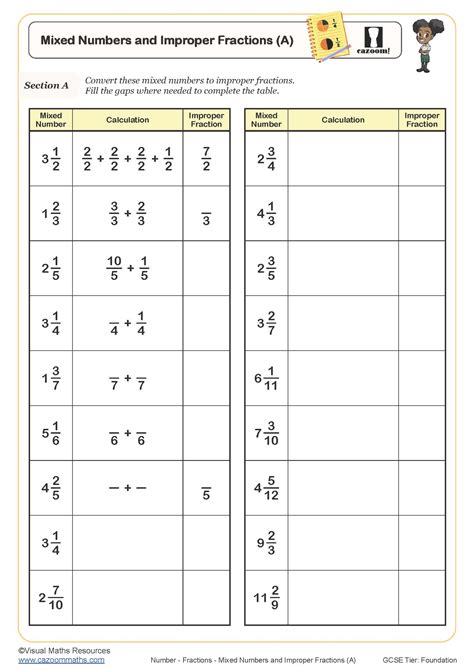
Converting improper fractions to mixed numbers is an essential skill to master. You can use worksheets with exercises that require you to convert improper fractions to mixed numbers. For example:
- 3⁄2 =?
- 5⁄3 =?
- 7⁄4 =?
Answers:
- 3⁄2 = 1 1⁄2
- 5⁄3 = 1 2⁄3
- 7⁄4 = 1 3⁄4
Way 3: Simplifying Improper Fractions
Simplifying improper fractions is another important skill to master. You can use worksheets with exercises that require you to simplify improper fractions. For example:
- 6⁄4 =?
- 9⁄6 =?
- 12⁄8 =?
Answers:
- 6⁄4 = 3⁄2
- 9⁄6 = 3⁄2
- 12⁄8 = 3⁄2
Way 4: Adding and Subtracting Improper Fractions

Adding and subtracting improper fractions can be a bit tricky, but with practice, you can master it. You can use worksheets with exercises that require you to add and subtract improper fractions. For example:
- 3⁄2 + 2⁄3 =?
- 5⁄3 - 1⁄2 =?
Answers:
- 3⁄2 + 2⁄3 = 13⁄6
- 5⁄3 - 1⁄2 = 7⁄6
Way 5: Real-World Applications
Improper fractions have many real-world applications, such as measuring ingredients for cooking, calculating distances, and determining quantities. You can use worksheets with real-world scenarios to practice applying improper fractions. For example:
- A recipe calls for 3⁄4 cup of flour, but you only have a 1⁄2 cup measuring cup. How many times will you need to fill the measuring cup?
- A bookshelf has 5 shelves, and each shelf can hold 3⁄4 of a foot of books. If you have 2 1⁄2 feet of books, how many shelves will you need?
Answers:
- You will need to fill the measuring cup 3 times.
- You will need 3 shelves.
📝 Note: Remember to always simplify your answers and check your work!
In conclusion, mastering improper fractions requires practice, patience, and persistence. By using worksheets and practicing with real-world scenarios, you can become proficient in converting, simplifying, adding, and subtracting improper fractions.
What is an improper fraction?
+
An improper fraction is a fraction where the numerator is greater than or equal to the denominator.
How do I convert an improper fraction to a mixed number?

+
To convert an improper fraction to a mixed number, divide the numerator by the denominator and write the remainder as a fraction.
What is the difference between a proper fraction and an improper fraction?

+
A proper fraction has a numerator that is less than the denominator, while an improper fraction has a numerator that is greater than or equal to the denominator.
Related Terms:
- Improper fraction worksheets pdf
- Improper fraction worksheets with answers
- Improper fraction worksheets grade 4



