7 Ways to Identify Real Numbers on Worksheet
In the dynamic world of mathematics, there exists a fascinating group of numbers known as real numbers. These numbers play a pivotal role in various mathematical contexts, from basic arithmetic to advanced calculus. For students, educators, and anyone keen on mathematics, understanding how to distinguish real numbers on a worksheet is fundamental. Here, we explore seven practical ways to identify and work with real numbers.
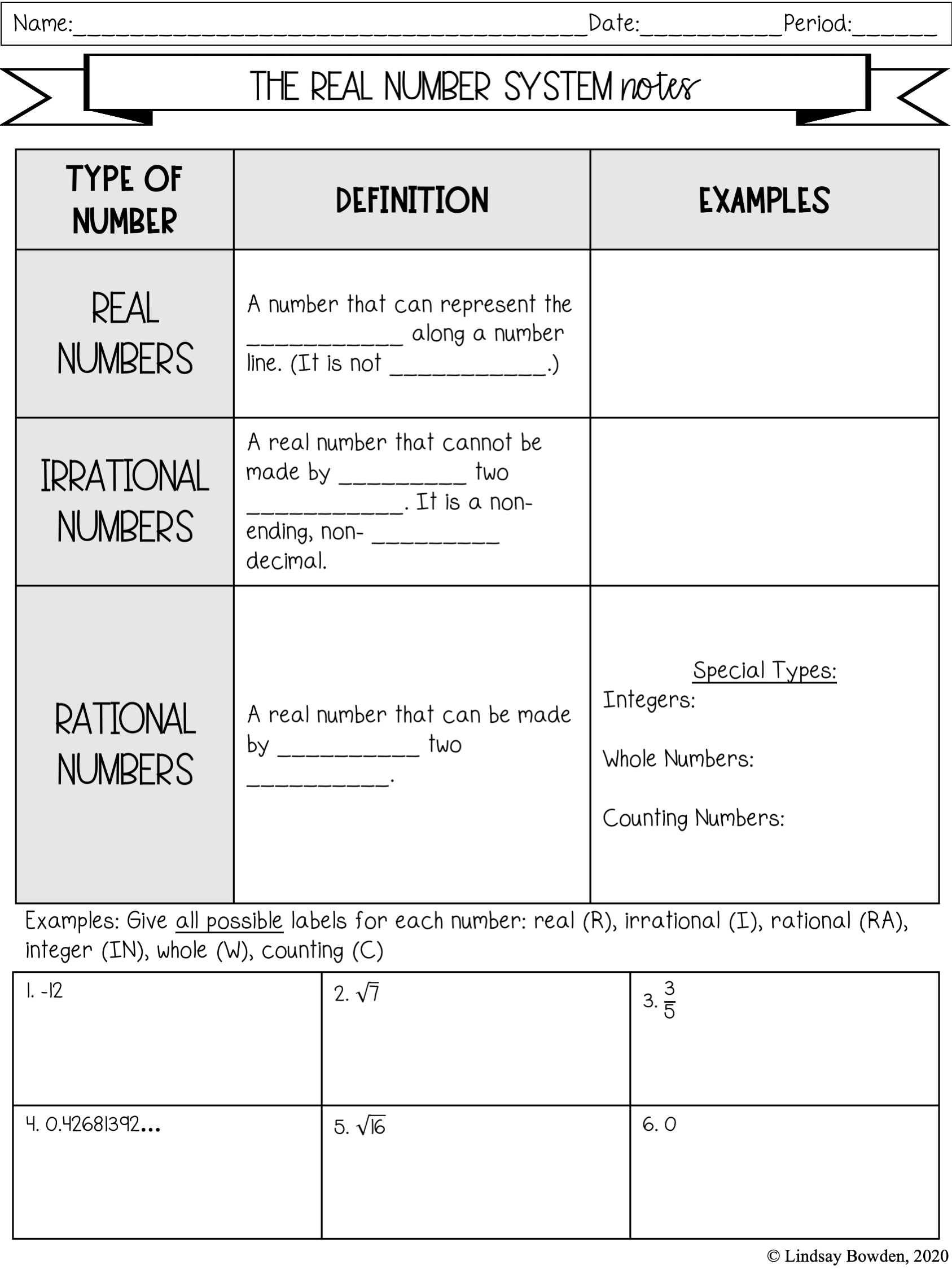
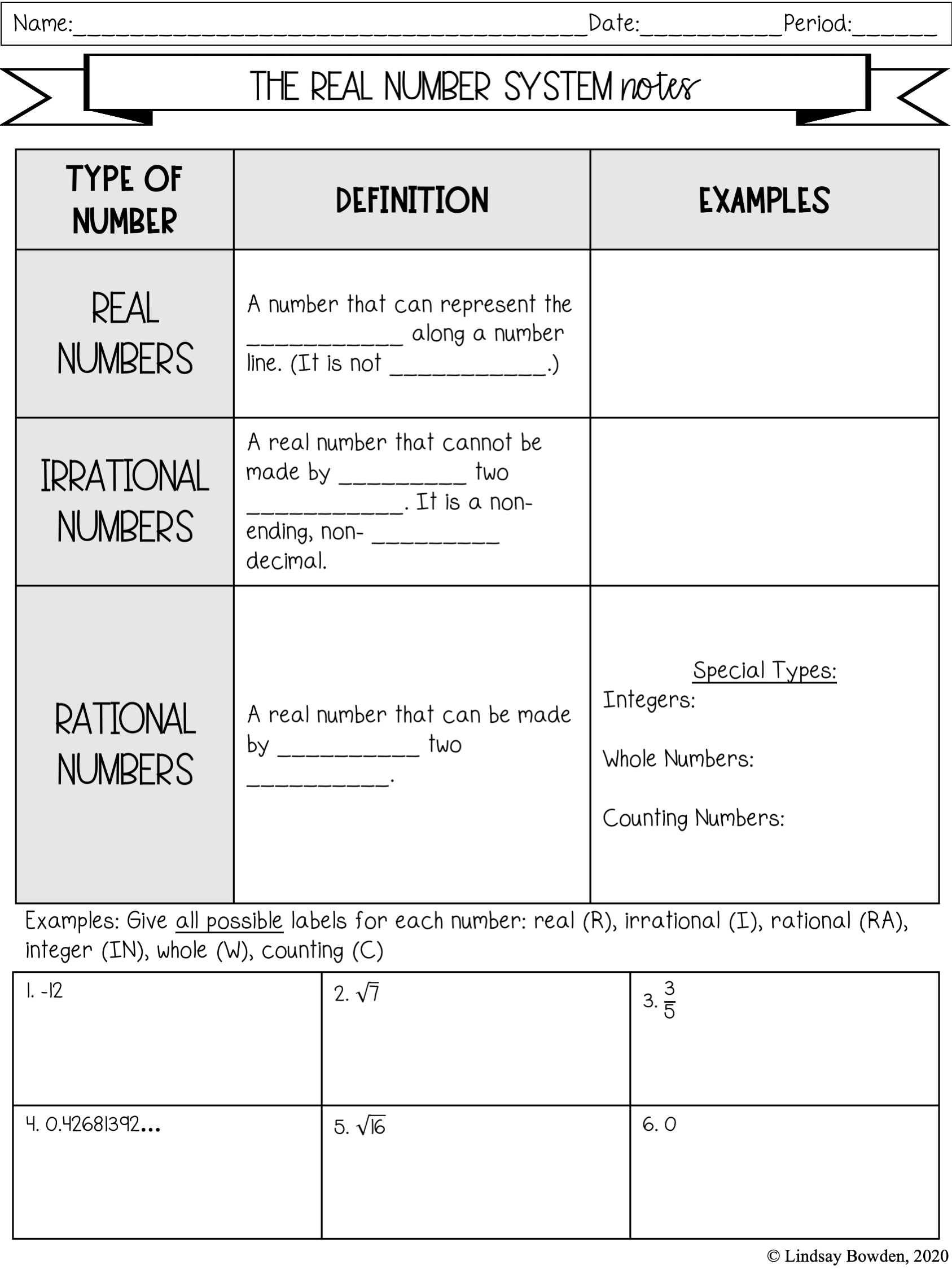
Understanding Real Numbers

Before delving into the identification methods, it’s crucial to grasp what constitutes real numbers. Real numbers include:
- Integers: Whole numbers (positive, negative, and zero).
- Rational Numbers: Numbers that can be expressed as a fraction of two integers, e.g., 1⁄2, 5⁄3.
- Irrational Numbers: Numbers that cannot be expressed as a fraction, such as π or √2.
- Floating Point Numbers: Numbers with a decimal point, e.g., 3.14, -5.25.

1. Decimal Representation

One of the simplest ways to spot real numbers is by their decimal representation. Any number that can be written with a decimal point or a fraction belongs to the realm of real numbers.
- If the decimal stops or repeats (e.g., 0.5, 0.125), it’s a rational number.
- If the decimal does not repeat or terminate (e.g., π = 3.14159…), it’s an irrational number.
2. Number Line Placement

Real numbers can be represented on a number line. Here’s how:
- Integers are located at the whole number points.
- Fractions and decimals are placed between these whole numbers.
📏 Note: Every point on the number line corresponds to a unique real number, with no gaps or overlaps.
3. Set Notation and Symbols
Mathematicians use specific notation to indicate sets of numbers:
- ℤ for integers
- ℚ for rational numbers
- ℝ for real numbers
If you see ℝ in a problem or on a worksheet, you’re dealing with real numbers.
4. Arithmetic Operations

Real numbers have well-defined arithmetic operations:
- Addition, subtraction, multiplication, and division yield real numbers (except division by zero).
This consistency in behavior helps identify real numbers in context.
5. Density Property

The density property states that between any two real numbers, there exists another real number. This can be used to check if numbers on a worksheet are real:
- If you can find an infinite number of real numbers between any two given numbers, they are real.
6. Completeness Axiom

Real numbers have an interesting property known as the completeness axiom, which essentially says that every bounded set of real numbers has a least upper bound. This can be a hint in identification:
- A sequence of real numbers that converges to a limit must have a limit that is also a real number.
7. Use of Functions

Functions can serve as a tool to identify real numbers:
- If a function f(x) has a domain or codomain of real numbers, then the outputs (x) and inputs (f(x)) must be real.
- Calculus, specifically integrals and derivatives, rely heavily on real numbers.
Real numbers are an essential concept in mathematics, not just for their inherent properties but also for their practical applications in various fields like physics, engineering, and finance. Understanding the different ways to identify real numbers helps in solving problems efficiently and accurately. By recognizing the characteristics of real numbers, from their placement on a number line to their behavior under arithmetic operations, one can navigate through mathematical challenges with confidence. This knowledge is foundational for anyone pursuing a deeper understanding of math, enabling them to identify, manipulate, and apply real numbers with precision and clarity in any worksheet or real-world scenario they encounter.
What are real numbers?

+
Real numbers include all rational (e.g., 1⁄2, 5) and irrational (e.g., √2, π) numbers. They can be any value that can be placed on a number line.
Can you give an example of how to use number line placement to identify real numbers?

+
Consider the number 2.5; it fits on the number line between 2 and 3, indicating it’s a real number. Additionally, between any two numbers like 2 and 3, you can always find another real number.
How do functions help in identifying real numbers?

+
If a function f(x) has real numbers as its input (domain) and output (codomain), then the numbers it deals with are real. This applies to most mathematical operations involving real numbers.