5 Ways To Calculate

Introduction to Calculations

Calculations are an essential part of our daily lives, and being able to perform them efficiently is crucial for making informed decisions. Whether it’s balancing a budget, measuring ingredients for a recipe, or determining the cost of a project, calculations play a vital role. In this article, we will explore five ways to calculate, each with its unique applications and benefits. From basic arithmetic operations to more complex mathematical concepts, we will delve into the world of calculations and provide you with the tools you need to become a proficient calculator.

1. Basic Arithmetic Operations

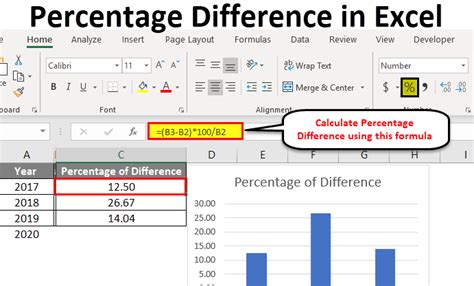
Basic arithmetic operations, such as addition, subtraction, multiplication, and division, are the foundation of calculations. These operations can be performed using various methods, including mental math, pen and paper, or calculators. To illustrate the importance of basic arithmetic operations, let’s consider a simple example: - Addition: 2 + 2 = 4 - Subtraction: 4 - 2 = 2 - Multiplication: 2 * 3 = 6 - Division: 6 ÷ 2 = 3 Mastering these basic operations is essential for more complex calculations and real-world applications.

2. Algebraic Calculations

Algebraic calculations involve solving equations and manipulating variables to isolate unknown values. This type of calculation is commonly used in science, technology, engineering, and mathematics (STEM) fields. For instance, consider the equation 2x + 5 = 11, where x is the unknown variable. To solve for x, we would subtract 5 from both sides of the equation, resulting in 2x = 6, and then divide both sides by 2, yielding x = 3. Algebraic calculations require a strong understanding of mathematical concepts and problem-solving strategies.

3. Geometric Calculations

Geometric calculations involve measuring and calculating the properties of shapes, such as perimeter, area, and volume. These calculations are essential in architecture, engineering, and design. For example, to calculate the area of a rectangle, we would multiply the length by the width (A = l * w). Geometric calculations require an understanding of spatial relationships and mathematical formulas.

4. Statistical Calculations

Statistical calculations involve analyzing and interpreting data to draw conclusions and make informed decisions. This type of calculation is commonly used in data analysis, research, and business. Statistical calculations include measures of central tendency, such as mean, median, and mode, as well as measures of variability, such as range and standard deviation. For instance, consider a dataset with the following values: 2, 4, 6, 8, 10. To calculate the mean, we would add the values and divide by the number of values (2 + 4 + 6 + 8 + 10 = 30, 30 ÷ 5 = 6).

5. Calculus Calculations

Calculus calculations involve studying rates of change and accumulation, and are commonly used in physics, engineering, and economics. Calculus includes two main branches: differential calculus and integral calculus. Differential calculus deals with rates of change and slopes of curves, while integral calculus deals with accumulation and area under curves. Calculus calculations require a strong understanding of mathematical concepts, such as limits and derivatives.

📝 Note: Mastering these five ways to calculate will help you develop a strong foundation in mathematics and improve your problem-solving skills.

To further illustrate the applications of these calculations, consider the following table:

Calculation Type	Application
Basic Arithmetic	Everyday transactions, budgeting
Algebraic Calculations	Science, technology, engineering, mathematics (STEM)
Geometric Calculations	Architecture, engineering, design
Statistical Calculations	Data analysis, research, business
Calculus Calculations	Physics, engineering, economics

In summary, calculations are an integral part of our daily lives, and mastering the five ways to calculate will help you develop a strong foundation in mathematics and improve your problem-solving skills. By understanding the applications and benefits of each type of calculation, you will be better equipped to tackle real-world problems and make informed decisions.