Solve Hard Multi Step Equations with Ease - Worksheet

How to Solve Multi-Step Equations

Multi-step equations can often appear complex and intimidating at first glance, but with the right approach, they can be solved systematically and with ease. Whether you're a student looking to master algebra, or someone refreshing your mathematical skills, understanding how to tackle multi-step equations will bolster your problem-solving toolkit. Let's dive into a comprehensive guide to solving these equations, backed by a practical worksheet for hands-on practice.
Basic Principles of Equations

Before we get into the specifics of multi-step equations, let's review the foundational principles:
- Equality: Both sides of an equation must remain equal; what you do to one side, you must do to the other.
- Distributive Property: Distribute multiplication over addition or subtraction where necessary.
- Combining Like Terms: Simplify expressions by adding or subtracting similar terms.
- Undo Operations: Perform the inverse operation to isolate the variable. For example, if you have an equation with addition, subtract to remove it.
Steps to Solving Multi-Step Equations
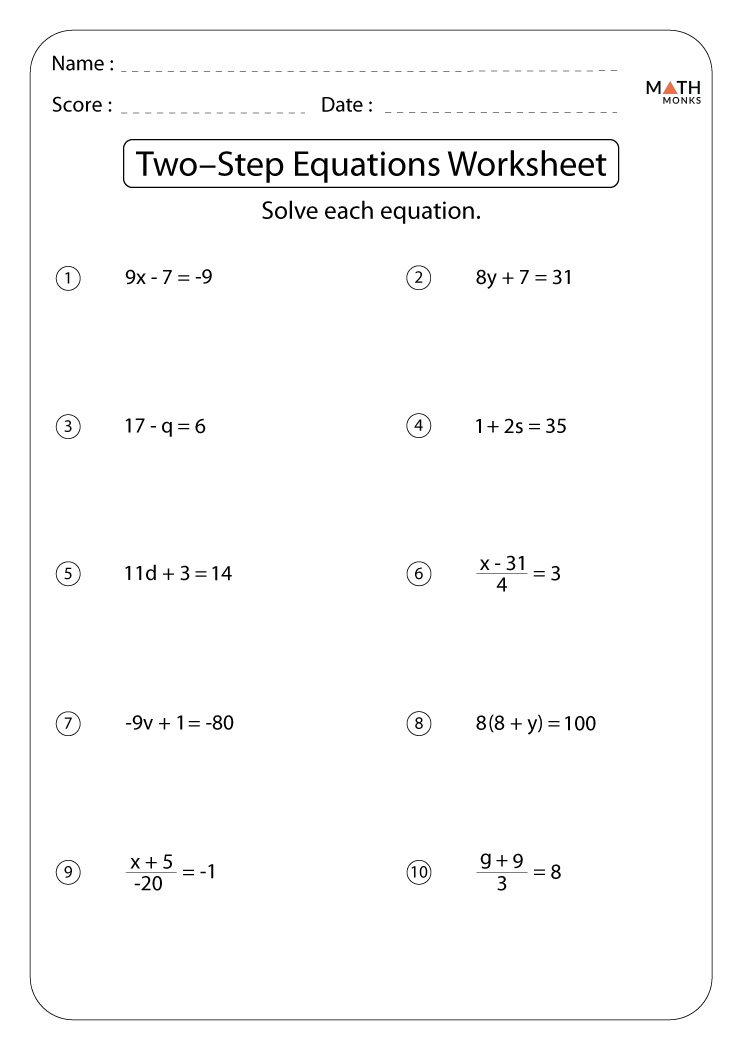
The key to solving multi-step equations is to follow a structured process. Here's a detailed guide:
Step 1: Remove Parentheses

If your equation contains parentheses, use the distributive property to remove them.
- Example: (2(x + 3) = 8) becomes (2x + 6 = 8) after distribution.
Step 2: Combine Like Terms

Once parentheses are gone, simplify by combining like terms. This reduces the number of terms you have to manage.
- Example: (2x + 4 + 5x - 7 = 15) simplifies to (7x - 3 = 15).
Step 3: Isolate the Variable

Your goal is to get the variable on one side of the equation. Here’s how:
- First, deal with constants by adding or subtracting them to both sides until the variable is the only thing left on its side.
- Then, isolate the variable by performing the inverse operations of the coefficients. If the coefficient is positive, divide; if negative, you can either divide by the negative number or multiply by its inverse (e.g., multiply by -1).
- Example: (7x - 3 = 15)
- Add 3 to both sides to get rid of the -3: (7x = 18)
- Divide both sides by 7: (x = 18⁄7)
Step 4: Check Your Work

After solving, substitute your solution back into the original equation to verify your answer.
- Example: For (2(x + 3) = 8), after solving for x, check: (2(\frac{18}{7} + 3) = 8).
✍️ Note: Always check your solution to avoid careless mistakes.
Common Mistakes to Avoid

Here are some common pitfalls to watch out for:
- Forgotten signs: Make sure you are careful with the signs of each term.
- Misapplication of the distributive property: Ensure you distribute multiplication correctly.
- Not isolating the variable: Failing to get the variable alone on one side can lead to incorrect solutions.
Practical Worksheet for Solving Multi-Step Equations

To reinforce what we've learned, let's work through a worksheet. Here's a table with equations to solve:
| Equation | Solution |
|---|---|
| 3(x + 2) = 15 | \[ x = 3 \] |
| 4 - 2x = -6 | \[ x = 5 \] |
| 3(2x + 1) - 4 = 8 | \[ x = 2 \] |
| 7x + 5 - 2x = 30 | \[ x = 5 \] |

Tips for Mastery

- Use visual aids: Drawing number lines or diagrams can help visualize the process.
- Practice with variations: Equations involving fractions, decimals, or negative coefficients offer good challenges.
- Work systematically: Break down the equation into steps before solving.
- Ask for feedback: Discussing your method with peers or tutors can improve your understanding.
By the end of this guide, you should feel more confident in your ability to solve multi-step equations. Remember, algebra is a language of logic and structure, and mastering it requires patience and consistent practice.
The journey to algebraic proficiency involves understanding, applying, and refining your techniques. With the approach outlined here, you'll find that these equations become less daunting, and your problem-solving skills will grow exponentially. Always remember to check your solutions, embrace mistakes as learning opportunities, and keep practicing.
What if an equation has more than one solution?

+
In higher algebra, you can encounter equations with multiple solutions, but in the context of solving basic multi-step equations, you’ll typically find a single solution. If you find your equation splits into two scenarios (like solving a quadratic), you should consider each case separately.
How do I handle fractions in equations?

+
If there are fractions, try to eliminate them early on by multiplying both sides of the equation by the least common denominator (LCD) to clear the fractions.
Can I use this method for inequalities?
+
Yes, but remember when multiplying or dividing both sides by a negative number, you must flip the inequality sign.