Graphing Quadratic Functions in Standard Form Made Easy

Understanding Quadratic Functions in Standard Form
Quadratic functions are a fundamental concept in algebra and are used to model a wide range of real-world phenomena, from the trajectory of a thrown ball to the shape of a satellite dish. In this blog post, we’ll focus on graphing quadratic functions in standard form, which is a crucial skill for any math student or enthusiast.
A quadratic function in standard form is written as:
f(x) = ax^2 + bx + c
where a, b, and c are constants, and x is the variable. The standard form is useful for graphing and analyzing the behavior of the function.
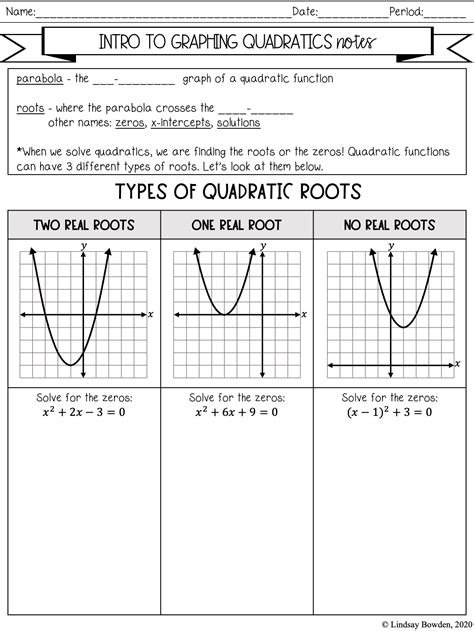
Key Features of Quadratic Functions in Standard Form

Before we dive into graphing, let’s review the key features of quadratic functions in standard form:
- Vertex: The vertex is the lowest or highest point on the graph, depending on the value of a. If a > 0, the vertex is a minimum point, and if a < 0, it’s a maximum point.
- Axis of Symmetry: The axis of symmetry is a vertical line that passes through the vertex and divides the graph into two mirror-image halves.
- X-Intercepts: The x-intercepts are the points where the graph crosses the x-axis. These points are found by setting f(x) = 0 and solving for x.
- Y-Intercept: The y-intercept is the point where the graph crosses the y-axis. This point is found by evaluating f(0).
Graphing Quadratic Functions in Standard Form

Now that we’ve reviewed the key features, let’s move on to graphing quadratic functions in standard form. Here are the steps:
- Determine the Vertex: Find the vertex by using the formula x = -b / 2a. This will give you the x-coordinate of the vertex. To find the y-coordinate, plug the x-coordinate into the function.
- Plot the Vertex: Plot the vertex on the graph.
- Determine the Axis of Symmetry: Draw a vertical line through the vertex. This is the axis of symmetry.
- Find the X-Intercepts: Set f(x) = 0 and solve for x. Plot the x-intercepts on the graph.
- Find the Y-Intercept: Evaluate f(0) and plot the y-intercept on the graph.
- Plot Additional Points: Choose a few x-values and calculate the corresponding y-values. Plot these points on the graph.
- Draw the Graph: Use the points you’ve plotted to draw a smooth curve.
📝 Note: Make sure to label the vertex, axis of symmetry, x-intercepts, and y-intercept on your graph.
Example: Graphing a Quadratic Function in Standard Form

Let’s graph the quadratic function f(x) = x^2 + 4x + 4.
- Determine the Vertex: x = -b / 2a = -4 / (2 * 1) = -2
- Plot the Vertex: The vertex is at (-2, 0).
- Determine the Axis of Symmetry: The axis of symmetry is the vertical line x = -2.
- Find the X-Intercepts: Set f(x) = 0 and solve for x. The x-intercepts are at (-2, 0).
- Find the Y-Intercept: Evaluate f(0) = 4. The y-intercept is at (0, 4).
- Plot Additional Points: Choose a few x-values and calculate the corresponding y-values.
| x | y |
|---|---|
| -1 | 3 |
| 0 | 4 |
| 1 | 3 |
| 2 | 0 |

- Draw the Graph: Use the points you’ve plotted to draw a smooth curve.
Tips and Tricks
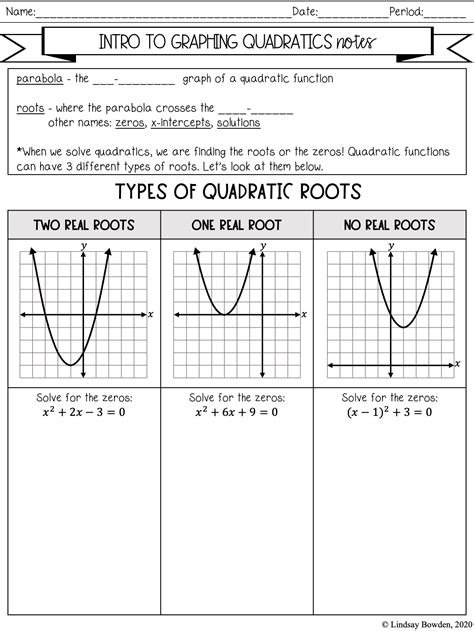
- Use a Graphing Calculator: If you have a graphing calculator, use it to graph the quadratic function. This can help you visualize the graph and check your work.
- Check Your Work: Once you’ve graphed the function, check your work by plugging in a few x-values to make sure the y-values match the graph.
- Practice, Practice, Practice: The more you practice graphing quadratic functions in standard form, the more comfortable you’ll become with the process.
By following these steps and tips, you’ll be well on your way to becoming a pro at graphing quadratic functions in standard form.
The key to mastering graphing quadratic functions in standard form is practice. With time and effort, you’ll become more comfortable with the process, and you’ll be able to graph these functions with ease. Remember to always label the vertex, axis of symmetry, x-intercepts, and y-intercept on your graph, and don’t be afraid to use a graphing calculator to check your work.
What is the standard form of a quadratic function?
+
The standard form of a quadratic function is f(x) = ax^2 + bx + c, where a, b, and c are constants, and x is the variable.
How do I find the vertex of a quadratic function in standard form?

+
The vertex can be found using the formula x = -b / 2a. This will give you the x-coordinate of the vertex. To find the y-coordinate, plug the x-coordinate into the function.
What is the axis of symmetry of a quadratic function?

+
The axis of symmetry is a vertical line that passes through the vertex and divides the graph into two mirror-image halves.
Related Terms:
- sketching quadratic graphs worksheet pdf
- graphing standard form worksheet pdf
- solving quadratics by graphing worksheet
- graphing quadratic functions printable
- graphing quadratics worksheet with answers
- graphing quadratic functions practice pdf



