Graphing Lines Made Easy: Free Worksheets

Understanding how to graph lines is a crucial skill in both algebra and geometry, offering foundational knowledge for many advanced mathematical concepts. Whether you're a student preparing for an upcoming test, a teacher looking for additional resources, or simply someone keen on refreshing your mathematical skills, mastering the art of graphing lines can open up a world of problem-solving capabilities. In this comprehensive guide, we'll walk through the process of graphing lines, utilizing free worksheets to make this learning journey interactive and effective.
What Does Graphing Lines Mean?

Graphing lines refers to the process of plotting points on a coordinate plane to create a visual representation of a linear equation. This technique helps us to see and analyze the relationship between variables directly. Here are the key components:
- The Slope-Intercept Form:
y = mx + b, where m is the slope and b is the y-intercept. - The Point-Slope Form:
y - y1 = m(x - x1), where (x1, y1) is a point on the line. - The Standard Form:
Ax + By = C, where A, B, and C are constants, and A should not be zero.
To graph a line, you need to:
- Identify the slope and y-intercept from the equation.
- Plot the y-intercept on the y-axis.
- Use the slope to find a second point by moving up or down (m units) and right or left (1 unit).
- Draw a line passing through these two points.
Using Free Worksheets to Learn
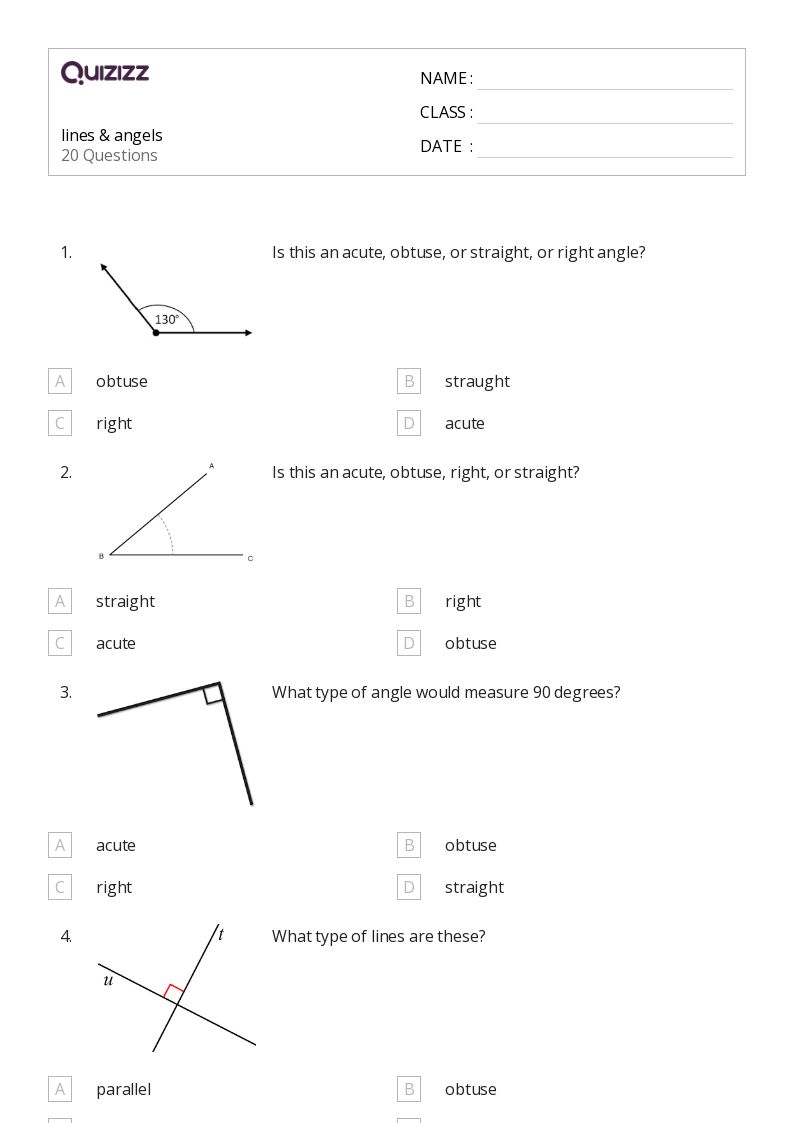
Free worksheets are an excellent resource for practice. They provide structured exercises that:
- Ensure a progression in difficulty to build confidence.
- Include examples with solutions for self-assessment.
- Offer visual aids to enhance understanding.
Types of Worksheets

Here's a categorization of worksheets you might encounter:
| Category | Description |
|---|---|
| Basic Graphing | Graphing simple equations with integer coordinates. |
| Slopes and Intercepts | Finding and graphing using the slope and y-intercept. |
| Word Problems | Translating word problems into linear equations and graphing. |
| Mixed Practice | Worksheets offering various types of line graphing problems. |

How to Use Worksheets Effectively

- Start with the Basics: Begin with worksheets that focus on plotting points and drawing lines through them.
- Understand Slopes: Use worksheets that delve into different slopes (positive, negative, zero, and undefined) to understand how they affect the line’s direction.
- Practice with Word Problems: Convert real-world scenarios into linear equations for a practical understanding.
- Time Yourself: After mastering the basics, time yourself to improve speed and accuracy.
- Check Your Answers: Many worksheets provide answer keys; ensure you check your work to understand where you might go wrong.
📝 Note: Worksheets can be customized or adjusted for difficulty, making them a versatile tool for self-paced learning.
Advanced Graphing Techniques

Once you’re comfortable with the fundamentals, you can explore more advanced techniques:
- Parallel and Perpendicular Lines: Understanding the relationship between slopes of lines that are parallel or perpendicular to each other.
- Systems of Equations: Graphing multiple lines to find their intersection point(s) or to understand they are parallel or coincident.
- Transformations: Learning how lines move across the coordinate plane through translations, reflections, and rotations.
Interactive Tools and Resources

Alongside worksheets, there are various interactive online tools that can enhance your learning experience:
- Online Graphing Calculators: Plot lines and analyze equations interactively.
- Educational Apps: Apps that provide step-by-step guidance and interactive exercises.
- Virtual Classroom Platforms: Participate in real-time graphing sessions with instructors or peers.
📚 Note: Incorporating these tools with traditional worksheets creates a well-rounded learning environment.
After mastering the art of graphing lines through practice, you gain not just the ability to visualize linear equations but also a deeper understanding of how mathematics applies to real-world scenarios. This skill enables you to:
- Model various real-life situations with linear equations.
- Solve problems in physics, economics, and engineering with graphical representations.
- Develop stronger problem-solving and analytical thinking.
Remember, the key to success is consistent practice. By regularly engaging with different worksheets, problems, and utilizing interactive tools, you not only improve your graphing skills but also your overall mathematical proficiency. Keep exploring, practicing, and you'll find graphing lines becomes intuitive and even enjoyable.
What is the importance of understanding slopes in graphing lines?

+
Slopes are fundamental in graphing lines because they describe how steep the line is and in which direction it moves. Understanding slope helps in predicting the behavior of a line, which is crucial in mathematics, physics, and real-life applications.
Can I use graphing software instead of pen-and-paper worksheets?

+
Yes, graphing software can complement traditional worksheets by offering a more dynamic way to visualize lines. However, manual graphing practice is crucial for understanding the process deeply.
Are there any tips for quickly identifying the slope and y-intercept of an equation?

+
Here are some tips:
- Look for the coefficient of x, which is m in
y = mx + b. - Identify b, which is the constant term, as the y-intercept.
- Practice converting equations into slope-intercept form quickly.