Geometry is an essential part of mathematics, not only because of its practical applications but also because it provides an excellent framework for understanding abstract mathematical concepts. One of the fascinating areas within geometry is the study of transformations, where objects are moved without altering their shape or size. Here, we delve into the world of geometric transformations through a collection of engaging worksheets that can make learning both fun and educational for students. Whether you're a teacher looking to introduce or reinforce transformation concepts or a student eager to master these skills, these worksheets can be incredibly beneficial.
The Fundamentals of Geometry Transformations
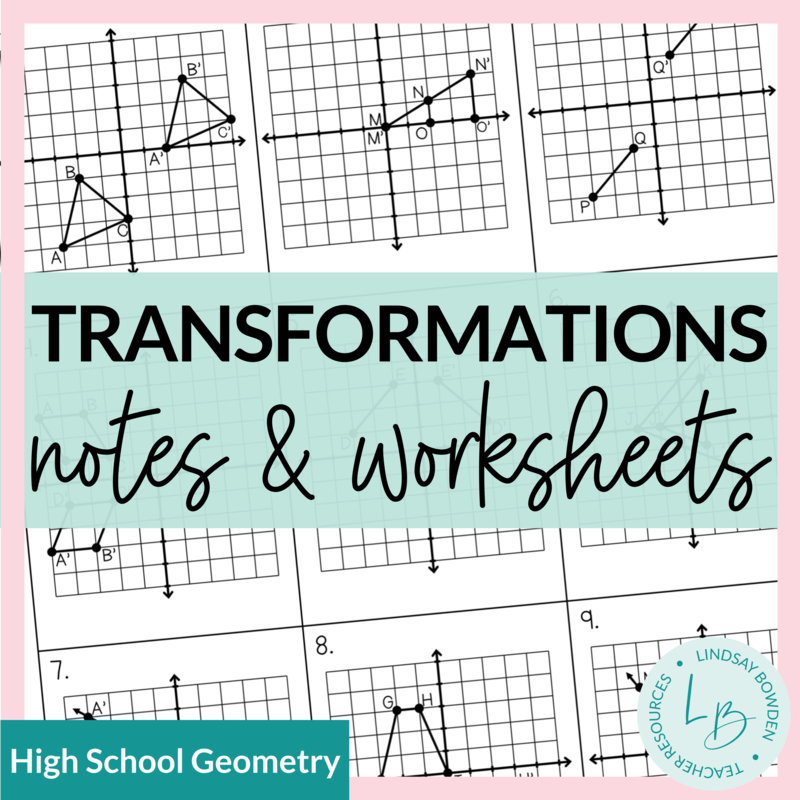
Before we jump into the worksheets, let’s quickly review the key types of transformations:
- Translation – Moving a shape without rotating or resizing it.
- Reflection – Flipping a shape over a line to create a mirror image.
- Rotation – Spinning a shape around a fixed point.
- Dilation – Resizing a shape, either enlarging or shrinking it, maintaining proportions.
- Composite Transformations – Combinations of the above transformations.
Worksheet 1: Translation and Coordinate Planes
In this worksheet, students are introduced to translations. They work with:
- Finding the new coordinates after translating a given shape.
- Drawing translated shapes on a coordinate plane.
- Calculating distances of translations.
An 
might be useful here to visualize how a triangle translates on a coordinate plane.
Worksheet 2: Reflections Over Various Axes
Reflection is another critical transformation where students explore:
- Reflecting shapes over the x-axis, y-axis, and lines of symmetry.
- Calculating the coordinates of reflected points.
- Understanding properties of reflection, like congruence and orientation.
💡 Note: The reflection always results in a congruent shape.
Worksheet 3: Rotations Around Points
Here, students delve into rotations:
- Rotating shapes around specific points, including the origin.
- Determining angles of rotation and new coordinates.
- Exploring the effect of positive and negative rotations.
Visual aids like 
can help students visualize rotations effectively.
| Transformation | Example of Operation | Key Point |
|---|---|---|
| Rotation | Turn 90° clockwise | Angle direction matters |
| Translation | Move (3, 2) units to the right and up | Coordinates change uniformly |
| Reflection | Over the x-axis | Opposite y-coordinate |
Worksheet 4: Dilation and Proportions
This worksheet focuses on dilation:
- Enlarging or shrinking shapes by given scale factors.
- Calculating the dimensions of dilated figures.
- Understanding how dilation affects angles and similarity.
An illustration can be added here showing different dilations of a shape 
.
Worksheet 5: Composite Transformations
The final worksheet deals with complex transformations:
- Performing a series of transformations on a shape.
- Determining the final position of a shape after multiple transformations.
- Exploring the commutative property of transformations.
Examples of composite transformations like “Translate, then Rotate” can be depicted with images or diagrams.
These geometry transformation worksheets not only provide students with the opportunity to practice and deepen their understanding of transformations but also help them visualize and conceptualize spatial relationships. By engaging with these exercises, students learn to manipulate geometric figures in both practical and abstract ways, which is crucial for advanced studies in mathematics and other related fields.
Why are geometry transformations important?
+
Geometry transformations are essential because they help understand the properties of shapes, how they can move or change in space, and their symmetry. They also lay the foundation for understanding more complex mathematical concepts like matrix transformations in linear algebra.
How can teachers incorporate these worksheets into their lesson plans?
+
Teachers can introduce these worksheets as part of lessons focusing on specific transformations. They can start with simpler worksheets and progress to more complex ones as students gain proficiency. Group activities, where students collaborate to solve transformations, can also be educational.
What are the common mistakes students make with transformations?
+
Common errors include confusing reflection with translation, misunderstanding the direction of rotations, and not maintaining the proportions in dilation. Practice through worksheets can help mitigate these mistakes.
Can these transformations be applied outside of the classroom?
+
Absolutely! Transformations are used in fields like computer graphics, architecture, engineering, and physics. For example, understanding rotations is crucial for 3D modeling, and dilations are used in scaling architectural plans.
