Mastering Triangle Congruence: Geometry Worksheet Proofs

Embarking on the journey of mastering triangle congruence in geometry can be a daunting task for many students. The concept involves understanding how triangles can be proven congruent through various methods, which are essential for deeper mathematical study and real-world applications in fields like architecture, engineering, and beyond. This comprehensive guide will delve into the intricacies of triangle congruence, providing in-depth explanations, methods, and practical exercises to solidify your understanding.
Understanding Triangle Congruence

Triangle congruence refers to two or more triangles that have the same size and shape, meaning all corresponding sides and angles are equal. Here are the primary methods to prove that two triangles are congruent:
- Side-Side-Side (SSS) Congruence: If three sides of one triangle are equal to three sides of another triangle, the triangles are congruent.
- Side-Angle-Side (SAS) Congruence: If two sides and the included angle of one triangle are equal to two sides and the included angle of another triangle, the triangles are congruent.
- Angle-Side-Angle (ASA) Congruence: If two angles and the included side of one triangle are equal to two angles and the included side of another triangle, the triangles are congruent.
- Angle-Angle-Side (AAS) Congruence: If two angles and a non-included side of one triangle are equal to two angles and the corresponding non-included side of another triangle, they are congruent.
- Right Angle-Hypotenuse-Side (RHS) Congruence: For right triangles, if the hypotenuse and one leg of one triangle are equal to the hypotenuse and one leg of another triangle, the triangles are congruent.
Worksheet Proofs for Triangle Congruence
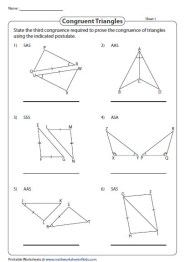
When it comes to proving triangle congruence through worksheets, here is how you can structure your practice:
Step-by-Step Proofs

- Identify the Given Information: Begin by listing what you know from the problem statement. This could include lengths of sides, measures of angles, or other geometric properties.
- Draw a Diagram: Visualize the triangles involved. Sketching can help in identifying additional information that might not be directly provided.
- Choose the Correct Congruence Postulate: Based on the given information, select which method (SSS, SAS, ASA, etc.) is most appropriate to use for proving congruence.
- Write the Proof: Step-by-step, outline your logic, showing each step that leads to the conclusion of congruence.
Example Worksheet

Consider the following problem:
| Given | Proof |
|---|---|
| In ∆ABC and ∆DEF, AB = DE, BC = EF, and AC = DF | 1. Given that AB = DE, BC = EF, and AC = DF. 2. Therefore, by SSS Congruence, ∆ABC is congruent to ∆DEF. |

📚 Note: When using diagrams or sketches to solve problems, ensure accuracy in your drawings to avoid misleading yourself with incorrect geometric assumptions.
Advanced Techniques in Triangle Congruence Proofs

Beyond the basic congruence postulates, there are additional strategies to enhance your proficiency:
- Isosceles Triangle Theorem: If two sides of a triangle are congruent, then the angles opposite these sides are also congruent.
- CPCTC (Corresponding Parts of Congruent Triangles are Congruent): Once triangles are proven congruent, you can use this to state that any corresponding part (side or angle) of these triangles are congruent.
- Using Midpoints and Parallels: Properties related to midpoints, parallel lines, and transversals can help in proving congruence by creating additional congruent triangles.
Practical Applications of Triangle Congruence

Understanding triangle congruence isn't just for solving geometry problems; it has practical applications:
- Surveying and Mapping: Establishing congruent triangles helps in accurately measuring land plots and determining distances without physically measuring each section.
- Design and Construction: Architects and engineers rely on these principles to ensure structural stability and design symmetry.
- Computer Graphics: Algorithms for rendering 3D graphics often depend on geometric congruence to create realistic images.
By consistently practicing with various types of problems and real-world scenarios, you can enhance your ability to spot congruence quickly and apply it effectively.
As we conclude our exploration of triangle congruence, remember that the key to mastery lies in understanding the principles deeply and practicing their application regularly. Each method of proving triangle congruence—SSS, SAS, ASA, AAS, and RHS—offers a unique lens through which to view geometric problems. This knowledge not only aids in solving complex mathematical exercises but also enriches your understanding of the world around you where symmetry and precision play crucial roles. Whether it's in the layout of cities, the design of structures, or the patterns found in nature, triangle congruence is a fundamental concept that intertwines with everyday life.
What is the difference between congruence and similarity in triangles?

+
Congruence means two triangles have the same size and shape, whereas similarity means the triangles have the same shape but potentially different sizes. Congruent triangles have all corresponding sides and angles equal, while similar triangles have corresponding angles equal but sides proportional.
Why is CPCTC important in triangle congruence proofs?
+
CPCTC, or “Corresponding Parts of Congruent Triangles are Congruent,” is crucial because it allows you to conclude that any part of one triangle is congruent to the corresponding part of another if the triangles themselves are congruent. This principle is often used as the final step in many geometric proofs to establish relationships between individual sides or angles.
Can triangle congruence be applied to other polygons?

+
Triangle congruence criteria are unique to triangles due to their fixed number of angles and sides. For other polygons, you would generally use methods like SSS (side-side-side) or SAS (side-angle-side) congruency applied iteratively or techniques like SSS for quadrilaterals which involves proving two triangles are congruent within the quadrilateral.