5 Geometry Worksheet Answers for Points, Lines, Planes

Geometry is a fascinating subject that forms the basis of spatial understanding in mathematics. It involves the study of properties, measurements, and constructions of various figures and shapes. For students and learners, understanding the basics like points, lines, and planes is crucial as they are the building blocks of all geometric constructions. Here, we delve into some key aspects of geometry with specific focus on points, lines, and planes, providing explanations and answers to common worksheet questions to help solidify your grasp of these foundational concepts.
Understanding Points

A point in geometry is the most basic object, representing a precise location in space. It has no dimensions, meaning it has no length, width, or depth. Here's what you need to know:
- Naming: A point is typically named with a single capital letter, for example, Point A or Point P.
- Representation: Points can be visualized as dots on a plane or in space.
Common Worksheet Questions:

- What is the notation used to represent points?
- How many points are required to define a line?
- Can points have size?
🖍️ Note: Although points are theoretically dimensionless, they are represented with a small dot on paper to aid visualization.
Lines in Geometry

Lines are straight paths that extend indefinitely in both directions. Here’s a deeper look into lines:
- Definition: A line extends in both directions with no end.
- Properties: Lines are straight, have length but no width, and are infinite.
- Representation: A line can be named by any two points on it or by a lowercase letter.
Key Questions:
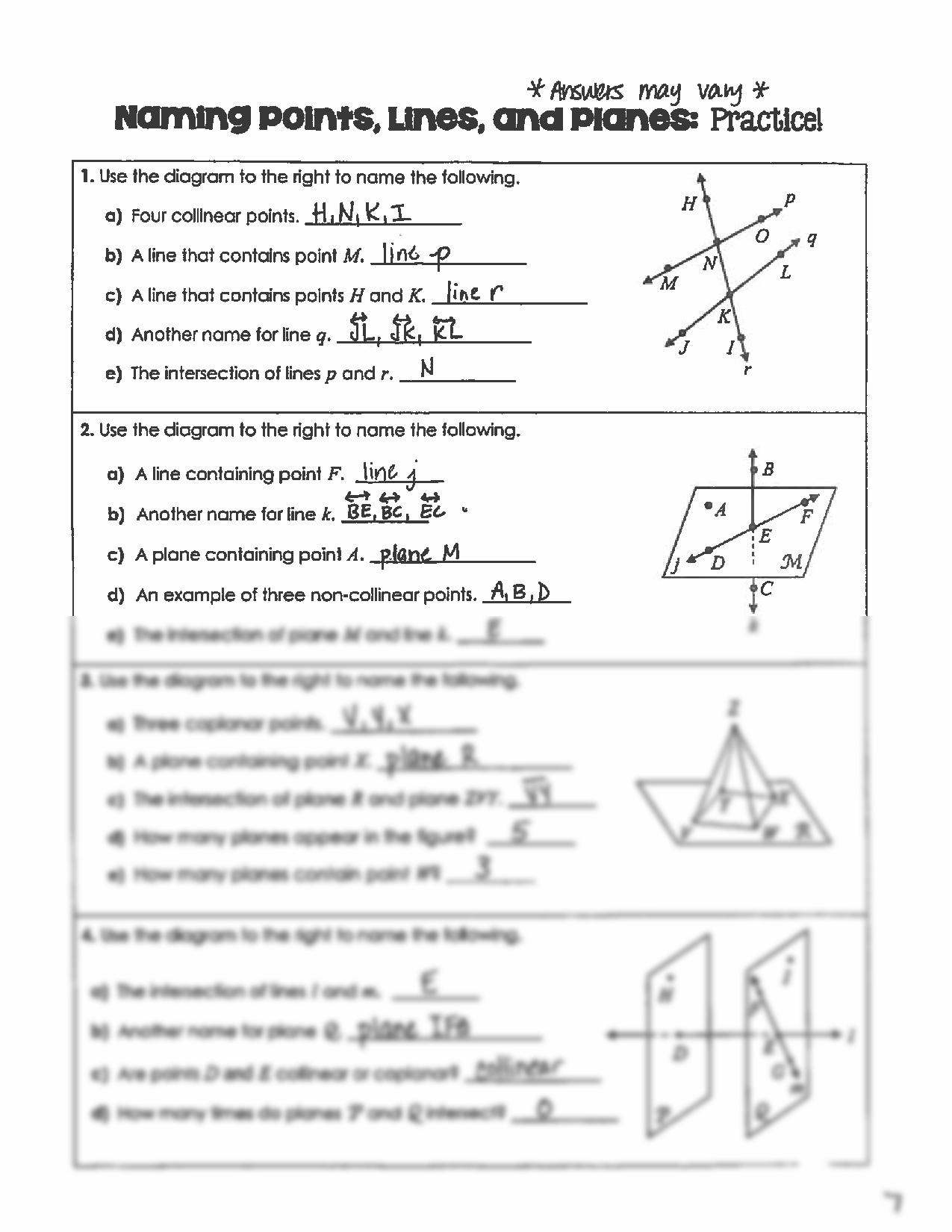
- How do you name a line?
- What's the difference between a line segment and a ray?
- What does it mean for lines to be parallel?
A line segment is part of a line with two endpoints, while a ray extends indefinitely from one endpoint. Parallel lines never intersect because they remain the same distance apart.
Exploring Planes

Planes in geometry are flat surfaces that extend indefinitely in all directions. Here's what you should know:
- Characteristics: Planes are two-dimensional and have infinite length and width but no thickness.
- Naming: Planes are usually denoted by a single capital letter or by three points not all in a straight line.
- Visualisation: Imagine a huge sheet of paper that never ends; this is how you can visualize a plane.
Worksheet Problems:

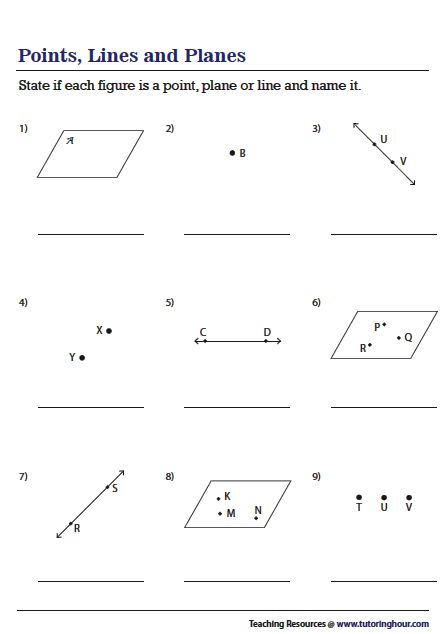
- What are the possible ways to name a plane?
- Can two planes intersect in a point? Can three points always define a plane?
⚠️ Note: Two planes can intersect in a line, not a point, unless they are parallel or coincide.
Interactions between Points, Lines, and Planes

Understanding how these basic elements interact is key to mastering geometry:
- Collinear Points: Points lying on the same line.
- Coplanar Points: Points or lines lying on the same plane.
- Intersecting Lines: Lines that meet at a single point.
- Skew Lines: Lines that do not lie in the same plane and do not intersect.
Examples and Answers:
| Concept | Question | Answer |
|---|---|---|
| Collinear Points | Are points A, B, and C collinear if AB + BC = AC? | Yes, if AB + BC equals AC, they are collinear. |
| Parallel Lines | Can two lines be both parallel and intersect? | No, parallel lines never intersect. |
| Planes Intersection | What is the intersection of two distinct planes? | It's a line unless they are parallel. |

In wrapping up this exploration of points, lines, and planes, we’ve covered fundamental concepts that underpin geometric studies. This journey through the basic elements of geometry not only provides answers to common worksheet questions but also enhances your understanding of spatial relationships. By grasping these basics, you lay a strong foundation for further explorations into geometry, whether it be through more complex shapes, theorems, or practical applications in real life.
Can a point lie on multiple planes?

+
Yes, a point can lie on an infinite number of planes since any three non-collinear points define a unique plane.
What is the minimum number of points needed to define a line?

+
Only two distinct points are needed to define a unique line.
What are skew lines?

+
Skew lines are lines that do not lie in the same plane and do not intersect.
How many planes can pass through a single point?

+
An infinite number of planes can pass through a single point, as you can rotate around the point to form different planes.
What does it mean for points to be coplanar?

+
Points are said to be coplanar if they lie on the same plane.