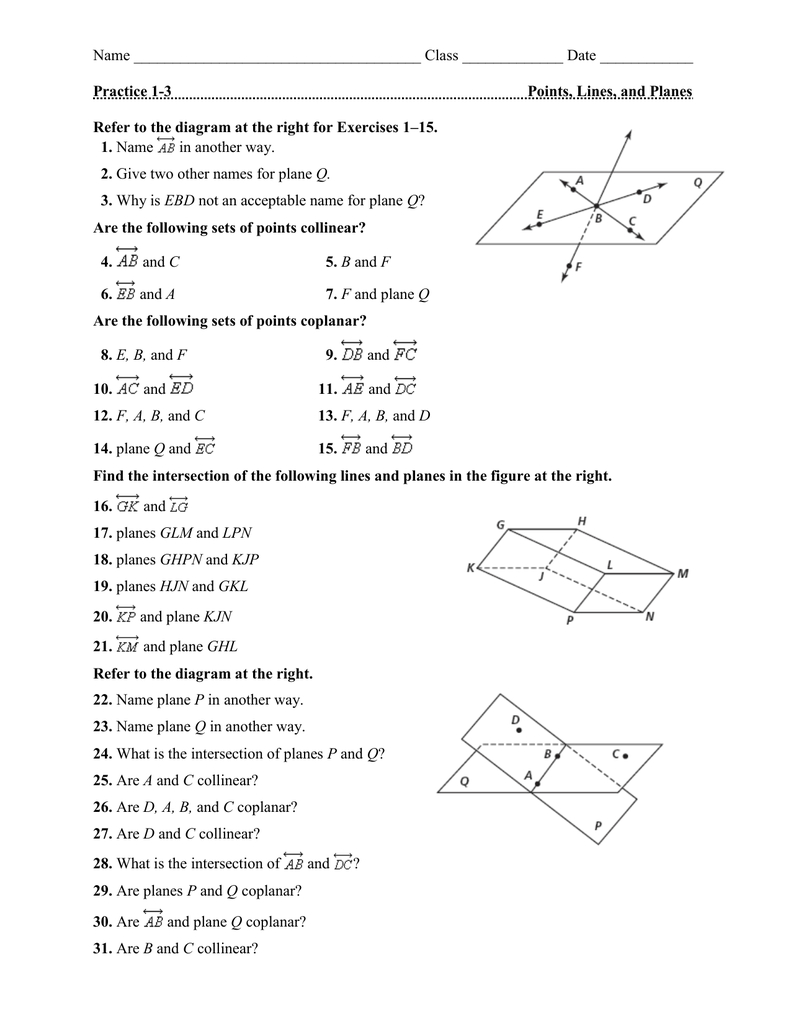
Geometry Worksheet: 5 Must-Know Points, Lines, Planes Answers

The world of geometry is a fascinating realm filled with shapes, lines, and points, which serve as the building blocks for understanding the physical world around us. Geometry isn't just for architects or engineers; it's for everyone interested in spatial relationships and the mathematical logic that governs our environment. In this comprehensive guide, we'll delve into some essential points, lines, and planes answers that are crucial for mastering geometric concepts.
Understanding Points


In the simplest terms, a point is a location in space without size or dimension. It’s like an infinitely small dot, which has no length, width, or height. Here are some key points to understand:
- Definition: A point is often denoted by a dot and can be named using a capital letter, like “A”, “B”, or “C”.
- Position: Points can exist in one, two, or three-dimensional space.
- Collinearity: Points that lie on the same line are called collinear points.
- Non-collinear Points: These are points that do not lie on the same straight line.
Understanding Lines


A line is fundamentally a straight, infinitely extending set of points, stretching in two opposite directions. Here’s what you need to know:
- Infinite: A line extends infinitely in both directions, denoted with arrowheads (↔).
- Segment: A line segment is a portion of a line with two endpoints; it has a definite length.
- Ray: A ray is a line that starts at a point and extends infinitely in one direction only.
- Parallel Lines: Lines that never intersect, even if extended infinitely, are parallel.
- Intersecting Lines: Lines that cross each other at a point.
Understanding Planes


A plane is a flat, two-dimensional surface that extends infinitely in all directions. Here are crucial points:
- Infinite Surface: A plane continues forever in all directions.
- Planes Intersection: Two planes intersect along a straight line.
- Coplanar: Points or lines that lie on the same plane are coplanar.
- Non-coplanar: These points or lines do not lie on the same plane.
Relating Points, Lines, and Planes

Understanding the relationships between points, lines, and planes forms the backbone of geometric problem-solving. Here’s how they interact:
- Point and Line: A point defines where a line starts or ends. Infinite lines can be drawn through any point.
- Line and Plane: A plane can intersect a line at one point (if they are skew), at every point along the line (if the line lies within the plane), or not at all (if they are parallel).
- Plane and Plane: Two planes intersect along a line or are parallel, never intersecting.
Applications of Points, Lines, and Planes


Geometry isn’t just a theoretical exercise; it has practical applications:
- Architecture: Lines and planes define the structure and space of buildings.
- Computer Graphics: Points, lines, and planes form the basis for 3D modeling and rendering.
- Navigation: Maps use points (locations), lines (routes), and planes (elevation models).
- Physics: Understanding the physical world requires geometric concepts for analyzing trajectories, fields, and forces.
🌟 Note: Learning these fundamental concepts is like learning the alphabet in geometry. Once you understand points, lines, and planes, more complex shapes and theorems will become easier to grasp.
Having covered these essential aspects of geometry, let's not forget that while these concepts might seem simple, they form the foundation for more advanced geometric studies. The beauty of geometry lies in its simplicity and the immense complexity it can describe. With a solid grasp of these fundamentals, anyone can appreciate and engage with the beautiful symmetries, structures, and patterns that make up the world around us.
What are the basic elements of geometry?

+
The basic elements of geometry are points, lines, and planes. Points are dimensionless locations in space, lines are one-dimensional sets of points extending infinitely, and planes are flat surfaces extending infinitely in two dimensions.
How do you define a plane in geometry?

+
A plane is defined as a flat, two-dimensional surface that extends infinitely in all directions. Any three points not on the same line determine a unique plane.
What is the difference between collinear and coplanar points?

+
Collinear points are points that lie on the same straight line, while coplanar points lie on the same plane. All collinear points are also coplanar, but not all coplanar points are collinear.