5 Essential Tips for Geometry Parallel Line Mastery
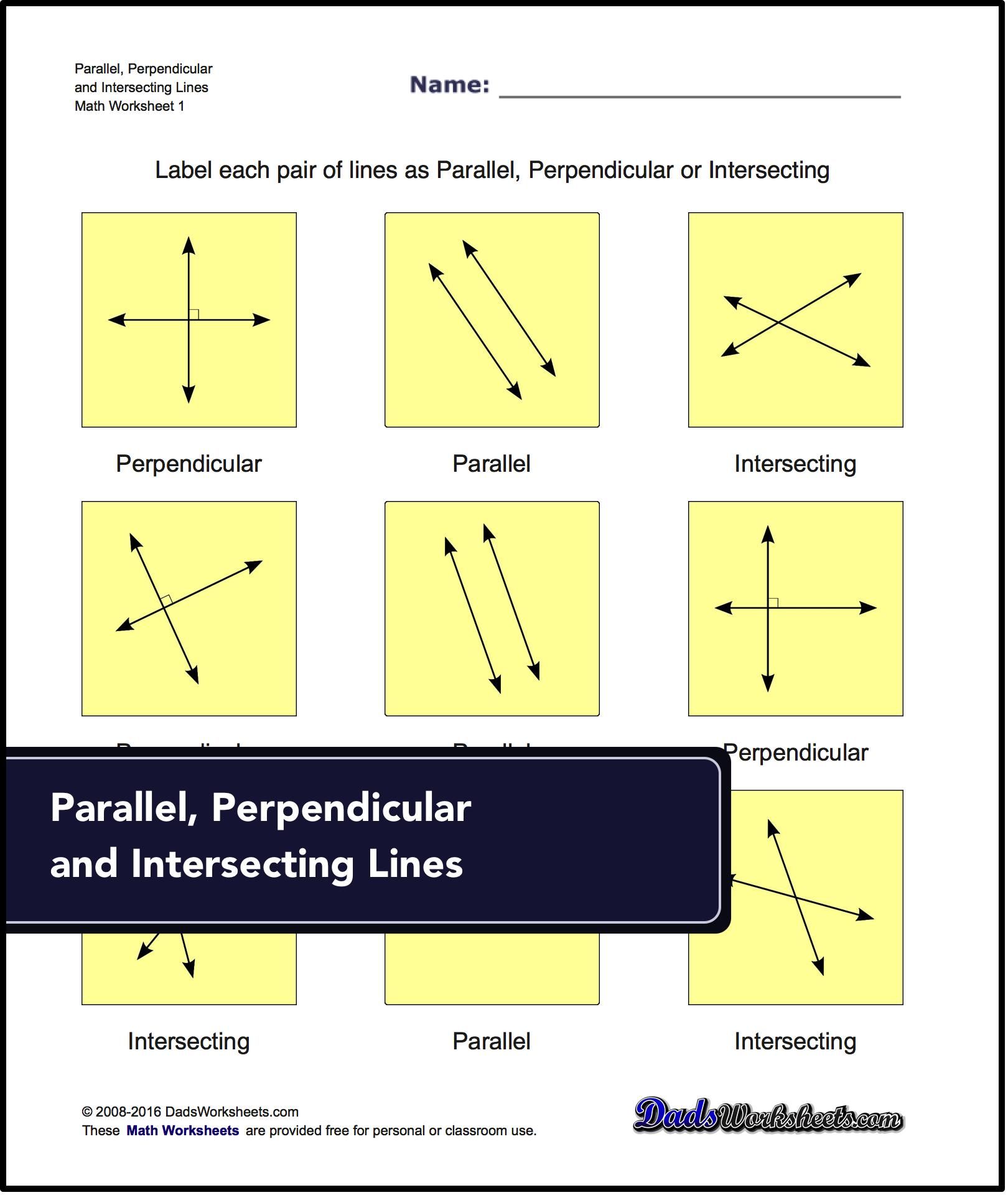
In the world of mathematics, geometry plays an essential role, and understanding the concept of parallel lines is fundamental. Parallel lines are lines that never intersect, no matter how far they are extended. Mastering the intricacies of parallel lines can provide a solid foundation not only in geometry but also in various other mathematical and real-world applications like architecture, design, and urban planning. Here, we explore five crucial tips to master this concept effectively.
1. Understand the Definition and Properties
Definition: Two lines are parallel if they have the same slope but different y-intercepts. They never cross each other, maintaining an equal distance from one another throughout their length.
- Properties:
- Transversal Angles: When a transversal line intersects two parallel lines, it creates several pairs of corresponding, alternate interior, and co-interior angles.
- Equal Angle Theorems: Corresponding angles are equal, alternate interior angles are equal, and the sum of co-interior angles is 180 degrees.
- Parallelism Tests: If any of the angle conditions for parallelism are met, the lines are parallel.

2. Visualize and Draw

Visual learning is incredibly powerful in geometry. Here are some steps to enhance your understanding through visualization:
- Start with a sheet of grid paper. Draw two lines with the same slope.
- Extend these lines to show that they never intersect.
- Introduce a transversal line that intersects both parallel lines. Label the angles to analyze their relationships.
- Use color-coding for different types of angles to differentiate them visually.
🖍️ Note: By sketching, you not only internalize the properties of parallel lines but also understand how changes in one angle affect the others.
3. Practice Angle Calculation

Geometry is not only about theory but also about practical application. Here’s how you can practice:
- Calculate the angles created by parallel lines cut by a transversal.
- Solve problems where you need to find unknown angles by applying the properties of parallel lines.
| Angle Pair | Relationship |
|---|---|
| Corresponding Angles | Equal |
| Alternate Interior Angles | Equal |
| Co-interior Angles | Sum to 180 degrees |

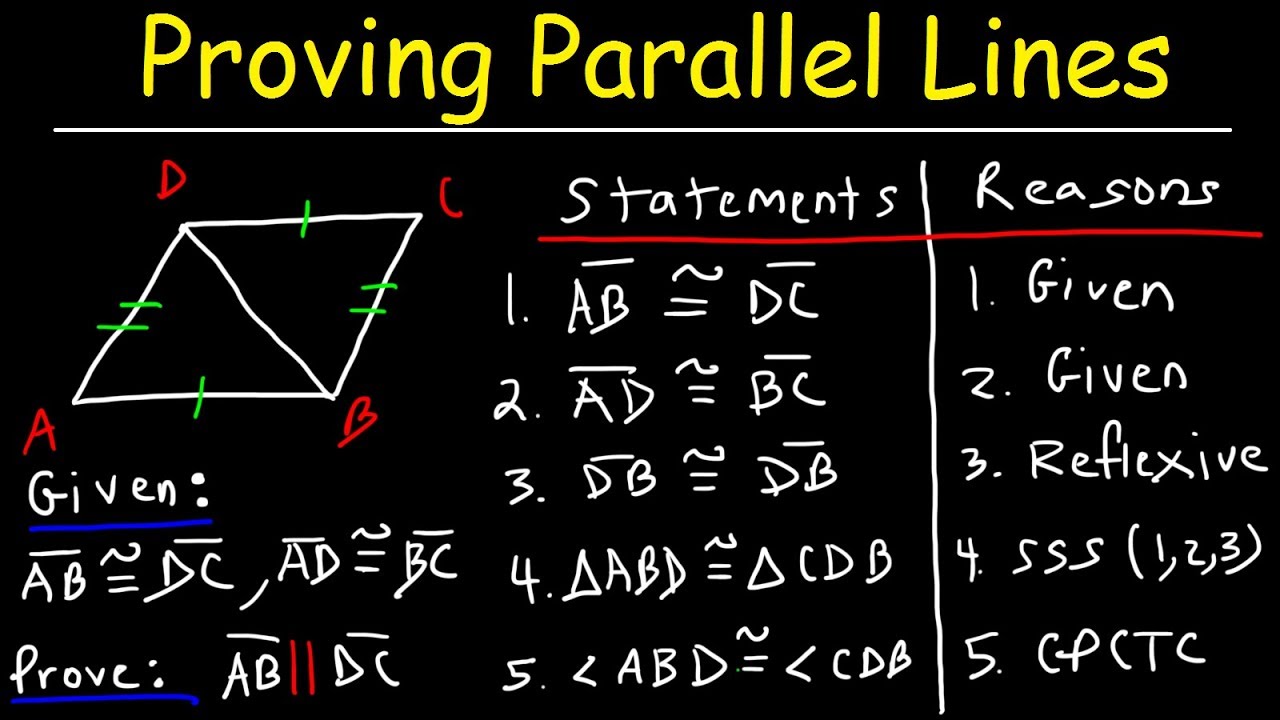
4. Use Proofs to Understand Better

Proofs are the backbone of geometry, providing logical steps to justify why certain properties hold:
- Proof of Parallelism: Demonstrate that if two lines are cut by a transversal and the alternate interior angles are equal, then the lines are parallel.
- Converse Properties: Prove that if lines are parallel, then specific angles formed must be equal or supplementary.

5. Real-World Application

Linking theory to practice by recognizing and solving real-world problems:
- Architecture: Understand how parallel lines are used in building design, where vertical supports must be parallel to ensure structural integrity.
- Road Planning: Observe how roads are designed to keep vehicles moving in parallel lanes to avoid collisions.
- Art and Design: Analyze artworks or designs where the use of perspective creates an illusion of parallel lines converging at a vanishing point.
In wrapping up, mastering parallel lines in geometry is not just about learning their properties; it’s about seeing the world through a different lens. Understanding how these lines work together to form angles, how they intersect or don’t, and applying this knowledge practically can enhance problem-solving skills and spatial reasoning. Remember, practice, visualization, and real-world application are your best allies in this journey. Whether you’re calculating angles, constructing proofs, or designing, the principles of parallel lines provide a foundation for geometric exploration and understanding.
Why are parallel lines important in geometry?

+
Parallel lines are fundamental in geometry because they form the basis for understanding angles, proofs, and many geometric constructions. They are used in theorems and real-world applications where maintaining consistent direction and spacing is crucial.
How do I identify parallel lines?

+
Parallel lines can be identified by their slope in a coordinate system, or visually, if they seem to extend infinitely without ever meeting. In a diagram, look for symbols indicating parallel lines, or use angle properties when lines are cut by a transversal.
Can parallel lines ever meet?

+
By definition, parallel lines do not meet in a Euclidean plane, no matter how far you extend them. However, in non-Euclidean geometries like spherical or hyperbolic, this rule might not hold true.