Geometry Basics: Distance & Midpoint Formula Answers

In the fascinating world of geometry, understanding the basics can unlock a universe of possibilities in mathematics, engineering, and even everyday life. Two fundamental concepts that form the bedrock of spatial understanding are the distance formula and the midpoint formula. These formulas are essential tools that help us navigate through the coordinates on a plane, providing solutions to numerous problems. Let's delve into what these formulas mean, how they work, and their applications.
Understanding the Distance Formula

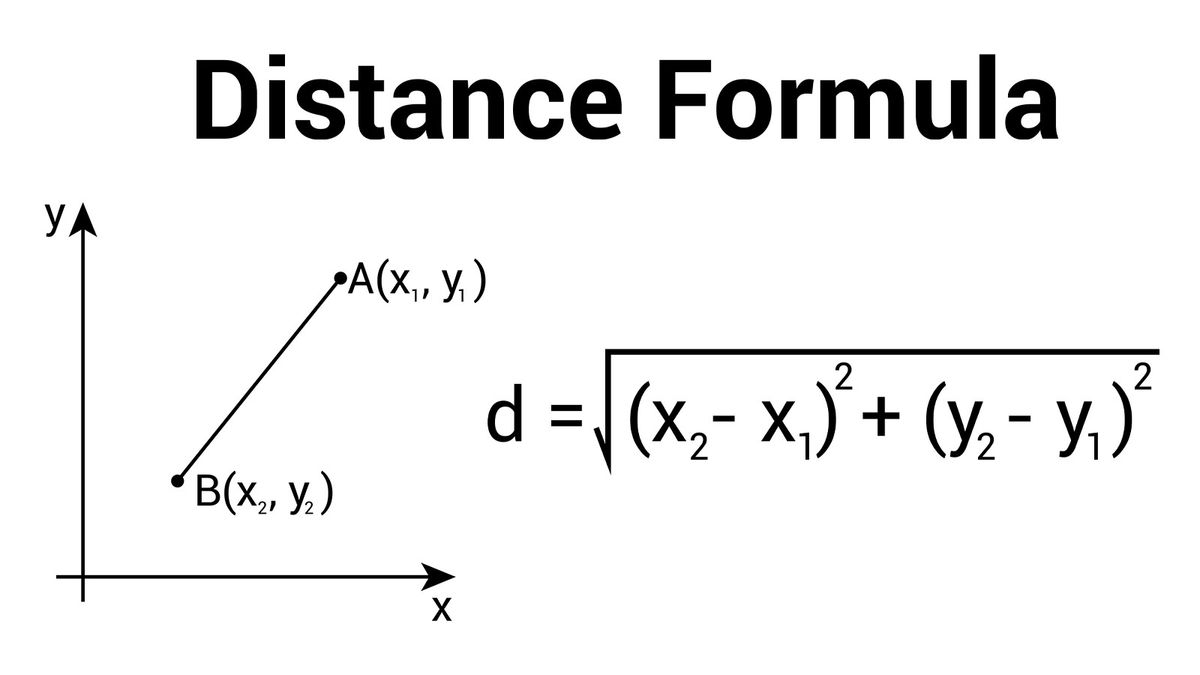
The distance formula is a method to calculate the length of a line segment connecting two points in a two-dimensional coordinate plane. Given two points ( A(x_1, y_1) ) and ( B(x_2, y_2) ), the distance ( d ) between them can be calculated as:
- ( d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} )
This formula is derived from the Pythagorean theorem, which states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. Here’s how it’s applied:
- Calculate the horizontal difference ( \Delta x = x_2 - x_1 ).
- Calculate the vertical difference ( \Delta y = y_2 - y_1 ).
- Apply these to the distance formula to find the straight-line distance between the points.
The Midpoint Formula

The midpoint formula provides the coordinates of the point that lies exactly halfway between two points. For points ( A(x_1, y_1) ) and ( B(x_2, y_2) ), the midpoint ( M ) is found using:
- ( M = \left( \frac{x_1 + x_2}{2}, \frac{y_1 + y_2}{2} \right) )
This formula essentially averages the x-coordinates and the y-coordinates:
- Add the x-coordinates and divide by two for the x-coordinate of the midpoint.
- Add the y-coordinates and divide by two for the y-coordinate of the midpoint.
Applications and Examples

Let’s look at some practical applications:
- Mapping and Navigation: By knowing the coordinates of starting and ending points, one can use the distance formula to determine the direct path or the midpoint to identify halfway points for meet-ups or stops.
- Engineering Design: Engineers often use these formulas to check measurements and ensure symmetrical placement of parts in design software or blueprints.
- Computer Graphics: In computer science, particularly in graphics and game programming, these formulas are crucial for calculating distances between objects, collision detection, and positioning elements on a canvas or screen.
Detailed Problem Solving

Let’s work through some examples:
Example 1: Find the distance between the points (1, 2) and (4, 6).
- Calculate ( \Delta x = 4 - 1 = 3 ).
- Calculate ( \Delta y = 6 - 2 = 4 ).
- Apply the formula: ( d = \sqrt{(3)^2 + (4)^2} = \sqrt{9 + 16} = \sqrt{25} = 5 ).
Example 2: Determine the midpoint of the line segment with endpoints (-2, 3) and (4, 9).
- Calculate ( \frac{(-2 + 4)}{2} = \frac{2}{2} = 1 ).
- Calculate ( \frac{(3 + 9)}{2} = \frac{12}{2} = 6 ).
- Thus, the midpoint is ( (1, 6) ).
Notes on Precision and Rounding
📝 Note: When performing calculations with coordinates, precision matters. Use exact values where possible, and round only at the final step to maintain accuracy.
These formulas are not just theoretical tools; they have direct implications in many fields. Understanding how to apply them accurately can streamline problem-solving processes, making complex tasks more manageable.
FAQ Section

What if the Points Are in Three-Dimensional Space?

+
In three-dimensional space, the distance formula includes a z-coordinate, becoming ( d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2 + (z_2 - z_1)^2} ). The midpoint formula extends similarly: ( M = \left( \frac{x_1 + x_2}{2}, \frac{y_1 + y_2}{2}, \frac{z_1 + z_2}{2} \right) ).
Can these formulas be used in curved space?

+
These formulas are derived for Euclidean geometry, which deals with flat surfaces. In curved or non-Euclidean space, other methods like differential geometry or approximations are used.
How does understanding these formulas benefit daily life?

+
Understanding these formulas can help in navigation, mapping routes, designing structures, and even in video games for determining distances and collisions. They simplify spatial calculations, making everyday problems involving measurements and directions easier to solve.