Geometry Basics: 5 Answers to Angle Addition Postulates

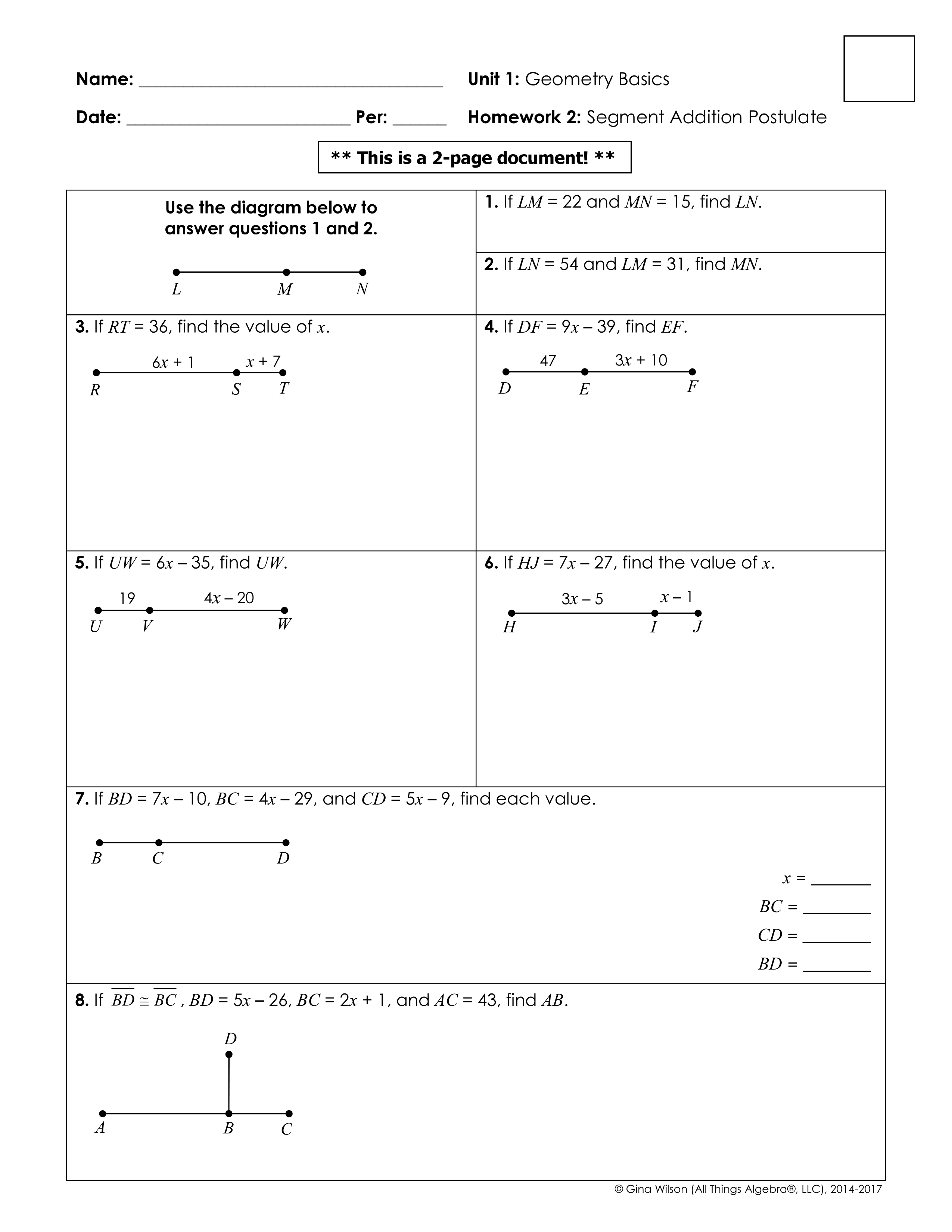
The angle addition postulate is a fundamental concept in geometry that allows us to understand how angles interact and combine within a geometric figure. By understanding this postulate, one can easily add or subtract angles in various shapes and scenarios, providing a robust foundation for more advanced geometrical concepts and proofs. This post delves into five common questions and answers related to the angle addition postulate, offering insights that will enhance your grasp on how angles work together.
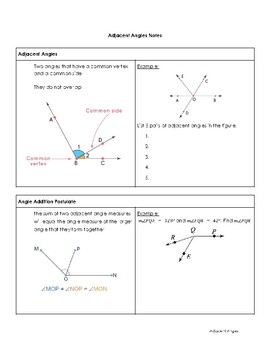
1. What is the Angle Addition Postulate?


The Angle Addition Postulate states that if a point lies on the interior of an angle, the sum of the two smaller angles formed by that point is equal to the measure of the original angle. Simply put, if you have an angle ABC and point D lies on line BC, then the measures of angles ABD and BDC add up to the measure of angle ABC. Here’s a basic example:
- Angle ABC = 90°
- Point D lies on BC, creating angles ABD = 30° and BDC = 60°
ABC = ABD + BDC
📌 Note: This postulate only works with angles that share a common side and have no interior points in common.
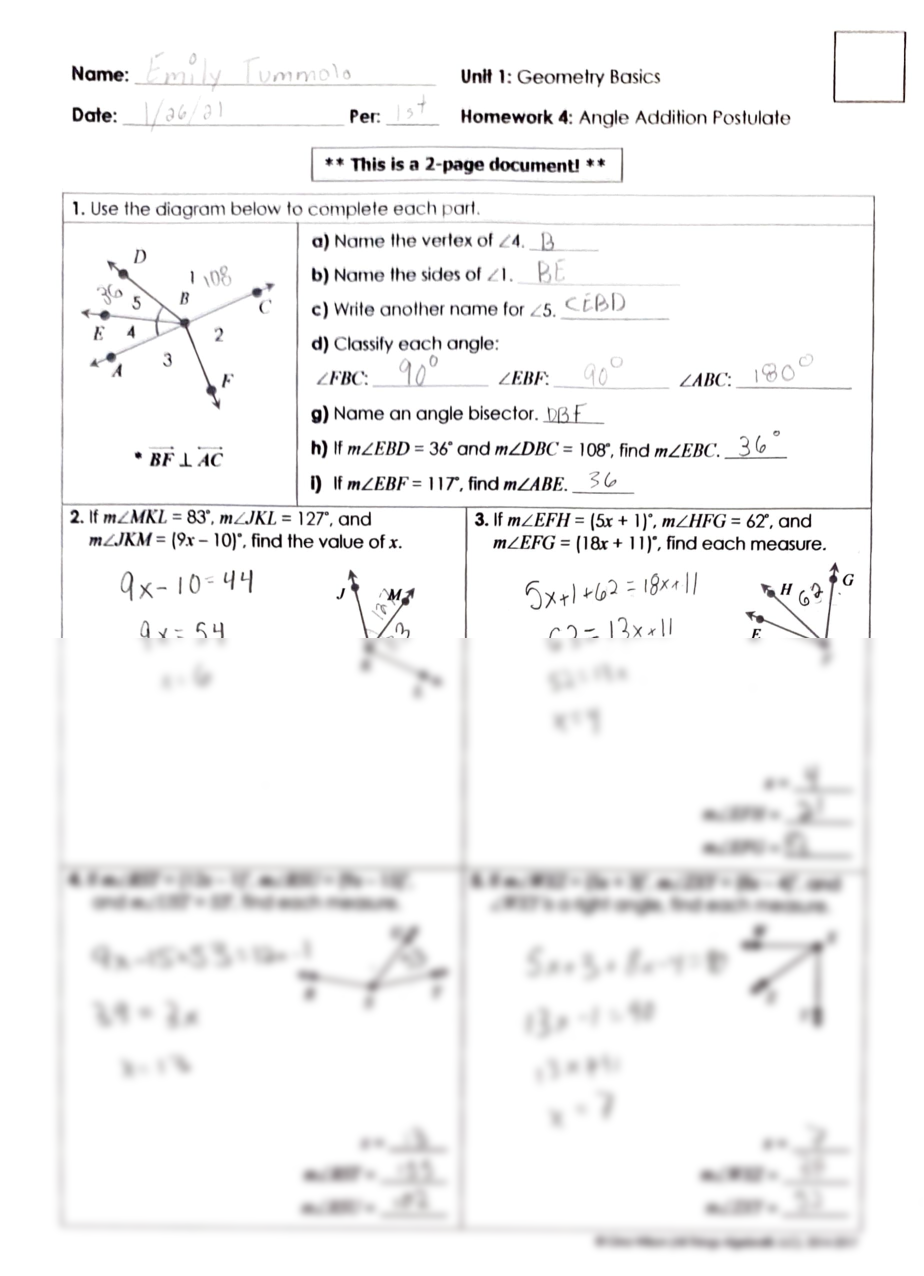
2. How is the Angle Addition Postulate Applied?
In practical terms, the Angle Addition Postulate is used in:
- Measuring Segments: When you have a line segment divided into multiple segments, and you know the angles formed at the division points.
- Solving Complex Problems: When dealing with triangles, polygons, or any shapes where angles need to be added or split into smaller parts.
- Geometric Proofs: It’s often used to prove theorems like the Triangle Sum Theorem.
Example:
If you're given that ∠AOB = 45°, ∠BOC = 20°, and point C lies on line AB, to find ∠AOC:
- ∠AOB + ∠BOC = ∠AOC
- 45° + 20° = 65°
Thus, ∠AOC = 65°
3. Common Misconceptions About the Postulate

Understanding common misconceptions can help clarify the true nature of the angle addition postulate:
- Misconception: It only applies to straight lines or right angles.
- Truth: It applies to any angle, regardless of the measure or shape of the line segments involved.
- Misconception: The postulate only works with external angles.
- Truth: It is also valid for internal angles that share a common side.
- Misconception: The angles must be adjacent.
- Truth: While typically demonstrated with adjacent angles, it can be used with any angles that share a side as long as the shared side does not overlap with other angles.
4. Why Does the Angle Addition Postulate Matter?
The Angle Addition Postulate is more than just a theoretical tool; it has practical applications:
- Design: Architects and engineers use it to calculate angles in structural components.
- Mathematics: It’s foundational in trigonometry and other areas where angle calculation is vital.
- Surveying: Surveyors use it to measure and map land accurately.
By providing a straightforward way to work with angles, this postulate allows for seamless integration into various mathematical problems and real-world scenarios.
5. Advanced Applications

As you delve deeper into geometry, the Angle Addition Postulate becomes even more critical:
- Non-Euclidean Geometry: Understanding how angles interact in non-Euclidean spaces.
- Dynamic Geometry: Using software to simulate and understand geometric transformations where angles change in real-time.
- Spatial Reasoning: It helps in developing spatial intelligence, crucial for fields like computer graphics and game development.
🌟 Note: For advanced applications, additional postulates and theorems become necessary to ensure precise calculations.
This in-depth exploration of the angle addition postulate illustrates not only its simplicity but also its depth. From basic addition of angles to complex problem-solving in various fields, this principle serves as a cornerstone for understanding how angles and shapes interact. Embracing this concept provides a clear pathway to mastering more intricate geometrical challenges and applications.
Can you have negative angles in the Angle Addition Postulate?

+
Technically, no. Angles are generally considered positive measures. However, in certain mathematical contexts or when considering rotation, a negative angle can indicate a clockwise direction, but it doesn’t change the postulate’s core principle of addition.
Does the Angle Addition Postulate apply to obtuse angles?
+
Absolutely. The postulate applies to any angles, regardless of their size. If an obtuse angle is divided, the sum of the smaller angles will equal the measure of the larger, original angle.
What happens if an angle is bisected by a point?

+
If an angle is bisected, it is divided into two equal angles. Using the Angle Addition Postulate, we can confirm that each smaller angle equals half of the original angle, making their sum equal to the original angle.