Geometry 1.2 Worksheet Answers: Solve Problems with Ease

In this geometry worksheet answer guide, we'll delve into problem-solving strategies to help you overcome challenges with shapes and spaces. Geometry, an integral part of mathematical education, equips students with the necessary skills to understand and calculate spatial relationships, angles, perimeter, area, and volume. Our focus will be on section 1.2 of your geometry curriculum, a stage where foundational concepts are applied in new ways, often causing confusion or errors if not approached correctly.
Understanding the Geometry 1.2 Worksheet

The geometry worksheet labeled as “1.2” generally introduces or revisits fundamental concepts like:
- Types of angles (acute, obtuse, right, straight)
- Parallel lines and transversals
- Properties of triangles and quadrilaterals
- Basic area and perimeter calculations
Here, students begin to apply the learned theories to solve practical problems, which is where this worksheet comes into play, offering a wide range of questions to test understanding.

Key Concepts for Geometry 1.2

Before diving into the solutions, let’s review the key concepts covered in this section:
Types of Angles

- Acute Angles: Angles less than 90°
- Obtuse Angles: Angles between 90° and 180°
- Right Angles: Exactly 90°
- Straight Angles: Exactly 180°
Parallel Lines and Transversals

When parallel lines are cut by a transversal, the following pairs of angles are formed:
- Corresponding Angles
- Alternate Interior Angles
- Alternate Exterior Angles
- Consecutive Interior Angles
These relationships allow for the calculation of unknown angles in diagrams with parallel lines.
Properties of Polygons

Here’s a quick reminder of polygon properties:
| Shape | Sum of Interior Angles | Formula for Area |
|---|---|---|
| Triangle | 180° | ½ × base × height |
| Quadrilateral | 360° | Varies based on type |

🔍 Note: Ensure to check the given or calculated angles and not to confuse the interior angles with the exterior ones.
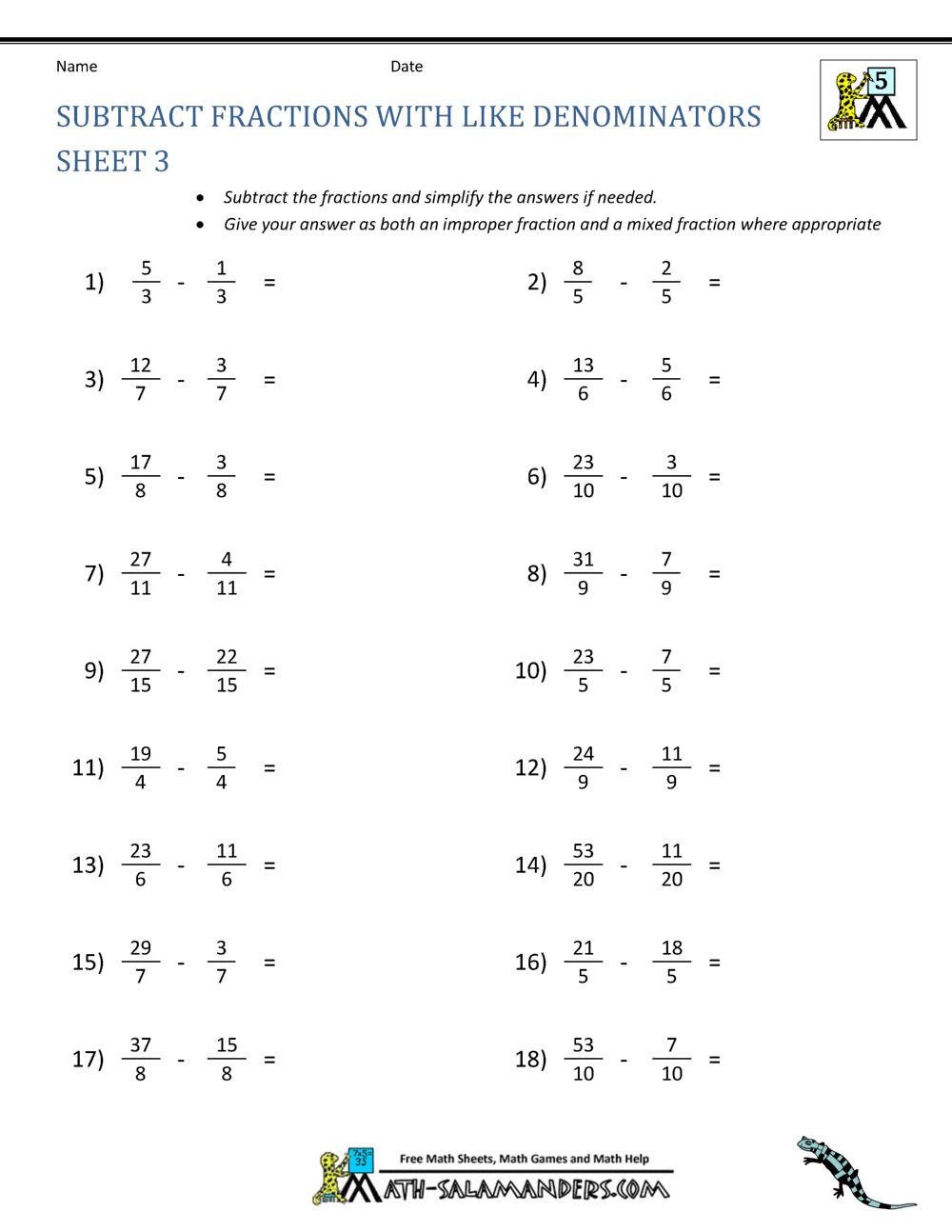
Solving Perimeter and Area
For calculating perimeter, sum up the length of all sides. For area, you’ll need to know the shape’s dimensions:
- Triangle: Use the formula for base and height or Heron’s formula if side lengths are given.
- Rectangle: Multiply length by width.
- Parallelogram: Base × height.
Geometry 1.2 Worksheet Answers

The following are step-by-step solutions for problems commonly encountered in the Geometry 1.2 worksheet:
Problem 1: Identify Angle Types

In this problem, you might be given angles and asked to identify their types. Here’s how:
- If an angle measures 45°, it’s acute.
- If an angle measures 120°, it’s obtuse.
- If the angle appears to be a perfect L shape, it’s right (90°).
Problem 2: Parallel Lines and Transversals

Given a diagram with parallel lines cut by a transversal, determine unknown angles:
- Identify pairs of angles and use their known relationships (e.g., alternate interior angles are congruent).
- If angle α = 40°, then all corresponding angles equal 40°.
- Subtract or add angles to find supplementary or complementary angles.
Problem 3: Triangle Angles

To find the third angle of a triangle when two are given:
- Sum of angles in a triangle = 180°.
- If angles are 45° and 75°, third angle = 180° - (45° + 75°) = 60°.

Problem 4: Quadrilateral Properties

Calculate missing angles in a quadrilateral:
- Sum of angles = 360°
- If three angles are known, the fourth is (360° - sum of known angles).
Recap

We’ve covered key geometric concepts from Section 1.2 and provided answers to common problems. Understanding the relationships between angles, lines, and polygons forms the foundation for more complex geometry. Always remember to:
- Identify the given information.
- Apply known properties and relationships.
- Solve methodically.
How do I identify if two lines are parallel?

+
To identify if two lines are parallel, look for:
- Equal alternate interior or exterior angles when a transversal cuts the lines.
- Corresponding angles being equal.
- Supplementary consecutive interior angles.
What’s the difference between perimeter and area?

+
Perimeter is the total length of the outline of a 2D shape. Area is the amount of 2D space the shape occupies, calculated using different formulas based on the shape.
How do I solve problems where triangles overlap?
+When triangles overlap:
- Identify and label shared segments or angles.
- Use properties of triangles (e.g., sum of angles) for each individual triangle.
- Combine or subtract areas if necessary to find the desired result.
What should I do if I’m stuck on a geometry problem?
+If you’re stuck on a geometry problem:
- Re-read the problem to ensure all details are clear.
- Break it down into smaller steps.
- Use diagrams or sketches to visualize the problem.
- Review the relevant geometric rules or theorems.
- Practice similar problems for better understanding.