Function Notation Worksheet: Boost Your Math Skills Now!

Are you looking to sharpen your mathematical prowess, particularly in function notation? Then this comprehensive worksheet guide is just what you need to elevate your skills and grasp those fundamental math concepts that underpin algebra, calculus, and other advanced topics. From understanding the syntax to solving complex problems, this post will walk you through everything you need to master function notation.
What is Function Notation?
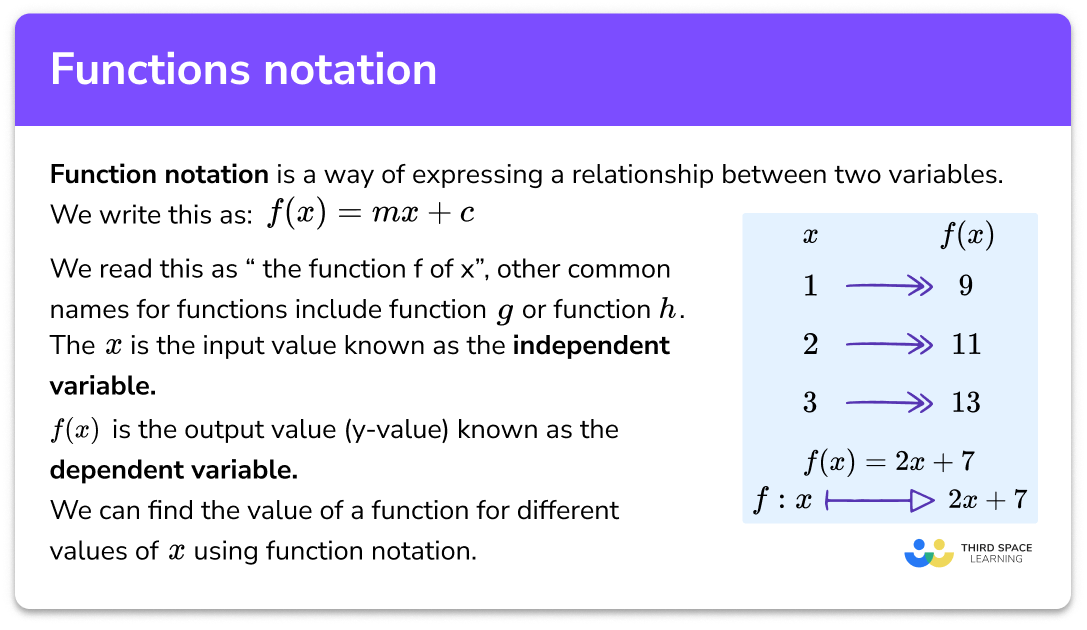
Function notation is a method of representing functions algebraically. It’s a shorthand that provides a clear, concise way to express the relationship between variables. Instead of writing long equations, you can denote a function by f(x) or any other letter of the alphabet, where f is the function name and x is the variable.
Basic Function Notation Rules

- Function Naming: Functions are often named f(x), g(x), or h(x), but can use any letter or symbol.
- Variable Representation: The variable inside parentheses (x in f(x)) represents the input.
- Output: What comes out of the function, often symbolized as y, f(x), or other forms.
- Operations: You can perform operations on the function, like addition, subtraction, multiplication, and division.
Examples of Function Notation

Let’s look at some basic examples to illustrate function notation:
- The function f(x) = 2x + 3 shows that for any input x, the function multiplies it by 2 and then adds 3.
- If you want to find f(2), plug in 2 for x: f(2) = 2(2) + 3 = 7.
Table of Common Functions

| Function | Notation | Description |
|---|---|---|
| Linear | f(x) = mx + b | Slope-intercept form where m is the slope and b is the y-intercept. |
| Quadratic | f(x) = ax2 + bx + c | Describes a parabola where a, b, and c define its shape and position. |
| Exponential | f(x) = a * bx | Represents exponential growth or decay. |

Practical Applications

Function notation isn’t just for theoretical mathematics; it’s prevalent in real-world applications:
- Economics: You can use it to model supply and demand curves.
- Physics: It helps describe relationships like velocity over time or the motion of objects.
- Computer Science: Functions are fundamental to algorithms and data structure analysis.
- Engineering: From calculating force to designing control systems, functions are key.
How to Solve Function Notation Problems

Here’s a step-by-step guide to solving problems involving function notation:
- Understand the Function: Identify what the function represents and its components.
- Substitute the Variable: Replace the input variable with the given value.
- Simplify the Equation: Solve the resulting equation for the output.
- Check your Work: Ensure your final answer makes sense with the given context.
⚠️ Note: Pay attention to the function’s domain. Certain values might not be valid for the input, leading to undefined outputs.
Challenges You Might Face

Here are some common pitfalls and how to overcome them:
- Misinterpreting the Notation: Ensure you understand what f(x) means versus what f itself is.
- Ignoring Domain Restrictions: Always check for restrictions on the function’s domain.
- Order of Operations: Adhere to the correct order of operations when simplifying expressions.
- Confusing Functions with Equations: Remember, functions imply a unique output for each input; equations can have multiple solutions.
Wrapping it Up

Mastering function notation not only enhances your understanding of mathematics but also opens doors to various fields where math models real-world phenomena. Remember to start with the basics, gradually build your skills, and practice consistently. Soon, you’ll find function notation to be as second nature as basic arithmetic, allowing you to tackle more complex mathematical problems with confidence.
Why is function notation important?

+
Function notation allows for efficient and clear representation of mathematical relationships, making it easier to work with complex functions and apply them in various contexts.
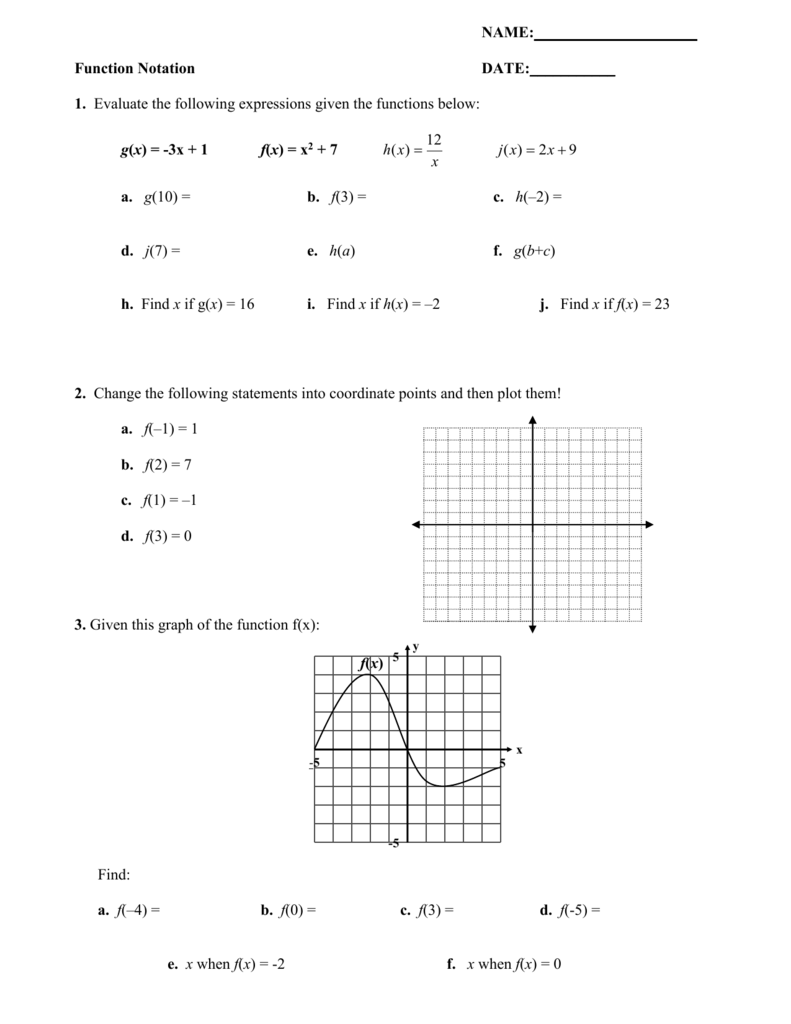
How do you solve a function for a specific value?
+
Substitute the given value for the variable in the function, simplify the expression, and interpret the result according to the context or domain restrictions.
Can a function have more than one variable?

+
Yes, functions can have multiple variables. These are called multivariate functions, and they often represent more complex relationships.