Free Body Diagram Mastery: Essential Worksheet Practice

In the study of physics, understanding forces and how they interact with objects is crucial. One of the most effective tools for visualizing these interactions is through Free Body Diagrams (FBDs). Whether you're just starting out or brushing up on your skills, mastering the art of creating FBDs can significantly enhance your understanding of physics, particularly in mechanics. This blog post will guide you through the process of creating FBDs with practical examples and step-by-step instructions.
Why Free Body Diagrams are Important

Free Body Diagrams are not just a part of academic physics; they are vital in engineering, architecture, and many other fields where forces play a role. Here’s why:
- Visual Representation: FBDs provide a clear visual representation of all forces acting on an object, simplifying complex problems.
- Problem Solving: They help in breaking down forces into components, making calculations easier.
- Conceptual Clarity: By isolating an object, FBDs help students understand how forces influence motion and equilibrium.
How to Create a Free Body Diagram

Creating a Free Body Diagram involves several steps:
- Identify the System: Determine which object or system you will analyze. Draw a simple sketch of this system.
- Isolate the Object: Imagine your object is in isolation, free from contact with anything else.
- List All Forces: Identify and list all forces acting on the object:
- Weight (Gravity) - Directed downwards.
- Normal Force - Perpendicular to the surface in contact.
- Frictional Force - Parallel to the surface in contact.
- Applied Forces - Pushes or pulls exerted by external agents.
- Tension - Forces due to a string or rope.
- Draw the Diagram: On your isolated object:
- Draw the object as a point or a simplified shape.
- Represent each force with an arrow pointing in the direction of the force. Label each arrow with its magnitude if known.
- Resolve Forces: If necessary, break forces into horizontal and vertical components.
🔍 Note: For a more complex object like a car on a ramp, consider breaking forces into their components parallel and perpendicular to the ramp's surface.
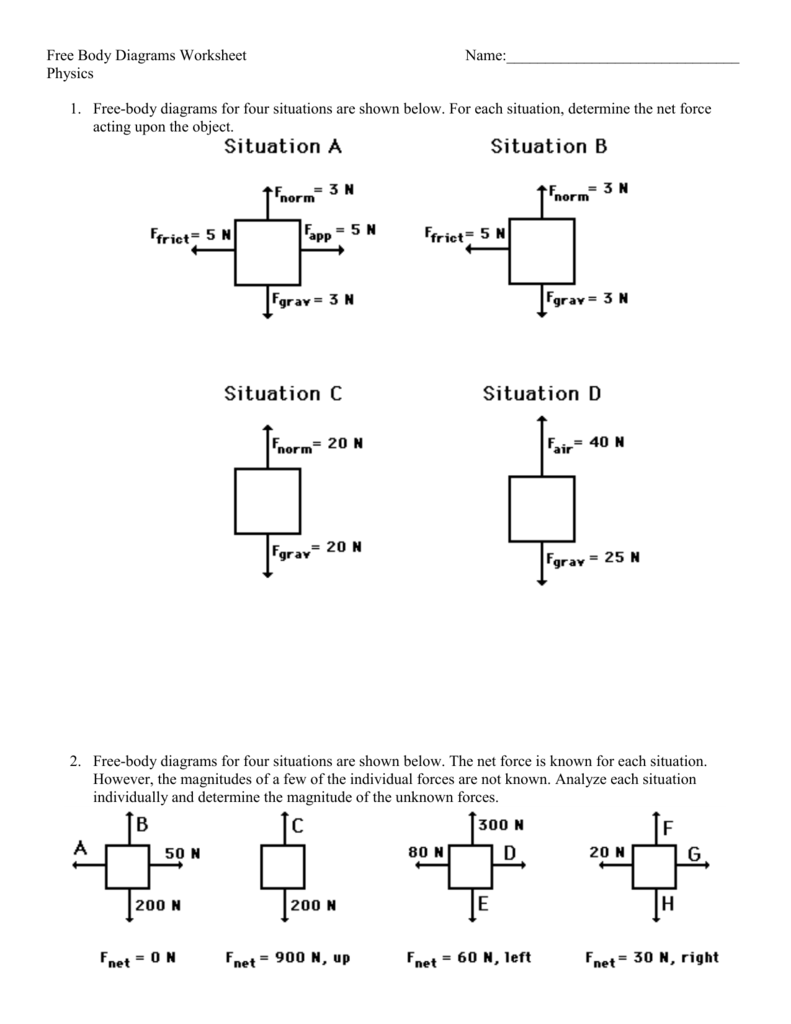
Worksheet Practice

To solidify your understanding, let’s work through some examples:
Example 1: Box on a Flat Surface

- Draw a box on a flat surface.
- Identify forces: Weight (acting downward) and Normal force (acting upward).
| Force | Direction |
|---|---|
| Weight (W) | Downward |
| Normal Force (N) | Upward |

Example 2: Object Hanging from a Rope

- Draw the object as a point, with a rope attached.
- Identify forces: Weight (downward) and Tension (upward).
| Force | Direction |
|---|---|
| Weight (W) | Downward |
| Tension (T) | Upward |
Example 3: Book on an Inclined Plane

- Draw the book on the incline.
- Identify forces: Weight (downward), Normal force (perpendicular to the incline), Frictional force (parallel to the incline), and possibly an applied force if the book is moving.
🔬 Note: When dealing with an inclined plane, remember to break forces into components parallel and perpendicular to the plane.
By practicing these examples, you will become more adept at recognizing and drawing FBDs accurately. Here are some additional tips:
- Always use arrows to represent forces, ensuring the length of the arrow reflects the magnitude when known.
- Label each force clearly.
- Check for equilibrium conditions (sum of forces = 0) if the object isn't accelerating.
With regular practice, Free Body Diagrams will become an indispensable tool in your problem-solving arsenal, enabling you to analyze and understand complex systems with ease.
What if I can’t identify all forces acting on an object?

+
If you are unsure of all the forces acting on an object, start with the most obvious ones like gravity and normal force. Remember, friction or tension might only be present under certain conditions (like contact with a surface or the presence of a string).
How do I handle forces not along the principal axes?

+
Break down these forces into their components along the x and y axes or along the inclined plane. This makes it easier to apply Newton’s laws of motion.
Can Free Body Diagrams help in dynamic problems as well as static ones?
+
Absolutely. FBDs are just as useful in dynamic scenarios where acceleration is present. Here, you would apply Newton’s second law (F = ma) to relate forces to the motion of the object.
With these insights and practices in your toolkit, you’re well on your way to mastering Free Body Diagrams, opening up a clearer path to understand forces, equilibrium, and motion in the fascinating world of physics.


