5 Tips for Solving Fractions with Unlike Denominators

The world of fractions can seem daunting when it comes to solving problems involving fractions with unlike denominators. However, mastering this concept not only deepens your understanding of arithmetic but also streamlines your ability to manage everyday mathematics, from cooking recipes to financial calculations. Let's dive into five essential tips that will make solving fractions with unlike denominators a breeze.
Understanding the Concept

Before we delve into the tips, it’s crucial to understand what unlike denominators mean. In simple terms, unlike denominators refer to two or more fractions where the denominators are not the same. Here’s a quick refresher:
- A fraction comprises a numerator and a denominator.
- The numerator represents the number of parts considered.
- The denominator indicates the total number of parts the whole is divided into.
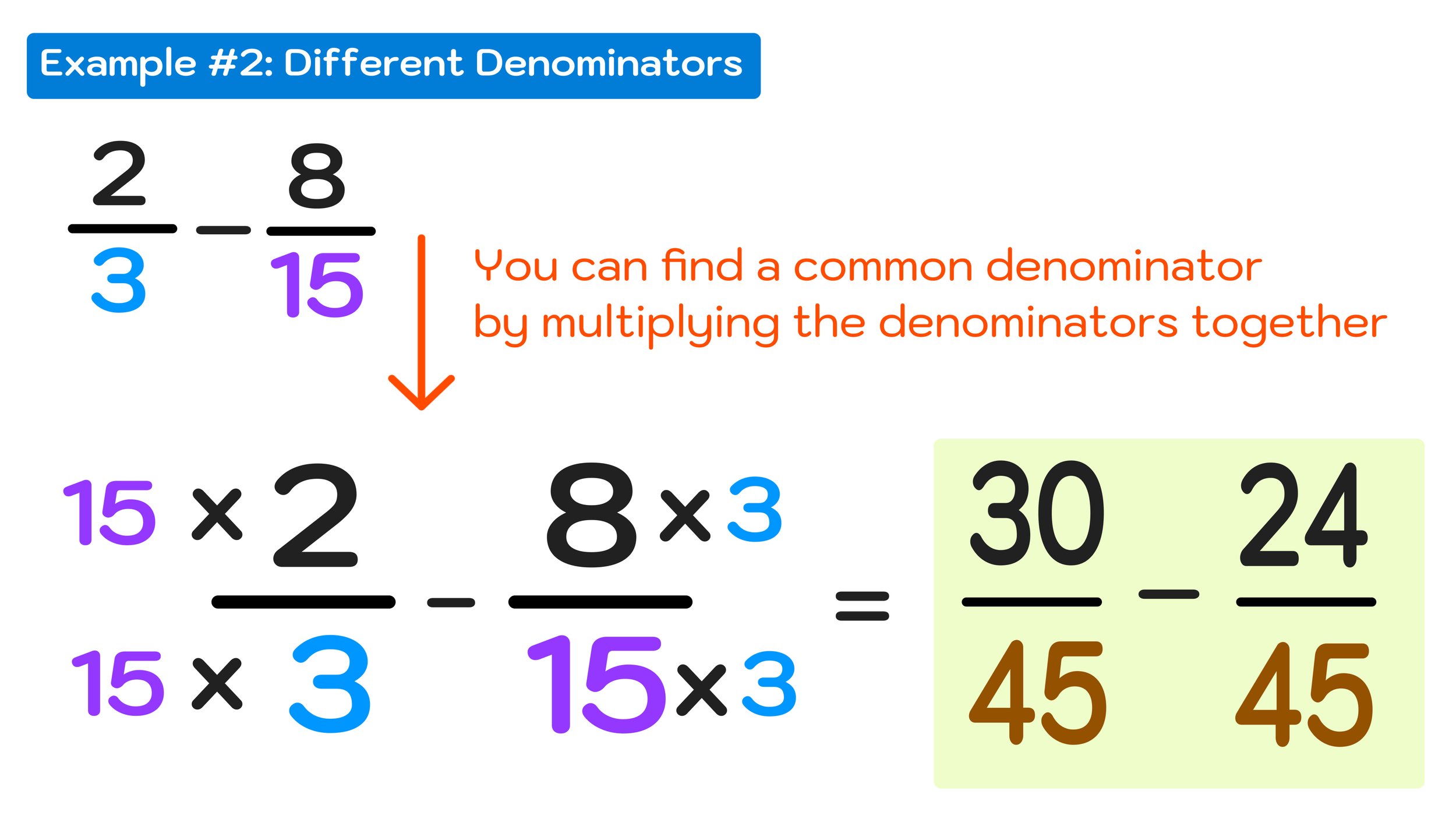
Tip 1: Find the Least Common Denominator (LCD)

The first step in solving fractions with unlike denominators is finding a common ground—literally. The Least Common Denominator (LCD) is the smallest number that all denominators in the problem can evenly divide into. Here’s how to find it:
- List out the multiples of each denominator until you find a common number.
- If the numbers are large, prime factorization can be useful to find the LCD more efficiently.
🔍 Note: You can also use the Least Common Multiple (LCM) method, but the LCD will be the same in many cases.
Tip 2: Convert Fractions to Equivalent Fractions with the LCD

Once you’ve found the LCD, you’ll need to convert each fraction into an equivalent fraction with the LCD as the denominator. Here’s the process:
- Divide the LCD by the original denominator of each fraction.
- Multiply both the numerator and the denominator by the result from step 1.
This transformation ensures that all fractions have the same denominator, making them easier to work with.
Tip 3: Perform the Desired Operation

With all fractions now sharing the same denominator, you can proceed with:
- Adding or subtracting by adding or subtracting the numerators.
- Multiplying or dividing by multiplying or dividing the fractions directly.
🔎 Note: Remember, when adding or subtracting, keep the denominator the same after the operation.
Tip 4: Simplify Your Answer
After performing the operation, you might end up with a fraction that can be simplified. Here are the steps to simplify:
- Find the Greatest Common Divisor (GCD) of the numerator and the denominator.
- Divide both the numerator and the denominator by the GCD.
Simplifying the fraction makes your final answer more manageable and often reveals patterns or relationships between numbers.
Tip 5: Practice, Practice, Practice

Like any skill, proficiency in solving fractions with unlike denominators comes from practice. Here are some ways to sharpen your skills:
- Create flashcards with fraction problems.
- Use online tools or apps designed for fraction arithmetic practice.
- Challenge yourself with increasingly complex problems over time.
In conclusion, while working with fractions with unlike denominators might initially appear complex, it's a skill that becomes second nature with practice and understanding. By following these five tips—finding the Least Common Denominator, converting to equivalent fractions, performing operations, simplifying your answers, and consistent practice—you'll navigate through fraction problems with ease and confidence. These strategies not only enhance your mathematical prowess but also empower you to tackle real-world applications of fractions.
Why is it important to find the Least Common Denominator?

+
Finding the LCD is crucial because it provides a common ground for all fractions involved, allowing for straightforward arithmetic operations by converting fractions into equivalent forms.
Can I always simplify my answer after operations on fractions?

+
Not always, but it’s a good practice. If the fraction can be simplified, doing so makes the answer more readable and often reveals underlying numerical relationships.
Is there an easier way to find the LCD for large numbers?

+
Yes, prime factorization can be more efficient for larger denominators. List the prime factors of each number, then multiply the highest powers of all prime factors present to find the LCD.