5 Simple Ways to Teach Fractions on a Number Line

Teaching fractions on a number line can transform what seems like a daunting concept into an understandable and engaging subject for students. By visualizing fractions through this method, learners can grasp the relationship between whole numbers, parts of a whole, and where fractions fit into the larger mathematical universe. Here are five effective and simple ways to teach fractions using a number line:
1. Introduce Basic Fractions
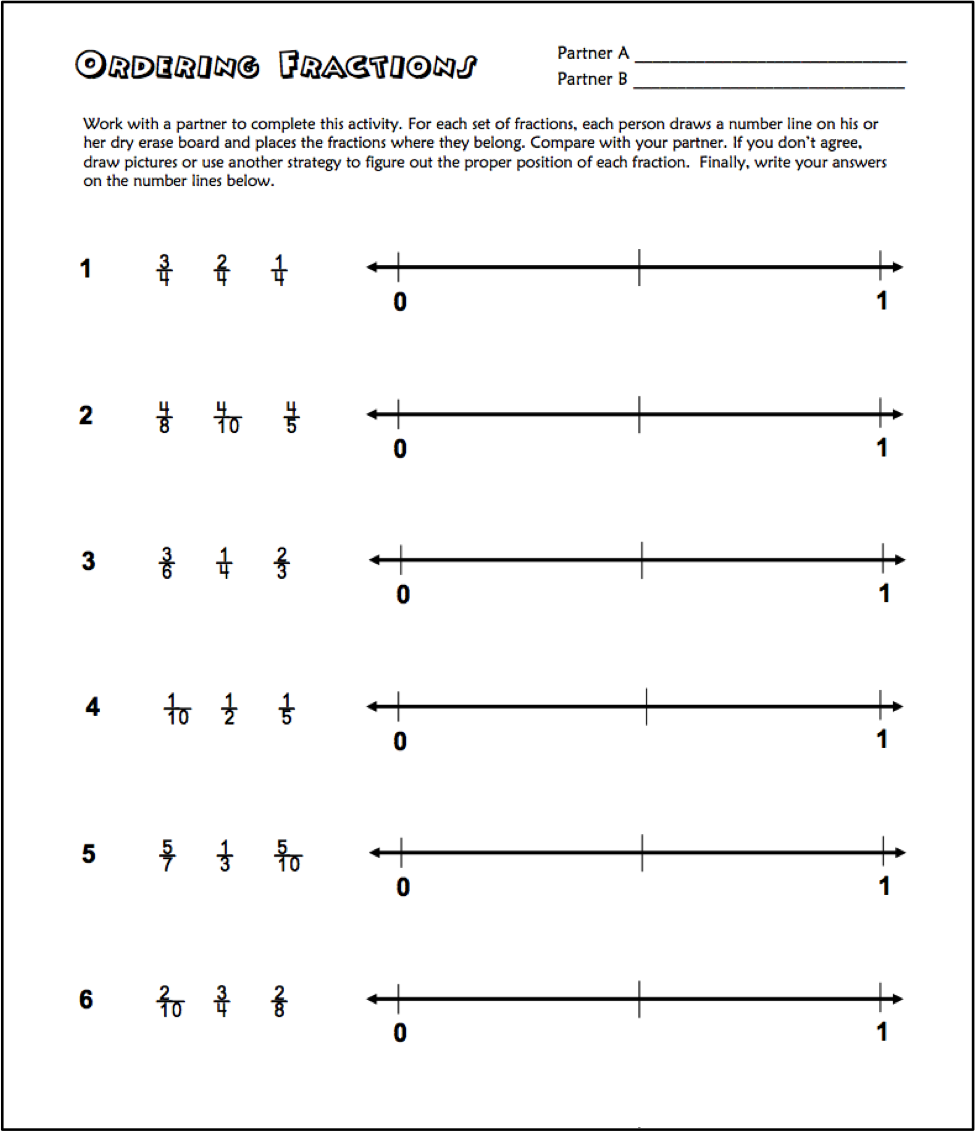
Start with the basics. Show students a number line where 0 and 1 are marked. Divide the line segment between these points into equal parts. For instance, if you're demonstrating the fraction 1/2:
- Draw a line from 0 to 1.
- Mark the halfway point at 0.5, labeling it as "1/2."
This visual representation helps students understand that fractions are not abstract but tangible divisions of a whole.

🌟 Note: For clarity, label the whole number as well as the fraction to show where the fraction fits in the number system.
2. Use Concrete Objects to Build Understanding

Enhance the learning experience by incorporating physical objects. For example:
- Have students use candy, small toys, or even blocks to divide segments on a physical or drawn number line.
- For 1/4, students can count out four candies and place them evenly along the line from 0 to 1, highlighting each quarter mark.
This method not only makes learning fun but also helps students connect the concept of fractions to real-life scenarios.

🎨 Note: Be sure to use colorful and varied objects to keep students engaged and facilitate visual differentiation of different fractions.
3. Interactive Games and Activities

Games can make the learning of fractions on a number line more enjoyable. Here are a couple of ideas:
- Number Line Hop: Create a giant number line on the floor or use tape to mark off segments. Students can 'hop' to the correct fraction when called out.
- Fraction Card Match: Make cards with fractions and corresponding points on a number line. Students match the fraction card to the correct point on a number line.
These activities encourage active participation and deepen the understanding of where fractions lie relative to one another.

4. Progress to Improper Fractions and Mixed Numbers

Once students are comfortable with basic fractions, introduce them to improper fractions and mixed numbers:
| Step | Description |
|---|---|
| Step 1 | Extend the number line beyond 1. |
| Step 2 | Label the line with numbers up to at least 2 or 3. |
| Step 3 | Show how fractions like 5/4 fit into the number line as 1 1/4. |

This step-by-step process visually demonstrates that fractions can extend beyond whole numbers.

5. Comparisons and Equivalents

Teach students to compare and find equivalent fractions using the number line:
- Choose two fractions, place them on the number line, and ask students which one is larger or if they are equal.
- Demonstrate equivalence by showing that 2/4 is the same as 1/2 because they occupy the same spot on the line.
This practice helps students see the practical application of fractions in ordering and equivalence, which is crucial for algebraic thinking.

Recapping these strategies, we've explored various engaging and visual methods to introduce and reinforce the concept of fractions using a number line. From basic division to playing interactive games, using concrete objects, understanding mixed numbers, and finally, comparing fractions, these techniques cater to different learning styles and make fractions accessible. By making learning interactive and concrete, students not only comprehend but also appreciate the beauty and utility of fractions in mathematics. Through these simple yet effective ways, teachers can help students demystify fractions, turning a potentially abstract idea into something that's grounded in visual understanding and real-world applications. Engaging with fractions in these tangible ways ensures that the fundamental building blocks of numeracy are well-understood and retained.
Why is it important to teach fractions on a number line?
+
Visualizing fractions on a number line helps students understand the proportional relationships between fractions and whole numbers, making abstract concepts concrete and accessible.
Can these methods be adapted for older students or advanced learners?

+
Yes, the strategies can be scaled up for complexity, introducing algebraic concepts, negative fractions, and operations with fractions on the number line for more advanced learning.
How do you keep students engaged during fraction lessons?

+
By incorporating games, physical activities, and relatable real-world examples, you can maintain student interest and encourage active participation in learning fractions.